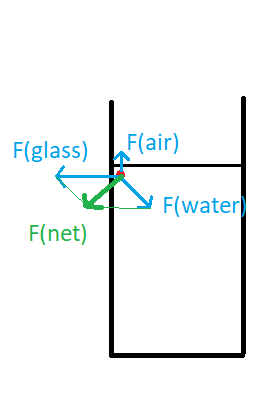
I've learnt that capillary action is caused by forces acting on molecules near the interface between solids, liquids (and gasses as well). But there are few things I don't understand.
Imagine we have water in a glass container as shown in the picture. Why would molecules near the container rise if the net force acting on them have no more "upwards vector" than let's say molecules in the middle? I can see that the vector of the net force is pointing more towards the container than vectors of the net forces of molecules in the middle, but it is not pushing it upwards!...
My second questions is, why does the surface level in a small tube rises the higher the smaller the diameter of the tube is (due to capillary action)? I think it is because smaller diameter means that a higher percentage of the molecules are being affected by the upward forces and the net force $F = F_{gravity}-F_{capillary}$ has "more up-direction".