The electron is a quantum mechanical entitity, i.e. it obeys solutions of quantum mechanical equations.
The quantum mechanical problem to be solved is : electron of momentum p impinges on two slits of width $w$ each, a $d$ distance apart. What is the probability distribution of seeing the electron on a screen after it has left the slits?
The probability will be given by the $Ψ^{*}Ψ$ of the solution of the above quantum mechanical problem.
Here is the experiment:
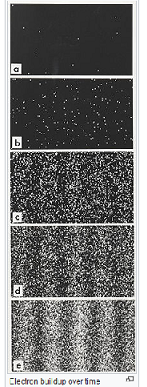
So truly the wave function justifies its name by showing a probability distribution with interference patterns expected from the solutions of a wave equations.
Why don't the slits themselves cause the wave function to collapse?
Because the fringe electrical fields of the slits are part of the boundary conditions which will define the wavefunction of the particular experiment.
Conversely if the slit is acting in the "wave domain", leaving the wave nature of the particle unchanged
The wave nature is not an attribute of the particle, the way the spin, the mass and the charge are. The wave nature is part of the particular solution of the quantum mechanical equation with its set up and boundary conditions, which will allow to see it or not.
A single electron leaves a bubble chamber track defining it as a particle. Its wave nature appears in the probability of hitting a hydrogen atom and ionizing it, there is a probability distribution for this.

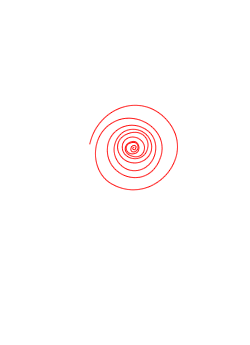
The curly line was produced by an electron that was struck by one of twelve passing beam particles in a liquid hydrogen bubble chamber. It curves in an applied magnetic field and loses energy rapidly, spiraling inwards.
The curly line is from the tail of the interaction of the charged Kaon beam traversing the chamber, a high momentum electron kicked off. All the dots are small energy electrons kicked off the hydrogen atoms of the chamber. It is all about probabilities, and the wave nature becomes evident only in the special experiments where interference in the accumulated distribution of individual scatters can be seen, as above with the single electron double slit.
This is an interesting experiment, showing how changing the boundary conditions affects the interference patterns.
So the answer to the title question :
In the double-slit experiment, why don't slits destroy interference pattern?
is that:
the slits are part of the boundary conditions that generate the specific wavefunction which gives to the probability distribution the interference patterns.



