Studying the QCD running coupling constant I ran into this figure:
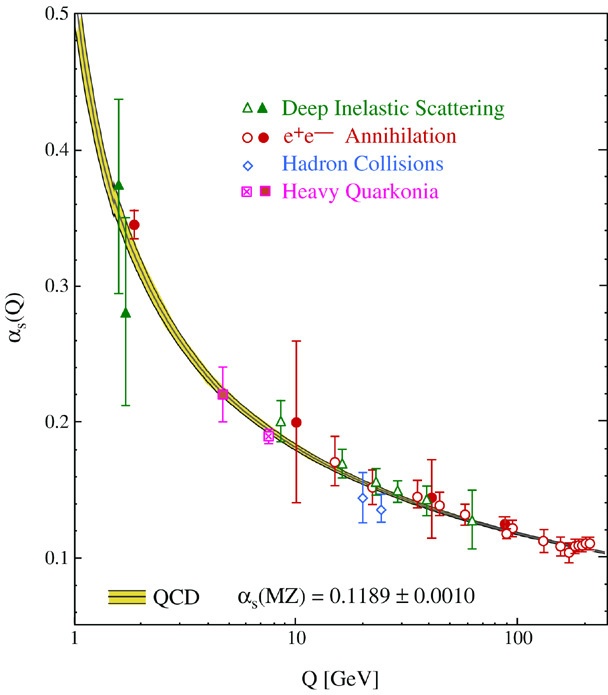
where Q$Q$ on the x$x$ axis represents the transferred momentum. I know from a Nuclear and Subnuclear Physics course that the strong interaction coupling constant is very small at small distances, so I was wondering why "high momentum transfer" equals "small distance" in this context.? I found similar questions in some physics forum and the answer was "for the Heisenberg Uncertainty Principle". I studied the Heisenberg Principle as \begin{equation} \Delta p\Delta x\ge\frac{\hbar}{2} \end{equation} so, to get a correspondence between "high momentum transfer" and "small distance" the equality should hold. If the inequality holds I could have "high momentum transfer" and "great distances" without violating the principle. Can someone explain to me why $\Delta p\Delta x\sim\hbar/2$$$\Delta p\Delta x\sim\hbar/2$$ seems to hold (instead of the version with $\ge$)?
