Despite the many (and some highly-upvoted) answers to the contrary, I believe the answer is actually that they can't, because if they did they would violate Conservation of Etendue and by extension the 2nd Law of Thermodynamics.
From the Wikipedia:
The etendue of a given bundle of light is conserved: etendue can be increased, but not decreased in any optical system. This means that any system that concentrates light from some source onto a smaller area must always increase the solid angle of incidence (that is, the area of the sky that the source subtends). For example, a magnifying glass can increase the intensity of sunlight onto a small spot, but does so because, viewed from the spot that the light is concentrated onto, the apparent size of the sun is increased proportional to the concentration.
As shown below, etendue is conserved as light travels through free space and at refractions or reflections. It is then also conserved as light travels through optical systems where it undergoes perfect reflections or refractions. However, if light was to hit, say, a diffuser, its solid angle would increase, increasing the etendue. Etendue can then remain constant or it can increase as light propagates through an optic, but it cannot decrease. This is a direct result of the fact that entropy must be constant or increasing.
Conservation of etendue can be derived in different contexts, such as from optical first principles, from Hamiltonian optics or from the second law of thermodynamics.
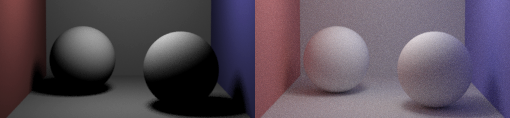
The floor acts as a diffuser in this case (or maybe more accurately, we can see it due to diffuse reflections which I understand to mean that it increases the etendue).
Also see Can you use a magnifying glass and moonlight to light a fire? and the related follow-up physics SE question.
To summarize my understanding of it, basically you cannot take light from a light source and focus it to make some other spot brighter than the light source. i.e. the sun is ~6000K so you can magnify the sun to burn paper, but the moon is only ~400K and you can't magnify this to burn paper. You can easily verify this by looking at a wall through a magnifying glass -- even though you can get more of the wall to focus onto your pupil, the brightness of the wall remains constant.
Basically if we take the light from the floor and redirect it onto a completely unlit surface, that surface could only become as bright as the floor, no matter how many reflections or magnifications are done etc.
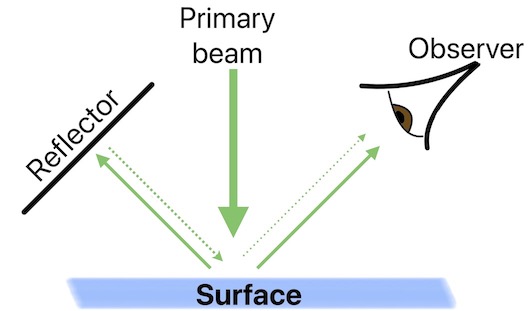
But that's onto an unlit surface -- couldn't we reflect the light back onto itself to make a spot brighter?
Proof by contradiction: let's say we could. The light from the floor goes into some optic system that redirects the light back to the floor and makes some portion of it brighter. Now we could introduce another optic system that magnifies this brighter spot (e.g. like magnifying the sun (that's small relative to the sky) with a magnifying glass) and projects it onto an unlit surface, to make that surface brighter than the floor initially was. This violates conservation of etendue, therefore it's impossible.
From a thermodynamic point of view, it would mean we could focus an object's thermal radiation such that to make another object hotter than it. Or even more blatantly, to make an object able to heat itself up forever with its own thermal radiation! Also impossible -- therefore we can't do it.
More straightforwardly, it's the brightness theorem (from the Wikipedia again):
A consequence of the conservation of etendue is the brightness theorem, which states that no optical system can increase the brightness of the light emitted from a source to a higher value than the brightness of the surface of that source (where "brightness" is defined as the optical power emitted per unit solid angle per unit emitting or receiving area).
In this case the floor becomes the light source. This brightness cannot be increased -- if we reflected the light back onto the object and it became brighter (even a tiny bit), it would violate the brightness theorem.
To address the other answers in turn:
@Filip Milovanović: Despite being well-written and providing examples of reflections making objects brighter, the examples all focus on light from the initial light source being redirected to make a spot brighter than it otherwise would be -- but the question was about the light from the object itself reflected back at it.
@Martin 'Kvík' Baláž: It's a relevant calculation showing the effect would be small, but as above if we could do it in reality then it seems we'd violate some laws of physics. I would want to see a measurement of this in reality with said specialized equipment.
@benrg @appliedSciences: It is compelling that the effect would be hard to see, but, as per above, it seems impossible.
@Jason Goemaat: It's a relevant point that the angles may indeed be wrong, but as above it seems no combination of angles, mirrors, lenses (be they concave or convex), etc., would work.
@gomennathan: The only point not already covered is that a room with white walls is brighter than a room with black walls -- that is true but it can be explained by the white walls reflecting more light/becoming a brighter light source than a black wall, which can then in turn make further objects brighter than they would be -- but, not brighter than the walls themselves! In other words it also doesn't answer the question of reflecting the light from the wall back to itself to make the wall brighter.
There is perhaps an important caveat -- the light from the light sources that reflects due to specular reflection would continue to have the same etendue as the light from the light source, as opposed to the increased etendue from the diffuse reflections. This specular component therefore should be able to be reflected to increase the brightness of the floor. But in this case, it isn't the light from the floor per se making the floor brighter, rather it's a more efficient use of the light from the light source (that wasn't initially diffusely reflected) making the floor brighter.
I wasn't aware of these distinctions when initially writing the question, but I see that the spirit of the question was such as to be asking why light from an object that has only diffuse reflections, can't be reflected to make that object brighter -- or equivalently, light from a light source directly itself. @Martin 'Kvík' Baláž's answer, for example, does make this assumption of a perfectly diffuse (i.e. Lambertian surface).
To conclude, it seems like you ought to be able to do this, but you can’t trick nature and you can’t get free energy …
I am open to this being wrong, but I would want to see an example of this actually happening in reality in an observable way (using specialized equipment if needed), along with an explanation of why the above reasoning doesn't follow, to be convinced!