How can a photon collide with an electron?

Question

Whenever I study the photoelectric effect and the Compton effect, I have always had a question about how a photon can possibly collide with an electron given their unmeasureably small size. Every textbook I've read says that the photo-electrons are emitted because the photons collided with them. But since the photons and electrons virtually have no size, how can they even collide? I have searched for the answer on the internet but I couldn't find any satisfying one.

Answer 1

This is an answer by a particle physicist that has been working with data for forty years:

Photons and electrons are quantum mechanical entities, and to really really understand their interactions, quantum mechanics has to be invoked.

When detected, the photon has a point particle footprint (as does the electron) consistent with the axiomatic particle table of the standard model.

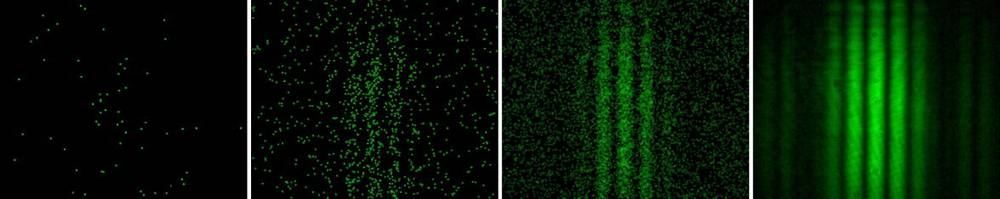
The leftmost frame shows the collision of a countable single photons on a screen (in a double-slit experiment).

The accumulation of photons (light emerges in a calculable manner from many photons), shows the wave nature's interference effects. It is the probability of landing on the (x,y) of the screen that displays a wave behavior. Not the individual photons.

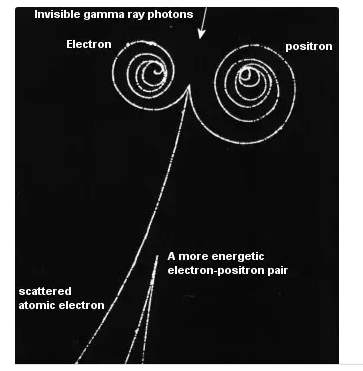
Here is another measurement of a photon

The original picture is here. That the single photon (gamma) electron interaction is at a point is evident.

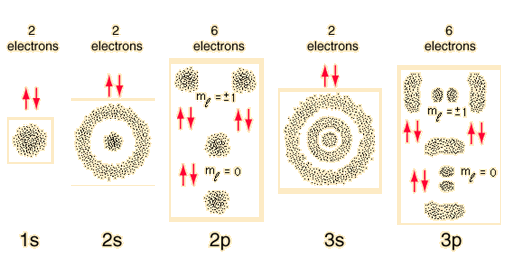
Now let us see how what we call size for macroscopic particles in quantum mechanics appears. It is all dependent on probabilities of a particle being at an (x,y,z) to interact with another particle. Look what an electron around a hydrogen atom has as a probable location:

This is what defines the macroscopic charge distribution, and the probability of an incoming gamma ray to interact with the electron is a mathematical combination of this, and the coupling constants of the quantum mechanical interactions.

A free electron has a very small probability to be hit by a photon. That is why high density beams are used in high energy experiments. In general it will be the coupling constants which will give high probabilities the closer the two point particles are, and of course not to forget Heisenberg's uncertainty principle, which also will define a volume in space and momentum where interactions can happen.

The photoelectric effect involves electrons that are in orbitals and a large number of atoms and molecules, and the fact that it exists means that there is enough probability for an incoming photon to hit an electron in the orbitals distributions of the specific solid.

Answer 2

It depends what you mean by "size". Light spreads out like a ripple in water so once that ripple reaches some object floating in the water it will disturb the object. Does the ripple have some definable "size"? It's just an ever expanding circle whose source is the center of the expanding circular ripple (maybe caused by you dabbing your finger in the water for example).

The particle nature of light is used as an explanatory device (just like its wave form is used as an explanatory device in diffraction) to describe the discrete amount of energy light carries. What's carrying it? Well the expanding circular wave front of course.

In conclusion, it is the electromagnetic wave front that collides with the electron carrying a packaged amount of electromagnetic energy called a "photon" just as it is the water ripple that impacts the floating object in the water.

And this package of energy, or photon, which is the energy of the wave must be great enough to excite the electron off its parent atom. If you want a further discussion of how exactly the photoelectric effect exhibits light's particle/wave duality I've answered it here: Photoelectric effect confusion

My answer on this question is solely meant to explain how light which is seemingly lacking a corpus (fancy word for "body") can impact something which does have a corpus (ie. a particle).

Answer 3

Both photons and electrons may be considered point-like particles, but the interaction/force that they feel has a range: the electromagnetic interaction has a pretty long range. Actually it is infinite in the absence of screening effects (ideal cases).

You could ask yourself, what does it even mean colliding? For example when you clap your hands, the atoms that form your skin do not collide or touch at all. It is just the "electric" repulsion that increases so much until you do not have the force in your muscles to overcome it.

Answer 4

Great question! You are correct in that an electron doesn't have a 'size' in the sense that it's not actually a little billiard ball sitting out in space. For that matter, neither is a photon. In physics we frequently switch back and forth between the wave and particle description of matter depending on which is a better description of the situation.

In the case of Compton scattering, we want to use momentum and energy conservation, so we don't need to look too closely at the interaction itself. Instead, just consider the initial state where you have an electron minding its own business and an inbound photon. And then the final state, where the photon has scattered in some new direction and the electron has picked up some momentum. In both the initial and final states, the photon and electron are far enough apart that they look like point particles. Then you can just solve the (relativistic) conservation of energy and momentum to get the Compton equation.

If you wanted to describe what is happening to the electron and photon during the 'collision', then you would probably have to treat them both as complex interacting quantum objects, integrating over their wavefunctions, etc.

Answer 5

Defining size on the quantum scale is tricky.

One way we might try would be to ask what is the minimum size a hole (an aperture) could be and still not disturb a photon or electron that passes through it.

The answer isn't too complicated. That aperture needs to be at least a few wavelengths wide in order to have only minimal diffraction effects.

Photographers often use as small of an aperture as possible in order to have a nice sharp focus even for different distances. But if the aperture is too small, they get diffraction effects that distort their image.

https://photographylife.com/what-is-diffraction-in-photography

Roughly speaking, to find out what will happen to a particle, you must find all the possible paths that a particle can take and add them up. Each path has a phase angle associated with it, and so the addition can have constructive and destructive interference results. This is what we usually associate with the wave nature of particles.

Usually, most contributions from each path will cancel out, leading to a classical path, but if there are important differences between in a small region of nearby paths, then quantum interference effects will be important. The size of that region depends on the wavelengths of the particles involved.

So when we imagine a photon and an electron interacting, you might then imagine the photon and the electron both have a continuum of possible paths that they can take, and while most paths don't intersect, those paths that actually do intersect have a critical contribution to the result of that sum over those paths.

I recommend reading Feynman's book QED: A Strange Theory of Light and Matter for a detailed (and fun) explanation of this path integral picture of quantum mechanics.

Answer 6

Indeed, the picture that one gets from a typical discussion of the Compton effect is far from realistic. Here are a few points to consider, if you are to do an actual experiment (the list is by now means exhaustive).

• Detecting a single photon or a single electron is hard (if not impossible) even with the modern equipment. Thus, in reality we are talking about many photons scattered from many electrons. This in fact is consistent with the Copenhagen interpretation of the quantum mechanics, as measurements done on a statistical ensemble.
• Photon is not a point-like particle, but a field. Many photons are an electromagnetic wave. Thus, we can view the interaction of an electron with a photon as an interaction of a point-like electron with an electromagnetic field periodic in space and time (this is actually the direct answer to the question).
• As I have already mentioned, many electrons are involved in an actual experiment, and electrons repel each other. Thus, one cannot really do such an experiment with free electrons, but rather with the electrons weakly bound on some material.

Answer 7

Let's start with a classical picture, where an electron possesses a negatively electric charges, $q=-e$, and light is an electro-magnetic field. Hence, in the classical description we expect that there exists an interaction between these two objects, because

• there exists an interaction between the electric field of the light and the charge, $\vec F = q\cdot \vec E$,
• there exists an interaction between the magnetic field of the light and the charge, $\vec F = q \vec v \times \vec B$,

Now, if we use a quantum picture and think of light as being composed of photons, we have to account for this interaction. This is done by using an interaction cross-section or interaction strength/amplitude. The math becomes involved -- its called quantum electro dynamics (QED).

Answer 8

It is very important to understand that you are asking about the absorption of a photon. Now if you try to imagine this as a classical collision of two balls, that is just not correct. You are confused because you think the photon needs to collide head on with a specific electron to get absorbed.

What is correct to say is that the whole QM system, the atom/electron system absorbs the photon.

Now you say that the electron that collides head on will absorb the photon. Let's take an atom with multiple electrons that are all able to absorb photons and move to higher energy levels.

What is correct to say is that the electron that will absorb the photon and move to a higher orbital will be the one that has a energy gap that is available for the electron to move to that matches the energy of the photon.

So these two QM entities, the photon (though the photon does not have a strict position observable) and the electron both have a probability distribution of being at certain places, and you are saying that if they collide head on, the electron will absorb the photon.

In reality, the atom/electron system will absorb the photon, and the specific electron that will move to a higher energy level will be the one that has an available energy gap that matches the photon's energy.

Answer 9

Welcome to the physics show! I approach this problem from a totally different viewpoint. Namely, the one that electrons and photons are no point particles at all (besides the fact that leptons and quarks are no elementary particles but let 's assume the electron is elementary; see for example the Rishon Model) and hidden variables.

I don't refer to string theory in which the same problem would occur as the one you are concerned with in your question. I know it's not mainstream but the more ideas the better and maybe it adds something useful!

Imagine a flat 2d (2-dimensional) space rolled up to a cylinder with a Planck radius. Particles are represented as 1d (of course also these circles have a Planck radius) circles on this cylinder as 1d circles. That is, their charge (whatever kind) is wrapped around the cylinder. As you can imagine particles can't miss each other in an encounter! Seen from a big distance the cylinder looks like a 1d line and the particles as point-like. In this case, there is obviously no difference between point and non-point particles. In both cases, they make an encounter.

Until now you can visualize it. 2d plane, curved in 2d space, from far away looking point line-like with point-like particles on them. What follows can't be visualized but is conceptual the same and it can be caught in math, not only this making of non-point-like particles but one can also apply the quantum mechanical math to them; in this respect, I'm not instrumental wrt the math ("shut up and calculate!"), but want to see the physics behind it (of which instrumentalists say that this is impossible).

Next step: "roll-up" a 3d flat space to obtain a 3d cylinder with a Planck radius. From afar (and inside it, in relation to huge structures of particles) the 3d cylinder looks like a 2d flat plane You can place 2d spheres on it in the same way you put a circle on the cylinder in the first case. The charges are smeared out over the 2d spheres. Like the circles (e.g. an electron and a photon) on the Planck sized cylinder always encounter (leptons like electrons, muons, quarks, etc. don't, only force particles and quarks and leptons) so do the 2d spheres on the 3d cylinder.

The last step, referring to the Universe around us. Again 1 space dimension higher: "roll-up" a 4d flat space in a flat 5d space. This time to obtain a 4d Planck radius cylinder. As you might guess we place 3d spheres on them (compactly filled with charge). And as you might guess from far away or inside the 4d cylinder it seems the cylinder is 3d, like the Universe we inhabit is. Again, the relevant particles can't miss each other, in the relevant situations.

The spaces in which we rolled up the 2d, 3d, and 4d flat spaces we can throw out of the window, in accordance with General Relativity (the seemingly 1d, 2d, and 3d cylinders have an inherent structure (like the curved space in GR).

You can apply the mathematical machinery of Quantum Field Theory on these theoretical Planck-sized particles and at the same time see the theory as non-instrumental.

Maybe one can even assume that the 4d, but from our perspective 3d space, consists out of (continuous) hidden variables that guide the particles and explain the probabilistic Nature of quantum mechanics. In this case, quantum gravity would be unnecessary simply because space(time) is, in this case, the reason for the probability Nature of quantum mechanics (like the probability Nature of the Brownian motion, which was later seen in the light of the before hidden atoms). If history took another turn, maybe the hidden variable theory was the now accepted interpretation of qm.