Planck's law
Until stars were formed a few hundred million years after the Big Bang (BB), the brightness of the Universe was extremely homogeneous and given by a near-perfect blackbody Planck spectrum with a temperature of $T = T_0(1+z)$, where $T_0=2.725\,\mathrm{K}$ is the current temperature of the CMB, and $z$ is the redshift corresponding to the time $t$. That is, the brightness at wavelength $\lambda$ is:
$$
B_\lambda(\lambda,T) = \frac{2hc^2}{\lambda^5} \frac{1}{e^{hc/\lambda k_\mathrm{B} T} - 1},
$$
where $h$, $c$, $k_\mathrm{B}$ are Planck's constant, the speed of light, and Boltzmann's constant, respectively.
Perceived brightness
I'm going to assume that you're human, and hence that the brightness you're interested in is the optical wavelength region, i.e. around $\lambda\sim550\,\mathrm{nm}$. The peak of a Planck spectrum shifts toward higher frequencies the higher the temperature is, and hence the ratio of optical-to-UV/X-ray/gamma radiation will decrease. But regardless, the absolute brightness will always increase at any wavelength for larger temperatures.
At $t\simeq10\,\mathrm{s}$, what later becomes our observable Universe was already $\sim30$ light-years in radius (although the observable Universe of that epoch was only 8000 km). The scale factor (ratio of the size at that time to the current size) was thus $a\sim7\times10^{-10}$, the corresponding redshift $z\sim1.4\times10^9$, and hence the temperature of the Universe was $T \sim 3.7\times10^9\,\mathrm{K}$.
Plugging into the equation above, and dividing by $4\pi$ to get the brightness per solid angle, I get that the brightness in the optical was
$$
B_\lambda(550\,\mathrm{nm},3.7\times10^9\,\mathrm{K}) \simeq 3\times 10^{19}\,\mathrm{W}\,\mathrm{m}^{-3}\,\mathrm{sr}^{-1}.
$$
So, what does this number mean? To get a feeling of how it would look, we can compare the amount of light received from a human field of view, to the light received when looking directly at the Sun. Andersen et al. (2018) did exactly this, in order to calculate the period of time that a human could see anything in the early Universe. They found that while the Universe became too dim for a human to sense any light around $t=5.7$ million years after BB, it was as bright as looking at the Sun when the Universe was around $T\simeq1600\,\mathrm{K}$, just over 1 million years after BB, and so had a brightness in the optical of around $B_\lambda(550\,\mathrm{nm},1600\,\mathrm{K}) = 1.5\times 10^7\,\mathrm{W}\,\mathrm{m}^{-3}\,\mathrm{sr}^{-1}$, or a factor of $1.7\times10^{12}$ times smaller than at $t\simeq10\,\mathrm{s}$.
In other words, ten seconds after the Big Bang the Universe was a trillion times brighter than looking at the Sun.
Brightness today
Today, the spectrum of the Universe is no longer a Planck spectrum, but is given by a mixture of cosmological and astrophysical processes. In this answer about the cosmic background radation, you can see that the brightness of the optical peak is roughly two orders of magnitude dimmer than the CMB peak brightness which, in turn, from Planck's law today has a brightness $\sim10^{24}$ times smaller than at $t\simeq10\,\mathrm{s}$. "Optical light" is here defined as a much broader region than what a human would see is, very roughly ten times as broad, so the perceived brightness would be another order of magnitude lower. Hence, today the Universe is 27 orders of magnitude less bright than at the photon epoch.
Brightness and color through the history of the Universe
The figure below shows, in green, the brightness of the CMB as a function of time after the Big Bang. A secondary $x$ axis on top show the corresponding temperature of the Universe. The background color shows the color of the Universe as would be perceived by a human being, calculated by convolving the spectrum of the radiation with the response function of the human eye: The first few tens of thousands of years, the Universe is a pale sapphire blue, turning white as it reaches the temperature of the Sun ($T_\odot \simeq 5\,780\,\mathrm{K}$). At $t\sim200\,\mathrm{Myr}$, stars begin to form and the calculation of the spectrum becomes more complicated (so I've grayed it it out), but today the Universe has reached a cosmic latte (Baldry et al. 2002). Note that, as mentioned above, only between $t\sim1\,\mathrm{Myr}$ and $t\sim6\,\mathrm{Myr}$ — where the temperatures was $1600\mathrm{K} \gtrsim T \gtrsim 500\mathrm{K}$ — could you actually see anything; prior to this epoch you'd go blind, and after this epoch, it'd be too dim (but you could in principle still see the color using sunglasses/binoculars, respectively).
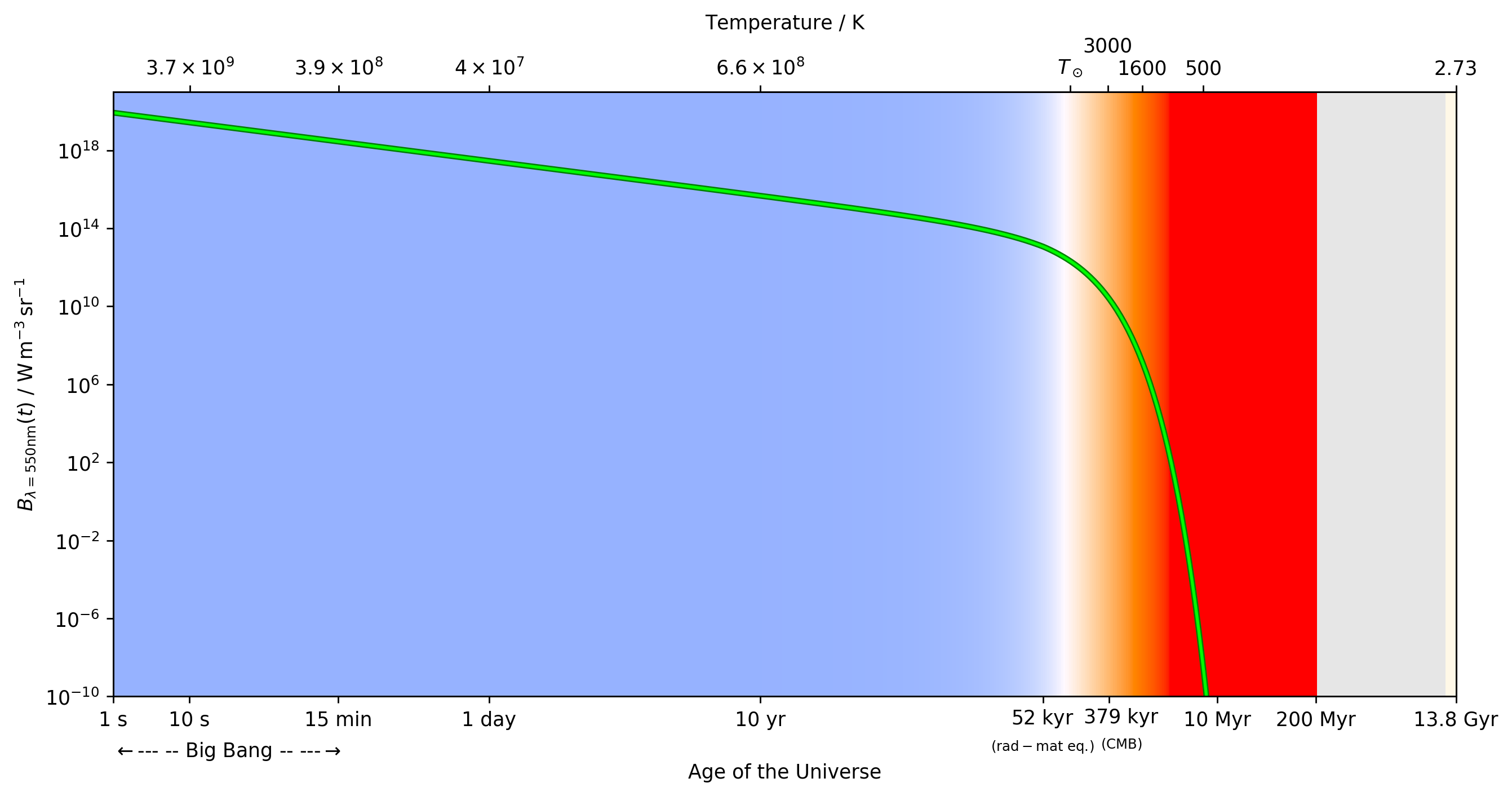
At $T\lesssim hc/\lambda k_\mathrm{B} \simeq 30\,000\,\mathrm{K}$ the exponential factor in the Planck law blows up so the brightness at $\lambda$ quickly decreases. The brightness is further decreased by the fact that, at $t\sim52\,\mathrm{kyr}$, the Universe transitions from being radiation-dominated to being matter-dominated and so the expansion goes from $a(t)\propto t^{1/2}$ to $a(t)\propto t^{2/3}$, i.e. faster.
At the time of recombination ($t\sim379\,\mathrm{kyr}$), the Universe is somewhat brighter than at $t\sim1\,\mathrm{Myr}$. Here, $T\sim3000\,\mathrm{K}$, so $B_\lambda(550\,\mathrm{nm},3000\,\mathrm{K}) = 3\times 10^{10}\,\mathrm{W}\,\mathrm{m}^{-3}\,\mathrm{sr}^{-1}$, or a billion times less bright than at $t\simeq10\,\mathrm{s}$.
A note on decoupling and mean free path
Before the photons decoupled from matter, they scattered frequently on free electrons, so their mean free path was small compared to the size of the (observable) Universe. It is often said thad the Universe was "foggy" until decoupling, but I think people often overestimate this fogginess. The mean free path is $\ell = 1 / n_e \sigma_\mathrm{T}$,
where $n_e$ is the number density of free electrons and $\sigma_\mathrm{T}\simeq6.65\times 10^{-25}\,\mathrm{cm}^2$ is the Thomson cross section of the electron. Calculating $n_e$ from the ionization state of the gas, this works out$^\dagger$ to roughly 2000 light-years just before recombination begins to kick in at a time $t\simeq200\,000\,\mathrm{yr}$ after BB, 20 light-years at $t\simeq50\,000\,\mathrm{yr}$ when matter started dominating over radiation, 16 kilometers at $t\simeq15\,\mathrm{m}$ when nucleosynthesis ended, and 20 meters at $t\simeq10\,\mathrm{s}$, when leptons and antileptons annihilated and the photon epoch began.
But this scattering is not really important for how bright the Universe were. Photons arrive at your eye all the time, and if they have scattered multiple times from their point of origin, you won't know where they were created, but you will still see them.
$^\dagger$I wrote a Python code called timeline to calculate $\ell$ and other quantities of the Universe as a function of time, available on GitHub here.