What is the Andromeda Paradox?

Question

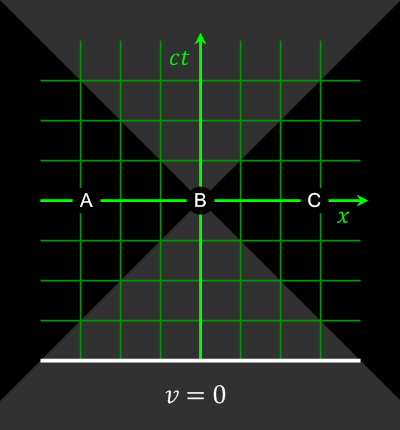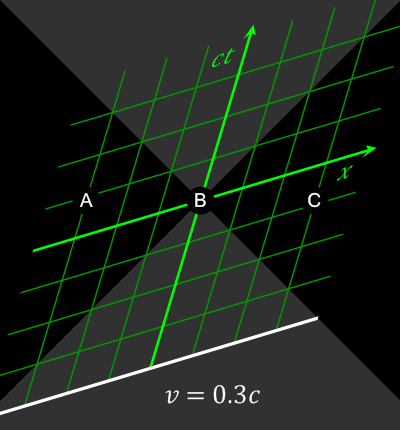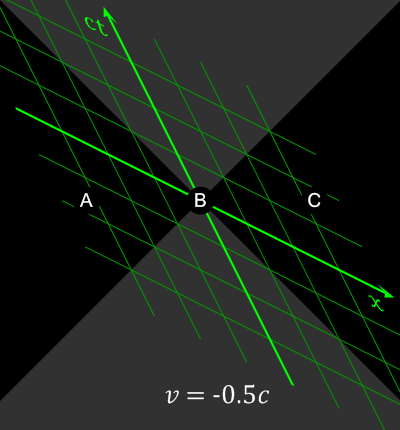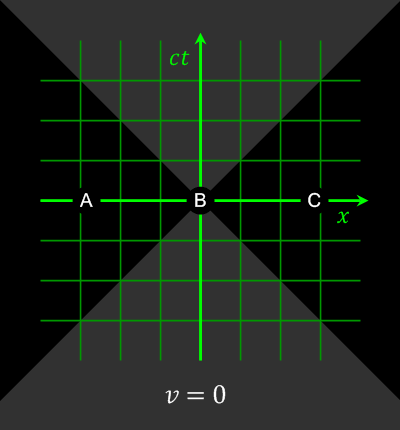
I have been studying causality (specifically why there is no such thing as a simultaneous instant of time across all observers) recently and I keep hearing references to the Andromeda paradox. Can anyone tell me what it is and how it is resolved?

I've tried reading what Wikipedia says about it, but I could really use someone's explanation.

Edit
Ok, after reading the related question, now I'd like to know does this imply there is some sort of privileged reference frame, in which there exists absolute velocity and absolute time? I ask because the answer for the other question seemed to indicate that the actions seem to be at different points of simultaneity but that in fact the person not moving is getting it right. But this seems strange to me because if we accelerated our galaxy to a different speed, we would still perceive our stationary observer as being right even though they'd see something different than before the acceleration.

So who is right? In what frame can we be sure that our present is Andromeda's present?

Answer 1

There can be at least two different flavors of paradoxes. In one, a result such as 2+2=5 is proved, and the problem must be either incorrect reasoning or a set of assumptions that was invalid. In the other type, exemplified by the EPR paradox, the correct result of an argument is so surprising that it seems like it must be a mistake.

Based on the description in the quote by Penrose, the Andromeda paradox is of the latter type. It is simply a dramatized example of the relativity of simultaneity, which is contrary to our intuition based on everyday life. In Penrose's presentation, the dramatization includes a summary of an imaginary dialog between the two observers, who seem to be confused because they don't understand relativity and expect simultaneity to be absolute. Penrose also comments that logical consistency is retained because events in Andromeda can only be learned about on Earth much later.

The paradox is both trivial and nontrivial. It's trivial because it's simply a description of how the relativity of simultaneity works, and therefore it doesn't require any complicated arguments to resolve. It's nontrivial because the relativity of simultaneity is a surprising and difficult concept when you encounter it for the first time.

It may help if we note that this would be the 2+2=5 type of paradox if it were possible to transmit signals instantaneously in relativity. Then the two observers would be disagreeing about something that they could easily check by faster-than-light communication. One of them would find out that he was wrong, and his frame of reference would have been found to be invalid -- which contradicts the basic assumptions of relativity.

Answer 2

I put my negative comment in the from of the answer. Here are the very basics of the simultaneity implications (or causes) of relativity:

According to the special theory of relativity, it is impossible to say in an absolute sense whether two distinct events occur at the same time if those events are separated in space, such as a car crash in London and another in New York. The question of whether the events are simultaneous is relative: in some reference frames the two accidents may happen at the same time, in other frames (in a different state of motion relative to the events) the crash in London may occur first, and in still other frames the New York crash may occur first. However, if the two events are causally connected ("event A causes event B"), the causal order is preserved (i.e. "event A precedes event B") in all frames of reference.

Events A, B, and C occur in different order depending on the motion of the observer. The white line represents a plane of simultaneity being moved from the past to the future.

If we imagine one reference frame assigns precisely the same time to two events that are at different points in space, a reference frame that is moving relative to the first will generally assign different times to the two events. This is illustrated in the ladder paradox, a thought experiment which uses the example of a ladder moving at high speed through a garage.

I see that this paradox is a basis of Einstein's theory. It is used to explain rather than to confuse. Once you understand that your daily experience of simultaneity is wrong, that there is no simultaneity between the spatially-separated places, then you should wonder: "where is paradox?". But, I guess the internet-banned people are already asking another question: "what is the ladder paradox?". Why do you ask for Andromeda paradox but not for explanation of velocity-related simultaneity? What is the question?

Answer 3

As long as you don't forget that Andromeda galaxy is cca. 2.5million light years away, then there should be no paradox at all. The observers are only seeing different slices of history that took place 2.5million years ago (plus/minus 1day), the decisions has already been made long time ago. It's like when you take a newspaper from a pile, more recent papers being on the top, the person behind you (the slower one) in the queue would take the older newspaper, your partner and anyone with you - you all would read the same - more recent paper. If you would move straight towards the Andromeda at any speed, then you'd just be skipping the queue, in any case you are only reading history in your paper, not the future... I know the question is bit old, sorry about bumping...