0
$\begingroup$

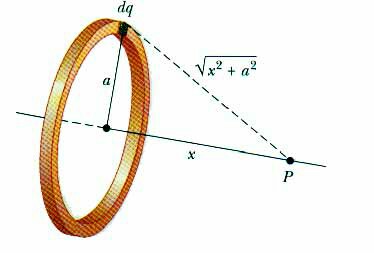
enter image description here

Suppose we have to find out potential $V$ at point $P$ in the given figure. I know that it can be easily done as

$$dV=\frac{k×dq}{\sqrt{(x^2+a^2)}}$$ and integrating $dq$ to $Q$. Where $k$ is a constant such that $k=\frac{4}{10^9}$. And the correct answer will be $$V=\frac{k×Q}{\sqrt{(x^2+a^2)}}$$

But my question is, I have studied that potential at any point can also be given by $$V=-\int{\vec{E}.\vec{ds}}$$. .......$(1)$

And also value of $E$ for ring at point $P$ is given as $$E=\frac{k×x×Q}{\sqrt{(a^2+x^2)^3}}$$. So by using equation $(1)$

$$V=-\int{\frac{k×x×Q.ds}{\sqrt{(a^2+x^2)^3}}}$$.

But I won't understand what is being varied i. e, which varying quantity is being expressed by $ds$. Is it is the $x$ (distance from centre along axis of ring). Suppose I assume that $x$ is being varied then I can write $ds$ as $dx$.

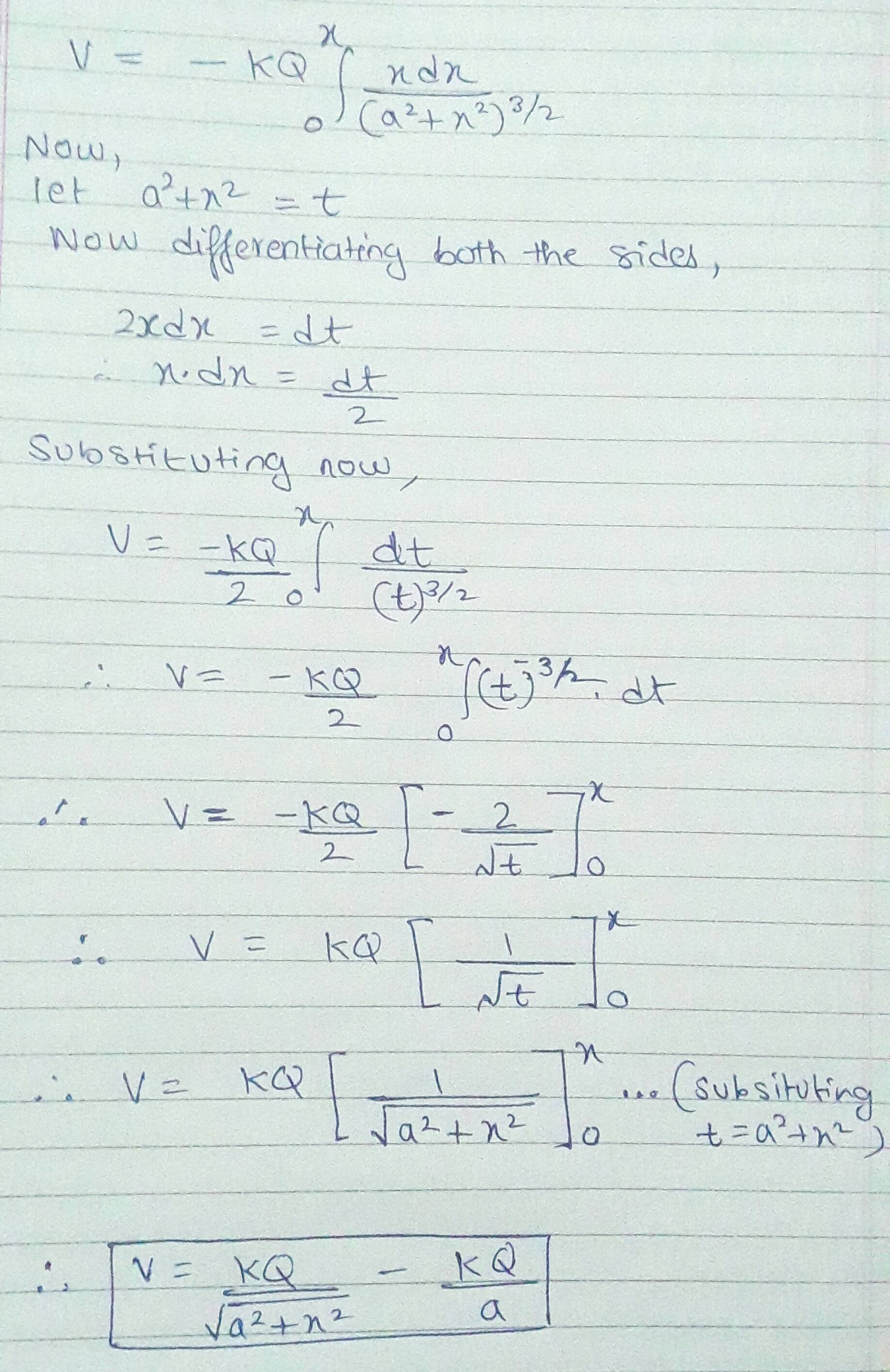
$$V=-k.Q\int_0^x{\frac{xdx}{\sqrt{(a^2+x^2)^3}}}$$.

But this gives me different answer than the correct one. I know that I have done something very wrong. Also I am not sure with the limits I have taken i.e from $0$ to $x$. Please help me identifying my mistake.

Responding to @Floris's comment, I have solved it as

enter image description here

This is that different answer. I think that my integration limits have went wrong, but I don't know the correct one. Now please help me.

Improve this question
$\endgroup$
5
  • $\begingroup$ Did you try computing the integral? How does it give you a different answer? $\endgroup$
    – Floris
    Commented Mar 27, 2017 at 18:14
  • 1
    $\begingroup$ OK wait I will write my attempt in the question. $\endgroup$
    – Avi
    Commented Mar 27, 2017 at 18:15
  • $\begingroup$ But first tell me that I am integrating it from 0 to x, Is it correct? Because I think there should be something different from x $\endgroup$
    – Avi
    Commented Mar 27, 2017 at 18:26
  • $\begingroup$ Layout tip: Put the full stops inside the double-dollar-signs to avoid having them flying around. $\endgroup$
    – Qmechanic ♦
    Commented Mar 27, 2017 at 18:31
  • $\begingroup$ Did you mean the full stops which represents multiplication? $\endgroup$
    – Avi
    Commented Mar 27, 2017 at 18:33

2 Answers 2

Reset to default
1
$\begingroup$

The answer you have calculated is correct, but it is not the potential at distance x from the center. It is the potential difference between the points at x=0 (center at the plane of the ring) and at a point x. This is the same result you will end up if for the initial formula for V you set x=0, that is you will get at x=0 a potential V=kQ/a. The formula V=-Integral(E.ds) holds for the potential difference estimated at the limits of the integration.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So it means that I should use my second method only when I want the difference in potential at two different points, otherwise for finding potential at a point I should use my first method. Am I correct? $\endgroup$
    – Avi
    Commented Mar 28, 2017 at 5:57
  • $\begingroup$ The potential at any point is calculated with respect to a point where the potential is considered to be zero. Thus, it is always calculated with respect to such a point where V=zero. In your case you may consider V=0 when x->+oo. Both methods are equivalent. $\endgroup$
    – SteveTheGrk
    Commented Mar 28, 2017 at 17:34
1
$\begingroup$

The problem is just with your limits - otherwise you did fine.

Potential as calculated with your first expression is relative to infinity (as you can see the potential is 0 when $x\rightarrow \infty$)

To get the same result with your second method you just have to integrate between $x$ and $\infty$, taking care of signs.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.