0
$\begingroup$

I understand from Faraday's Law of induction that any change in the magnetic flux acting on a conductor should force the charge carriers there to accelarate.

So far,all the examples I have found were about solenoid coils and transformers. I tried to make up an equation with poor math skills.

Here it is:

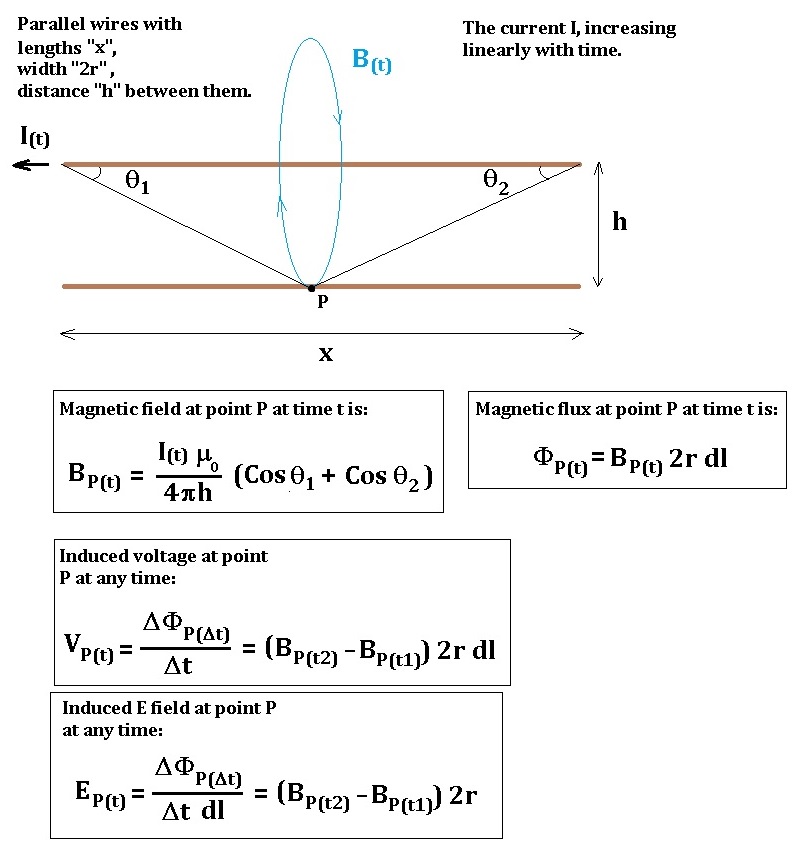enter image description here Could we calculate the induced E field correctly with this equation?

I'm not sure about the direction of the induced E. Maybe as in transformers, it would be in opposite direction with the current which induced it.

Improve this question
$\endgroup$
5
  • $\begingroup$ And if the current in the primary cable is decreasing, would the induced E field be in the same direction? $\endgroup$
    – Xynon
    Commented Feb 23, 2017 at 17:49
  • $\begingroup$ If the current in the primary cable is increasing, the direction of induced current in the other wire Will be in the opposite way of which the primary current is inreasing in. If the current stays the same, the current in the other wire Will stop flowing. If the current starts decreasing in the primary wire, it Will start to again flow in the opposite way from which the primary current is decreasing in. So in those 2 cases it Will change direction. So in which ever way the current is changing, the induced current Will be in the opposite way of that current. $\endgroup$
    – MaDrung
    Commented Feb 24, 2017 at 6:46
  • $\begingroup$ I meant in the change of current, not current. Sorry for confusing you. $\endgroup$
    – MaDrung
    Commented Feb 24, 2017 at 6:53
  • $\begingroup$ That equation which you derived gives the value of net electric field at any moment in time, maybe calculus can be used to sum all those fields over time. $\endgroup$
    – Sarthak Sharma
    Commented Feb 24, 2017 at 7:05
  • $\begingroup$ But the E field is already constant at all times, as long as the inducing current keeps rising at a steady rate. Becuase the induced E field is proportional to the [change in current] / [change in time]. $\endgroup$
    – Xynon
    Commented Feb 24, 2017 at 7:48

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.