Charge between parallel plates given voltage

Question

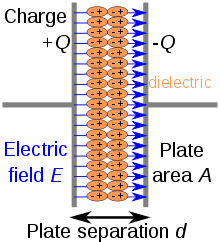
When you connect a parallel-plates capacitor to a voltage source, why is it assumed that the plates will have equal but opposite charge?

According to the formula, the voltage only fixes the charge difference between the plates. Integrating inside the capacitor we have:

$$V = \int E \ dx = \frac{\sigma_1 - \sigma_2}{2 \epsilon_0}d.$$

I know that the electric field should be 0 at infinity, but I keep in mind that the electrodes are (large but) finite, so, this is always the case.

You can argue that there is inversion symmetry in the setup. Then the question is: will the plates still have opposite charge if we enclose the capacitor inside an irregular metallic box of fixed charge $Q$? (Thus without disturbing the electric field inside the box).

Answer 1

A capacitor is assumed to be self-contained and isolated, with no net electric charge and no influence from any external electric field. The conductors thus hold equal and opposite charges on their facing surfaces.

As electric field is established which originate from the positive plate and end on the negative plate. Also, the field is uniform so is the solution.

Answer 2

I stumbled upon this similar question. This OP is more general and I found the way to extend the answer.

Apparently the plates are coupled to the environment through a capacitance as well. By superposition we have

$Q_1 = Q_{12} + Q_{10},$

$Q_2 = -Q_{12} + Q_{20},$

$Q = Q_0 = -Q_{10} - Q_{20},$

where the index $i = 0, 1,2$ corresponds to the $i$th plate ($0$ is the environment). It holds:

$Q_{12} = C_{12} (V_1 - V_2),$

$Q_{i0} = C_{i0} (V_i-V_0).$

We can solve these equations to find $Q_1$ and $Q_2$ (and $V_0$).

In experiments one of the plates is grounded (as well as the environment), which means that we will never have exactly opposite charges on them, having the case of the given link at the beginning. But in real life $C_{i0} << C_{12}$, thus this issue can be neglected.