- The Bondi k-calculus (an algebra-based method) develops the basic ideas of special-relativity in the $tx$-plane (without using the transverse direction). This approach is presented in his book “Relativity and Common Sense” (1962, 1964). In addition, Bondi presented “E=mc2: Thinking Relativity Through”, a series of ten lectures on BBC TV running from Oct 5 to Dec 7, 1963. (Read more at my contributed article to a blog at https://www.physicsforums.com/insights/relativity-using-bondi-k-calculus/ )
I have used it to develop the "longitudinal light clock" without appealing to the standard textbook "transverse light clock". In particular, I draw the light signals in the longitudinal light clock to form the "light-clock diamonds" (the "causal diamond" between consecutive tick events). All light-clock diamonds for all inertial observers have equal area since the Lorentz boost transformation has determinant one.
This is developed using the Bondi k-calculus in my article:
Relativity on rotated graph paper,
AmJPhy 84, 344 (2016); https://doi.org/10.1119/1.4943251 .
An early draft is at https://arxiv.org/abs/1111.7254 .
Rather than use "time-dilation" from the transverse clock,
I use the Principle of Relativity in the $tx$-plane.
The key figure (using $v=(3/5)c$) is
Once the light-clock diamond size is established (using the Bondi k-calculus in the above), the spacelike-diagonal of the light-clock diamond determines the reflection events on the mirror worldlines of the longitudinal light-clock. So, draw through those events parallels to the timelike diagonal (the observer's worldline). This shows length-contraction as viewed in the lab frame. (The construction can be shown to be symmetric between the observers.)
- I have a different approach (that doesn't use the Bondi $k$-calculus directly) which appears in my recent contributed chapter
Introducing relativity on rotated graph paper
Ch 7 in Teaching Einsteinian Physics in Schools
Kersting and Blair, Routledge 2021, https://doi.org/10.4324/9781003161721
I will describe it below.
You can play with the ideas in this visualization:
https://www.geogebra.org/m/HYD7hB9v#material/UBXdQaz4 (make sure BOB's diamonds are shown)
The key idea is that the diamond size is determined by the Principle of Relativity. (The diamond shape is determined by the Speed of Light Principle and the velocity of the observer.)
The two observers perform the same experiment
and should expect the same results:
2 seconds after they meet, send a light signal to the other.
Assuming absolute time and absolute space fails to satisfy the principle, but the third configuration works.
Using $v=(3/5)c$... and assuming the Speed of Light Principle.. we have the shape of Bob's diamonds... but what is the correct size?
Assuming absolute time (so that the heights of the diamonds are equal),
Alice (red) receives Bob's signal at 3.2, whereas Bob (blue) receives it at 5.
Bob's ticks need to be scaled up from this size.
This hints that there is time-dilation... but by how much?

Assuming absolute space (so the lengths of the light-clocks are equal),
Alice (red) receives Bob's signal at 5, whereas Bob (blue) receives it at 3.
Bob's ticks need to be scaled down from this size.
This hints that there is length-contraction... but by how much?
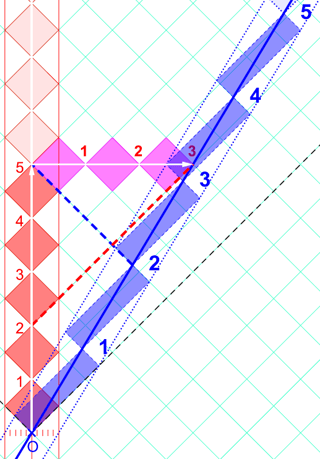
By playing around (taking a hint from the geometric mean?),
we get agreement with the Principle of Relativity by each receiving the other signal at 4 ticks.
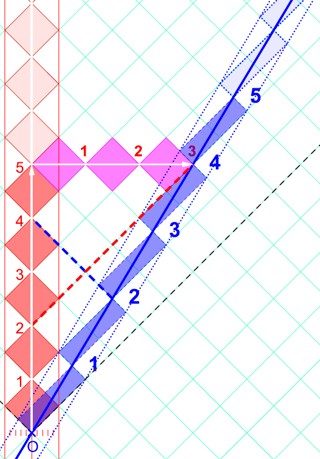
The ratio $(4\mbox{ ticks})/(2\mbox{ ticks})$ is the Doppler factor $k=2$ for $v=(3/5)c$,
where we have used the Principle of Relativity and the Speed of Light Principle...
...and, as a consequence, we now know the factor for time-dilation and length-contraction.
Try it for $v=(4/5)c$.
https://www.geogebra.org/m/kvfsq664 (updated)... make sure BOB's diamonds are shown
By the way, we find that the areas of Alice's clock diamonds are equal to Bob's clock diamonds. It turns out that the area of a causal diamond (in units of clock-diamonds) is equal to the square-interval between the corners of its diagonal.
For more information, consult my article and chapter above.
See also
https://www.physicsforums.com/insights/spacetime-diagrams-light-clocks/
https://www.physicsforums.com/insights/relativity-rotated-graph-paper/

