- The Bondi k-calculus (an algebra-based method) develops the basic ideas of special-relativity in the $tx$-plane (without using the transverse direction).
This approach is presented in his book “Relativity and Common Sense” (1962, 1964). In addition, Bondi presented “E=mc2: Thinking Relativity Through”, a series of ten lectures on BBC TV running from Oct 5 to Dec 7, 1963.
(Read more at my contributed article to a blog at https://www.physicsforums.com/insights/relativity-using-bondi-k-calculus/ )
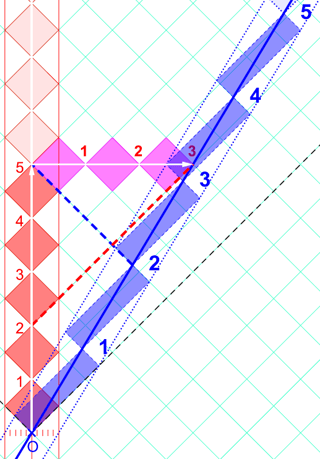
Using $v=(3/5)c$... and assuming the Speed of Light Principle.. we have the shape of Bob's diamonds... but what is the correct size?
Assuming absolute time (so that the elapsed timesheights of the diamonds are equal),
Alice (red) receives Bob's signal at 3.2, whereas Bob (blue) receives it at 5.
Bob's ticks need to be scaled up from this size.
This hints that there is time-dilation... but by how much?

Assuming absolute space (so the lengthlengths of the light-clock is the sameclocks are equal),
Alice (red) receives Bob's signal at 5, whereas Bob (blue) receives it at 3.
Bob's ticks need to be scaled down from this size.
This hints that there is length-contraction... but by how much?