$\vec E $ is identical to $E_x \hat i +E_y \hat j+ E_z \hat k$. Right?
So, ${\rm d}\vec{E}={\rm d} E_x \hat i +{\rm d}E_y \hat j+ {\rm d} E_z \hat k$.
If I say now that your vector integral is actually sum of three different scalar integrals, would you agree?
$$\int{\rm d}\vec E = \int{\rm d} E_x \hat i +\int{\rm d}E_y \hat j+ \int{\rm d} E_z \hat k$$
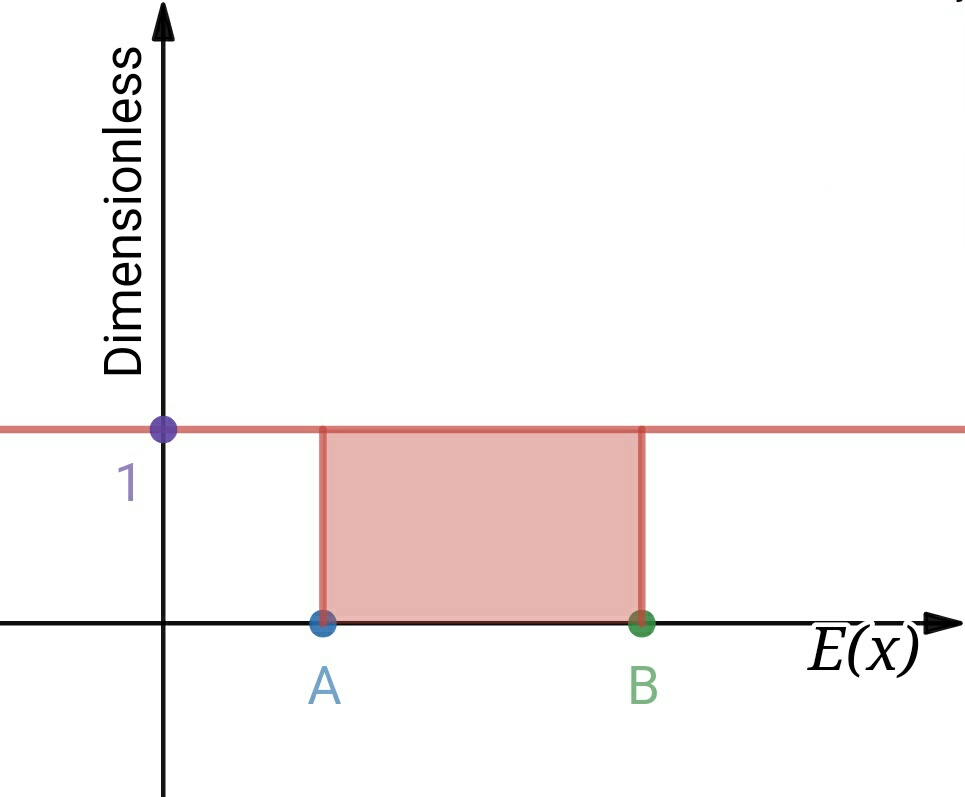
Now take a look at the graphs of $\int_{\small A}^{\small B} 1\times {\rm d}\vec E$, where I've assumed $A$ and $B$ be the magnitudes of electric fields in the $+^{ve} \space x$ direction due to infinitesimally small elements of rod at points $a$ and $b$ respectively.
Don't you think the area represents $1\times\Delta E_x=\Delta E_x$?
Same would be the analysis of $E_y$ and $E_z$.
Hence, $$\int{\rm d}\vec E = \int{\rm d} E_x \hat i +\int{\rm d}E_y \hat j+ \int{\rm d} E_z \hat k\\ = \Delta E_x \hat i +\Delta E_y \hat j+ \Delta E_z \hat k = \\ \Delta \vec E$$
And if you take for granted, that there is no field in absence of the rod (which logically do makes sense), we would arrive at a place where $$\Delta \vec E = \vec E$$