$\vec E $ is identical to $E_x \hat i +E_y \hat j+ E_z \hat k$. Right?
So, ${\rm d}\vec{E}={\rm d} E_x \hat i +{\rm d}E_y \hat j+ {\rm d} E_z \hat k$.
If I say now that your vector integral is actually sum of three different scalar integrals, would you agree?
$$\int{\rm d}\vec E = \int{\rm d} E_x \hat i +\int{\rm d}E_y \hat j+ \int{\rm d} E_z \hat k$$
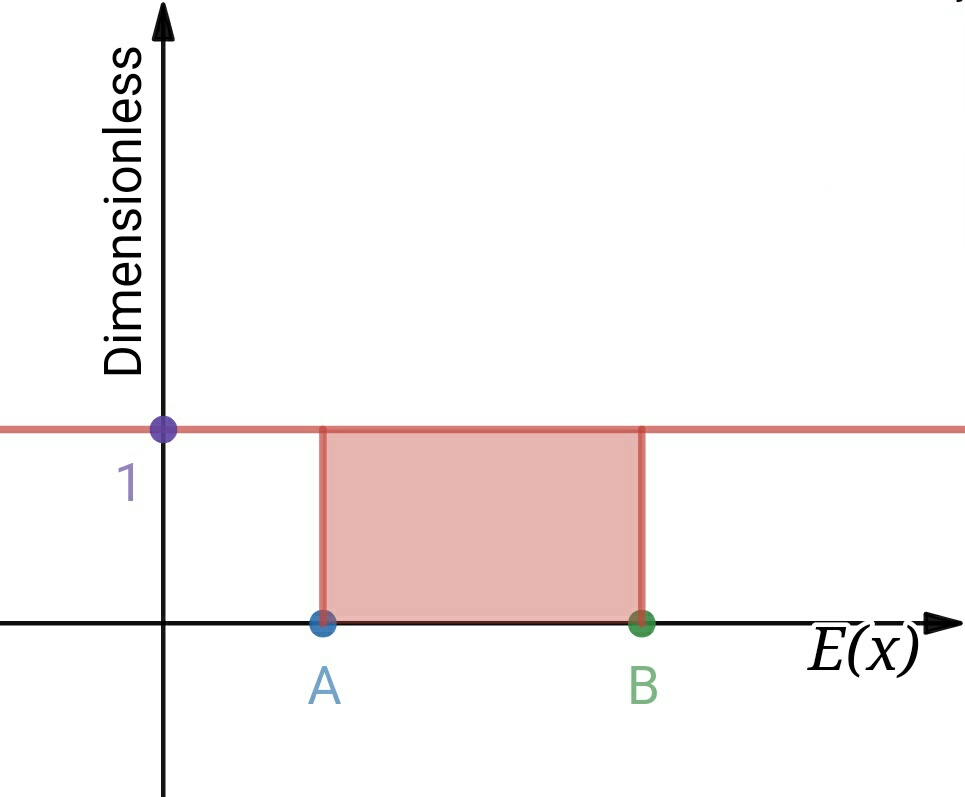
Now take a look at the graph of $\int_{\small A}^{\small B} 1\times {\rm d} E_x$, where I've assumed $A$ and $B$ be the magnitudes of electric fields in the $+^{ve} \space x$ direction due to infinitesimally small elements of rod taken near points $a$ and $b$ respectively.
Don't you think the area represents $1\times\Delta E_x=\Delta E_x$?
Same would be the analysis of $E_y$ and $E_z$.
Hence, $$\int{\rm d}\vec E = \int{\rm d} E_x \hat i +\int{\rm d}E_y \hat j+ \int{\rm d} E_z \hat k\\ = \Delta E_x \hat i +\Delta E_y \hat j+ \Delta E_z \hat k = \\ \Delta \vec E$$
And if you take for granted, that there is no field in absence of the rod (which logically do makes sense), we would arrive at a place where $$\Delta \vec E = \vec E$$
Edit
@Kalcifer 's comments forced me to add following edits.
What I think from your comments is that you have understood why this integral $\int_a^b {\rm d}\vec E$ represents $\vec E$, but are unable to follow up with how this represents what it does. So here it goes,
Once you have successfully written a vector $\vec A$ in terms of its components in mutually perpendicular directions (say x, y and z), you arrive at
$$\Delta A_x = \int_{A_{a_x}}^{A_{b_x}} {\rm d}A_x\\ \Delta A_y = \int_{A_{a_y}}^{A_{b_y}} {\rm d}A_y\\ \Delta A_z = \int_{A_{a_z}}^{E_{A_z}} {\rm d}A_z$$
This is because of independency of vector components along mutually perpendicular directions. Also, you must keep in mind that component of a vector along any direction is a scalar quantity. They are magnitudes. And the unit vectors are just constants whose presence in any way do not interfere with the calculation of magnitudes in that particular direction. But to avoid any confusion, let's leave them alone.
Don't you think the above written three integrals are nothing more than just an abridged from of the Fundamental theorem of Calculus?
So, $$\Delta \vec A =\Delta A_x \hat i + \Delta A_y \hat j + \Delta A_z \hat k \\ = \int_{A_{a_x}}^{A_{b_x}} {\rm d}A_x \hat i + \int_{A_{a_y}}^{A_{b_y}} {\rm d}A_y \hat j+ \int_{A_{a_z}}^{E_{A_z}} {\rm d}A_z \hat k\\ \Rightarrow \Delta\vec A=\int_a^b {\rm d}\vec A $$
About the constant function 1
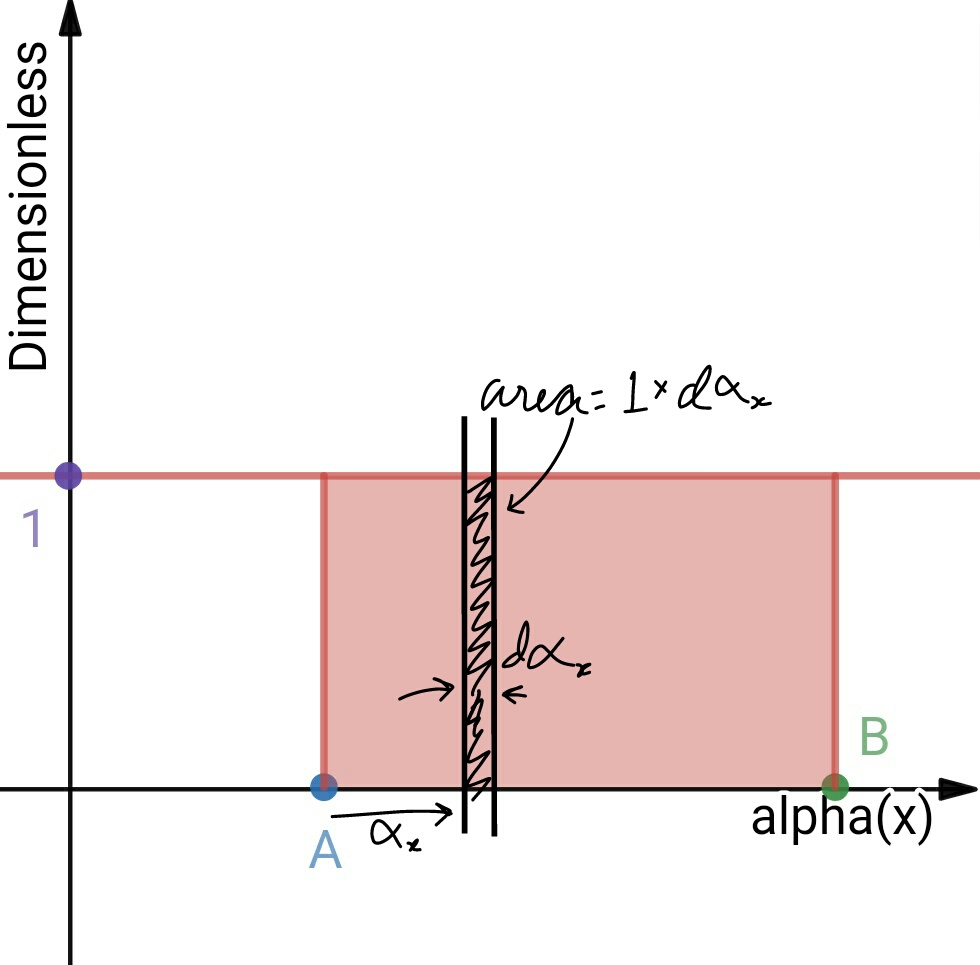
Mathematics gives you power so that you can write any scalar quantity, say $B$ as $$B= \beta \space (some \space units)$$ Where $\beta$ is numerical magnitude and is accompanied by the units specifying what quantity it is and in what scale it is measured. Similarly, any vector $\vec A$ can be represented as $$\vec A= \vec\alpha \space (some \space units)$$ Where $\vec\alpha$ is a dimensionless vector which says how large is $\vec A$ and is pointing in which specific direction and is accompanied by some units specifying what quantity it is and in which scale is it measured. So, our scalar integral (say of x component) converts to
$$A_x = \int {\rm d}A_x = \int 1 \times {\rm d}\alpha_x \space (some \space units)$$$$A_x = \int {\rm d}A_x\\ = \int 1 \times {\rm d}\alpha_x \space (some \space units)$$
So we are left with the integral of a dimensionless quantity ${\rm d}\alpha_x$ to muddle with. Wouldn't it represent the sum of areas of small rectangles of width 1 and length ${\rm d}\alpha$ (infinitesimally small)? Wouldn't this sum be represented as $$\lim_{n \to \infty}\sum_{i=1}^n 1\times {\rm d}\alpha_x\\ =1\times\alpha_x$$ So from this, what do we infer about "1".
We can clearly see that, "1" has no physical significance but it's presence could mathematically be justified and explained. It holds an importance in graphical representation of Riemann integral but is devoid of any importance in the physical aspects of integration. Even in graphical representation, it is there to formulate a dimensionless unitary area whose sum would yield the required Riemannian sum.
In simple words, the number "1" exists there as the universal factor and would keep on existing whether we like it or not.
Note: In the above graph, both the axes have quantities that are dimensionless.