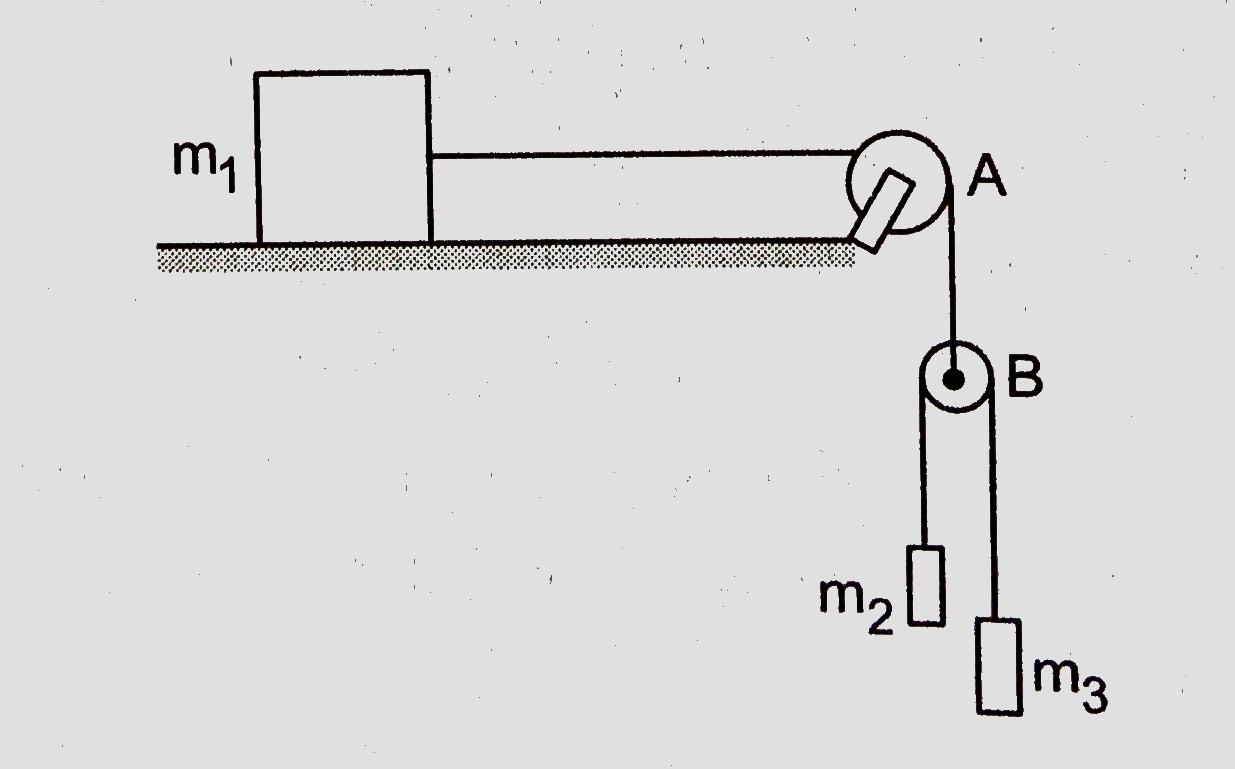
Three blocks of masses m1, m2 and m3 are connected as shown in the figure. All the surfaces are frictionless and the string and pulleys are light. Find the acceleration of the block of mass m1.

In this problem I know acceleration of the pulley B is same as acceleration of the block of mass $\ {m_{1}}$. But acceleration of bodies with mass $\ {m_{2}}$ and $\ {m_{3}}$ will be different since $\ {m_{2}}$ not equals $\ {m_{3}}$. So, I know that I cannot consider this(Pulley B, Blocks of mass $\ {m_{2}}$ and $\ {m_{3}}$) as a single system. But If the imagine putting those three objects in a box such that what's happening inside will not be visible to me then why I cannot consider this as a single system? The mass will thus be $\ {m_{2}}$ + $\ {m_{3}}$ and acceleration will be that of the block of mass $\ {m_{1}}$. So, I tried to find out the acceleration and I got $a=\dfrac {\left( m_{1}+m_{2}\right) g}{m_{1}+m_{2}+m_{3}}$ but, the answer is given as $\ a =\ \dfrac {g}{1+\dfrac {m_{1}}{4}\left( \dfrac {1}{m_{2}}+\dfrac {1}{m_{3}}\right) }$. So, why I am wrong here? P.S. How could I solve it in a few steps because the original solution I have is quite long.