In the Van der Waals equation,

$$\left(p+\frac{a'}{v^2}\right)(v-b')= kT$$

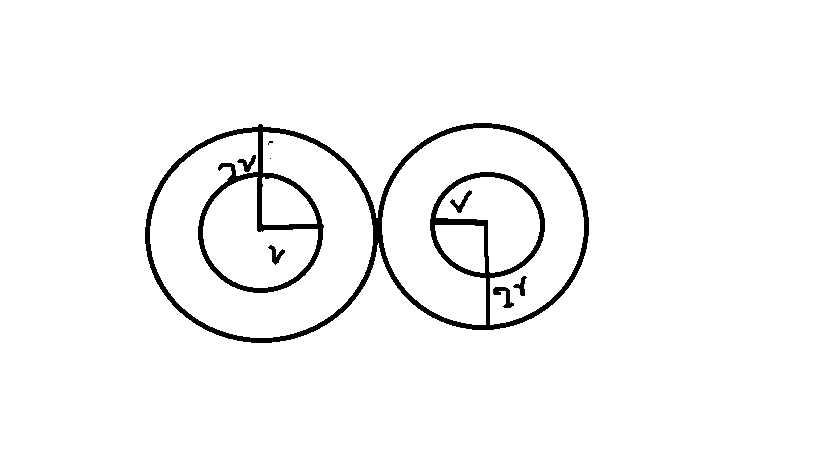
The excluded volume b is not just equal to the volume occupied by the solid, finite-sized particles, but actually four times that volume. To see this, we must realize that a particle is surrounded by a sphere of radius 2r (two times the original radius) that is forbidden for the centers of the other particles. If the distance between two particle centers were to be smaller than 2r, it would mean that the two particles penetrate each other, which, by definition, hard spheres are unable to do.

I am not getting what is the issue with this? Atoms are still not overlapping.

We could simply replace the $b=\textrm{Volume of each sphere}\cdot\textrm{No.of atoms}$

Is my visualisation incorrect?