10
$\begingroup$

Here's what's bugging me for quite a long time. Imagine the every day situation, that you are standing in a bus with your back on wall having only limited space on the floor and no handle to hold. You are quite ok for acceleration effects in 3 of 4 directions. You will not fall behind, the wall is there. Right and left direction is relatively easily handled by a wide stance, but you are troubled about falling forward. Now how would you stand to be able to withstand the acceleration effects the most effectively?

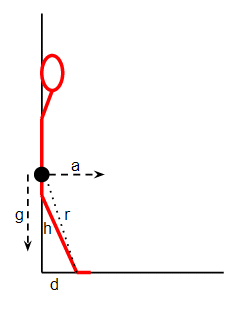
As it is probably a bit too vague (and maybe not completely understandable), let me elaborate it and put some numbers just to demonstrate the situation. Consider a normal human (average height and normal vertical weight distribution, if a notion like this exists) and let's state that the limitation on the floor space is defined as 50 cm from the wall. You cannot put your feet further, because someone is standing there.

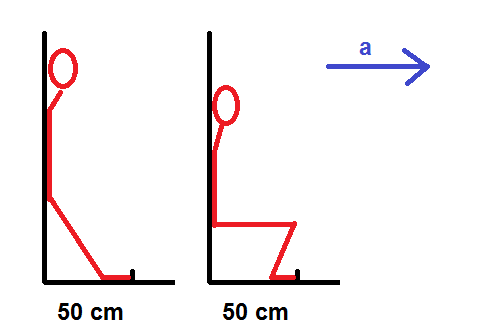
If we skip all exotic and unnatural stances, we are basically left with two extremes and a continuum between them. One extreme is that you are standing with your legs straight, second extreme is that you squat (let's consider here you will not go below the point, when your legs are in parallel with the floor) (and let's ignore how inconvenient this stance is).

My physical imagination is too weak to at least guess, which of these stances is more stable, I just don't suspect seeing optimum somewhere between (but not sure about that either). I also don't have any idea, how it should be calculated and if it's something rather trivial, or something very hard.

Here's a picture of what I was speaking about. Figure on the left is what I eventually do in the bus, figure on the right is what comes intuitively to me as better way (center of mass is lower and maybe the angles are more favorable). So.. is it better to bend the knees (even a bit)?

two extreme stances

Improve this question
$\endgroup$
1
  • $\begingroup$ Ask any surfer or skateboarder. The only diff in riding a bus is that the majority of the time the acceleration vector is along the axis of motion. $\endgroup$
    – Carl Witthoft
    Commented Jan 31, 2014 at 12:45

1 Answer 1

Reset to default
10
$\begingroup$

Let's draw in a few distances and forces:

Bus

The black dot is your centre of mass (normally just below the navel I think). $a$ is the deceleration of the bus and $g$ is the acceleration due to gravity.

If your feet don't skid, the question is whether you will rotate about the point where your feet touch the floor. To answer this we need to calculate the net moment about your feet. If this is negative it will be trying to rotate you anticlockwise so you will stay pinned to the wall. If the moment is positive you will rotate clockwise and fall over.

The moment is the force times the perpendicular distance to the pivot, so the net moment is:

$$ T = -gd + ah $$

And therefore the condition for you not falling over is:

$$ gd > ah $$

When you crouch you reduce the value of $h$, so you reduce $ah$ and this makes you less likely to fall over. There is a slight subtlety to this as bending your legs will change the position of your centre of gravity (it will move up), but overall bending your legs is a good thing (it will also do wonders for your thigh muscles :-).

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks for very clear answer! It all seems pretty straightforward. But as you mentioned, that bending the legs moves up the center of gravity, doesn't it also move forward? It might be negligible effect (have no idea how to incorporate it to the inequality), but if I imagine shortening $d$ to zero (and ignoring length of the feet), then straight position is at least stable when $a = 0$, but any bending itself will cause falling over. Or does this have effect only when center of mass moves beyond $d$? $\endgroup$
    – Erlik
    Commented Jan 31, 2014 at 10:08
  • $\begingroup$ Good point, squatting will move your centre of mass forward because the centres of masses of your thighs and calves will move away from the wall. You'd have to assume some sizes and weights for the various bits of the body then you could calculate exactly how $h$ and $d$ change as you squat. I'm afraid I feel little enthusiam for this - it's straightforward but tedious, and my guess is that the centre of mass doesn't change that much. $\endgroup$
    – John Rennie
    Commented Jan 31, 2014 at 10:16

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.