Can anybody please explain what is the difference between equilibrium state and steady state, as quoted by book by Degroot and Mazur. Also, does violation of Principle of Detailed Balance means the system will not reach equilibrium?
$\begingroup$
$\endgroup$
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
The meaning of the word equilibrium depends on its context; it occurs in, inter alia, biology, chemistry, economics, and physics.
In physics, a good definition is that interaction rates are time symmetric - a process occurs just as fast forwards as backwards. As a consequence, the system's macroscopic properties do not change with time.
A steady state is a state with properties that do not change with time, but it isn't necessarily time symmetric.
$\begingroup$
$\endgroup$
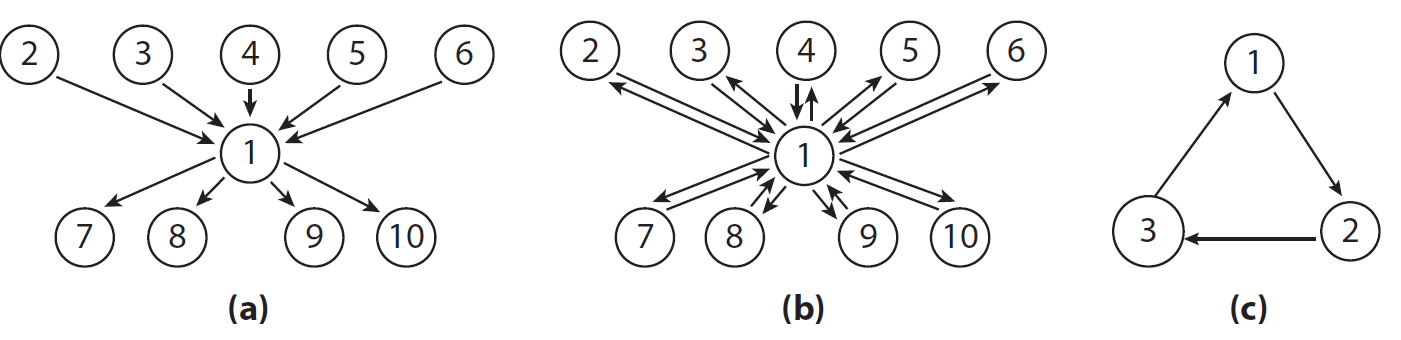
The difference between steady state, equilibrium, and nonequilibrium can be understood using the diagrams given by D.Chowdhary et. al in the book Stochastic Transport in Complex Systems.
a) Steady State: The total probability current into 1 is exactly balanced by total probability current out of the site 1. Hence, rate of proabability current at 1 is 0. Or mathematically it means that $P_C$ is independent of time: $$\sum_{C'}W_{CC'}P_{C'} = \sum_{C}W_{C'C}P_C $$ b) Detailed Balance or Equilibrium: The existence of equilibrium demands much more stronger condition: $$W_{CC'}P_{C'} = W_{C'C}P_C$$ for all configurations $C$ and $C'$. The net probability current must be balanced in the forward and reverse direction by the members of each pair of configuration. c) Nonequilibrium steady states: When detailed balance is violated, then the condition of pairwise-balance holds which mainly means that $$W_{CC'}P_{C'} = W_{C''C}P_C$$ It implies that for every configuration $C'$ which can be reached directly from C a unique configuration $C''$exists. In fig (c), you can see that probability current from $1\rightarrow 2$ is exactly balanced by $2 \rightarrow$ 3, and similarly current from $2 \rightarrow 3$ is balanced by $3 \rightarrow 1$. At each site, the rate of change of probability current is zero.
In particular, the difference between steady state and equilibrium can be seen in this experiment on MIT website:Difference between steady state and Equilibrium
$\begingroup$
$\endgroup$
When looking at gas dynamics in particular, it is also possible that the steady state is not in equilibrium because the flow has become frozen. In other words, the energy is locked in each molecule and unable to transfer to others for various reasons, usually because the time between collisions is too large.
This is in obvious contrast to an equilibrium state.
An example of the difference between equilibrium and frozen flow for a rocket nozzle shows that the two are bounding cases for the real situation. The flow is in equilibrium in the subsonic region, non-equilibrium through the throat and eventually becomes frozen as the flow accelerates considerably through the nozzle.
To answer the second part, a violation of detailed balance does not mean that it will not reach equilibrium, just that it is not yet in equilibrium. Detailed balance is valid only at equilibrium -- if the system is not yet in equilibrium, it is not possible for the processes to be reversible.