1
$\begingroup$

I was wondering about this today, mainly for baking a cylindrical cake.

Say you have a solid cylinder which is at temperature T0 inside.

Say you then move the cylinder to a warm location (like an oven) which is at temperature T1.

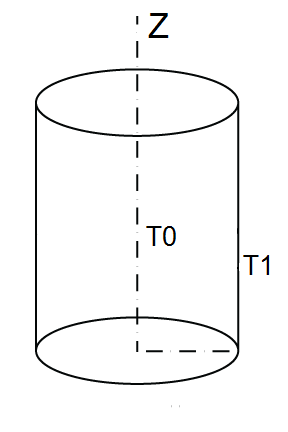
Solid Cylinder

Is it possible to achieve a rate equation for dT/dt=f(t) where T is the temperature exactly in the centre of the cylinder and t is time?

And therefore work out the time it would take this location to reach a temperature T2 somewhere between T0 and T1.

To keep it as general as possible I would prefer to assume the cylinder is height h and radius r.

I've been trying to use the thermal diffusivity equation:

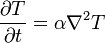
Diffusivity Eqn

But my working gets messy quickly, I figured as this is quite a simple shape it should be less complicated than some similar examples I've found.

EDIT:

So using my notes from a differential equations course a few years back I managed to do the following:

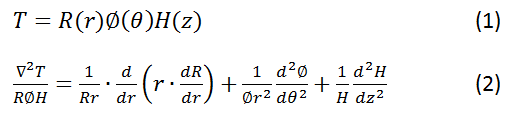
Working

But I'm stuck here..

Improve this question
$\endgroup$
4
  • $\begingroup$ The top and bottom also has heat flow in, so the solution would only be rotational invariant, i.e. $T(s,\theta,\phi)=T(s,\phi)$ $\endgroup$
    – unsym
    Commented Dec 17, 2013 at 22:56
  • $\begingroup$ You could probably assume that the majority of the heat flow warming the centre (which I'm interested in) will come from the top and bottom as height<<radius. $\endgroup$
    – Edd
    Commented Dec 17, 2013 at 23:00
  • $\begingroup$ Yes. You can solve the heat equation "analytically" for this geometry. Once you have the solution you can find the derivative in time at any point. Using separation of variables with $T=A(r)B(\theta)C(Z)$, since it is cylindrical geometry, you will end up with Bessel functions in $r$ and Fourier series in $\theta$ and $Z$. $\endgroup$
    – SimpleLikeAnEgg
    Commented Dec 17, 2013 at 23:01
  • $\begingroup$ @SimpleLikeAnEgg That rings a bell, I'm going to have to check back through my dusty second year physics notes. $\endgroup$
    – Edd
    Commented Dec 17, 2013 at 23:02

1 Answer 1

Reset to default
2
$\begingroup$

This is a simple (yet not simple to solve!) transient heat transfer problem using cylindrical coordinates. There are solutions available in handbooks if you do not want to go through the math and Bessel function solutions arise. Its been a while since I have done this myself.

The boundary condition is the tricky part. If you use a constant temperature for the surface, i.e, Dirichlet BC, the problem is considerably easier but would not represent the reality of the situation. In reality, its is convection and radiation that is responsible for the heat transfer in the exposed areas and conduction where the cylinder rest (i would assume the base from the way you have drawn it). If you are using convective boundary condition with known $h$, then you can use these charts and how to use them provided by Cengel (also attached herein).

With radiative heat transfer, its more complicated. You would need to use Stephan-Boltzmann law and somehow estimate the emmisivity of your surface, as well as the view factor. However, you could in come up with an conservative heat transfer coefficient, $h$, that could allow include the heat radiation part as well.

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.