0
$\begingroup$

I did a lot of browsing and searching, to find nothing regarding this.

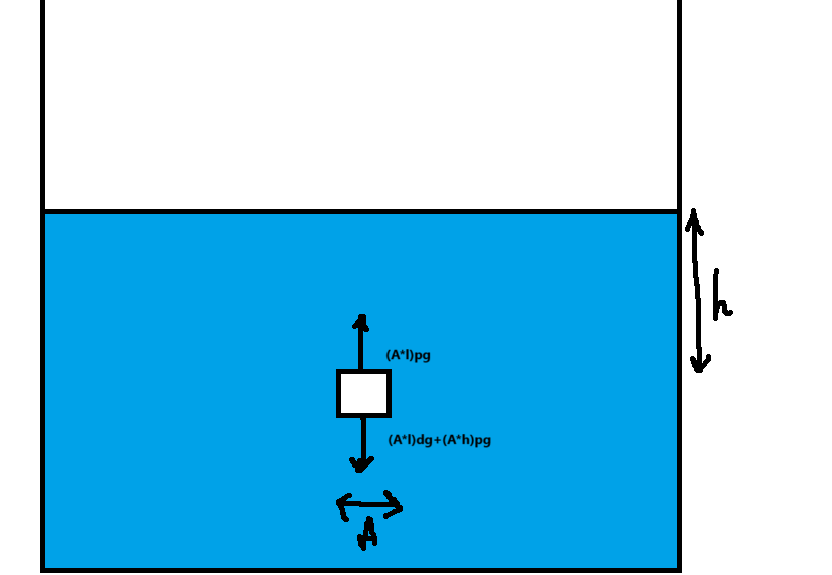
Assume a body submerged in water (or any liquid for that matter). When we draw the FBD, we show the buoyant force, the weight of the object. These two forces simulate and determine whether the object sinks or floats (neglecting viscosity and other non-ideal conditions).

My question is, why don't we also include the weight of water with these two forces?

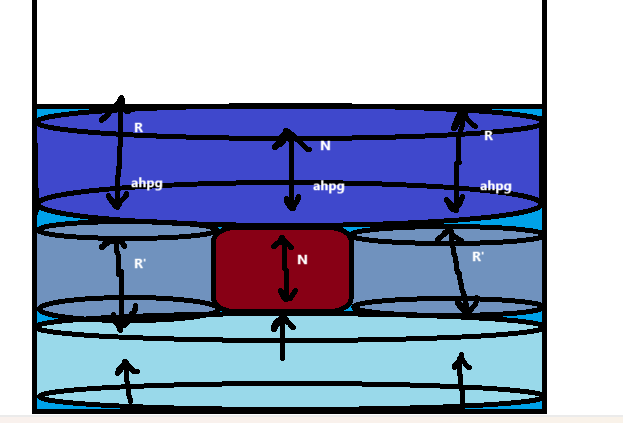
enter image description here

Another question i had was how do your find the force on an object(say cylinder) at a depth $h$ without using any imaginary cylinders (using pure mathematics and fbd's, please do not answer saying that F= Area*Pressure at that point = $A*hpg$). My approach was something like this

enter image description here

As you may guess it gets pretty messy and doesnt really work.

Improve this question
$\endgroup$
1

3 Answers 3

Reset to default
4
$\begingroup$

The "weight" of the water is covered for in the buoyancy force, so to say.

Water above the object pushes down on it with a force equal to its gravitational force (its weight). But other water tries to "get below" it, pushing it up. The sum of these two effects is what we know as buoyancy.

Improve this answer
$\endgroup$
2
$\begingroup$

Not to be too pedantic about it, but: the weight of the water is a force acting on the water. In other words, it would be incorrect to view "the weight of the water" as force as acting on a buoyant object.

There are of course contact forces between the water and the buoyant object, which are the two forces you've drawn in your diagram. (There are also forces on the sides of the object, but those cancel out.) In fact, the weight of the water column above your object is exactly equal to the contact force exerted on the top face of your object, since $$ W = m g = A h \rho g $$ with $h$ being the height of the water column above. It's as though the water column was "sitting on top" of the object and exerting a contact force on it, just like a stack of objects on a table.

(The upwards force from below is a bit harder to conceptualize this way, but it must be there because the adjacent water columns have to support themselves, and the pressure must be the same at a given depth regardless of what's above or below it.)

Improve this answer
$\endgroup$
1
  • $\begingroup$ Re, "Not to be too pedantic..." IMO, that's hard to do when you're trying to teach how something actually works. My dictionary defines "pedantic" as, "Overly concerned with minute details or formalisms." But, when the details and the formalisms are the explanation, then it's kind of hard to over emphasize them. You're only being pedantic when you refuse to take the shortcut that everybody else in the room always takes, and it's clear that everybody else in the room understands what is being cut short. $\endgroup$
    – Solomon Slow
    Commented 22 hours ago
0
$\begingroup$

When you're drawing the free body diagram, you're looking for forces that act on this body. Does the weight of water act on the body? It certainly acts on water, but does it act on the body?

If you're careful, you can see that the buoyant force the body experiences derives from the weight of water (see Steeven's answer). On an intuitive level however, you can also think of it as the weight of water acting on the water, but not on the body.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thanks for the answer. Apologies for editing the question, could you also answer the other question. Thanks $\endgroup$
    – Star Gazer
    Commented 23 hours ago
  • $\begingroup$ @StarGazer how do your find the force on an object? You integrate, using calculus, It's how we treat varying numbers (e.g. kinematics with non-constant acceleration). If you've not encountered calculus before, you should within a few years. $\endgroup$
    – Allure
    Commented 23 hours ago
  • $\begingroup$ Well thanks but i was looking for the mathematics however complex it may be. As we know the net force on the body is simply Buoyant Force - Weight, How do i derive it using calc? $\endgroup$
    – Star Gazer
    Commented 22 hours ago
  • 1
    $\begingroup$ A force balance on a motionless infinitesimal cube with side length $dx$ shows that the weight $\rho g (dx)^3$ must be offset by a pressure difference $dP$ acting on the top and bottom areas $(dx)^2$. So $\frac{dP}{dx}=\rho g$. Integrate this from the surface with gauge pressure $0$ to get gauge pressure $P(h)=\rho g h$ at depth $h$. $\endgroup$
    – Chemomechanics
    Commented 21 hours ago

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.