After reading this chapter of Feynman Lectures oh Physics: https://www.feynmanlectures.caltech.edu/I_20.html, inspired by Feynman's method in showing that torque is a vector, I decided to show that angular velocity is a vector using his method.
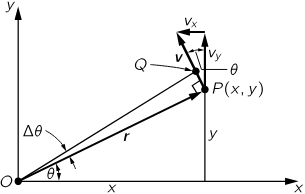
The above image shows the displacement of a particle in the $xy-$plane when it rotates about the origin $O$ from $P$ to $Q$. The image comes from https://www.feynmanlectures.caltech.edu/I_18.html.
From the picture, one can deduce that in a small time interval $\Delta t$, the displacement $PQ$ is: \begin{equation} r\Delta\theta = -\Delta x\sin{\theta} + \Delta y \cos{\theta} \end{equation} where $x$ and $y$ are respectively the $x-$ and $y-$positions of the particle, $r = \sqrt{x^2 + y^2}$ is the distance of the particle from the origin and $\theta$ is the angle from the positive $x-$axis to the line $OP$. If there is a '$\Delta$' in front of a quantity, that means a small(infinitesimal) change in that quantity. Divide both sides by $\Delta t$ and rearrange gives: \begin{equation} \frac{\Delta\theta}{\Delta t} = -\frac{\Delta x}{\Delta t} \frac{\sin{\theta}}{r} + \frac{\Delta y}{\Delta t} \frac{\cos{\theta}}{r} \end{equation} Because $\sin{\theta} = y/r$ and $\cos{\theta} = x/r$ and $r = \sqrt{x^2 + y^2}$: \begin{equation} \frac{\Delta\theta}{\Delta t} = -\frac{\Delta x}{\Delta t} \frac{y}{x^2 + y^2} + \frac{\Delta y}{\Delta t} \frac{x}{x^2 + y^2} \end{equation} In the limit as $\Delta t \to 0$, the right-hand side of the above equation gives the angular velocity in the $xy-$plane. Denote this quantity as $\omega_{xy}$ and note that $\lim_{\Delta t \to 0} \frac{\Delta x}{\Delta t} = \frac{dx}{dt} = v_x$, which is the velocity of the particle in $x-$direction, and $\lim_{\Delta t \to 0} \frac{\Delta y}{\Delta t} = \frac{dy}{dt} = v_y$, which is the velocity of the particle in $y-$direction: \begin{equation} \omega_{xy} = -v_x \frac{y}{x^2 + y^2} + v_y \frac{x}{x^2 + y^2} \end{equation} Similarly, the angular velocity in the $yz-$plane, $\omega_{yz}$, and the angular velocity in the $zx-$plane, $\omega_{zx}$, are: \begin{equation} \omega_{yz} = -v_y \frac{z}{y^2 + z^2} + v_z \frac{y}{y^2 + z^2}\\ \omega_{zx} = -v_z \frac{x}{z^2 + x^2} + v_x \frac{z}{z^2 + x^2} \end{equation}
So far so good. Now, in Feynman's example of showing torque being a vector, he introduced a new set of axes, which is generated by rotate the old axes about the $z-$axis by an angle $\phi$ (In Feynman's original example, he used the letter $\theta$ instead of $\phi$. It doesn't matter which letter I use. Here I use $\phi$ to avoid confusion between symbols.) Thus the new set of axes $x'$, $y'$ and $z'$, which tells the position of the same particle in the new axes, can be expressed in terms of the old axes as: \begin{equation} x' = x \cos{\phi} + y \sin{\phi}\\ y' = y \cos{\phi} - x \sin{\phi}\\ z' = z \end{equation} The similar relation holds for $v_{x}'$, $v_{y}'$ and $v_{z}'$ in the new set of axes too because velocity is a vector. Therefore: \begin{equation} v_{x}' = v_{x} \cos{\phi} + v_{y} \sin{\phi}\\ v_{y}' = v_{y} \cos{\phi} - v_{x} \sin{\phi}\\ v_{z}' = v_{z} \end{equation}
According to Feynman, if I can prove that the new angular velocities in $x'y'-$, $y'z'-$ and $z'x'-$planes have exactly the same relationship with the old angular velocities as that of $x$, $y$ and $z$ with $x'$, $y'$ and $z'$ or of $v_{x}$, $v_{y}$ and $v_{z}$ with $v_{x}'$, $v_{y}'$ and $v_{z}'$, then problem solved-The angular velocity is indeed a vector! Now let's try it!
First consider new angular velocity in the $x'y'-$plane, $\omega_{x'y'}$: \begin{equation} \omega_{x'y'} = -v_{x}' \frac{y'}{x'^2 + y'^2} + v_{y}' \frac{x'}{x'^2 + y'^2}\\ = -(v_{x} \cos{\phi} + v_{y} \sin{\phi}) \frac{y \cos{\phi} - x \sin{\phi}}{(x \cos{\phi} + y \sin{\phi})^2 + (y \cos{\phi} - x \sin{\phi})^2} + (v_{y} \cos{\phi} - v_{x} \sin{\phi}) \frac{x \cos{\phi} + y \sin{\phi}}{(x \cos{\phi} + y \sin{\phi})^2 + (y \cos{\phi} - x \sin{\phi})^2}\\ = \frac{-v_x y \cos^2{\phi} + v_x x \sin{\phi} \cos{\phi} - v_y y \sin{\phi} \cos{\phi} + v_y x \sin^2{\phi} + v_y x \cos^2{\phi} + v_y y \sin{\phi} \cos{\phi} - v_x x \sin{\phi} \cos{\phi} - v_x y \sin^2{\phi}}{x^2 \cos^2{\phi} + y^2 \sin^2{\phi} + 2xy \sin{\phi} \cos{\phi} + y^2 \cos^2{\phi} + x^2 \sin^2{\phi} - 2xy \sin{\phi} \cos{\phi}}\\ =\frac{-v_x y + v_y x}{x^2 + y^2}\\ =\omega_{xy} \end{equation} where the identity $\sin^2{\phi} + \cos^2{\phi} = 1$ is used. Now this $\omega_{x'y'} = \omega_{xy}$ corresponds to the relationship between the $z-$components of the new and old angular velocity vectors. Now we try to do it for $\omega_{y'z'}$, which corresponds to the $x-$component and I am expecting $\omega_{y'z'} = \omega_{yz} \cos{\phi} + \omega_{zx} \sin{\phi}$: \begin{equation} \omega_{y'z'} = -v_{y}' \frac{z'}{y'^2 + z'^2} + v_{z}' \frac{y'}{y'^2 + z'^2}\\ = -(v_{y} \cos{\phi} - v_{x} \sin{\phi}) \frac{z}{(y \cos{\phi} - x \sin{\phi})^2 + z^2} + v_z \frac{y \cos{\phi} - x \sin{\phi}}{(y \cos{\phi} - x \sin{\phi})^2 + z^2}\\ = \frac{-v_y z \cos{\phi} + v_x z \sin{\phi} + v_z y \cos{\phi} - v_z x \sin{\phi}}{(y \cos{\phi} - x \sin{\phi})^2 + z^2}\\ = \frac{-v_y z + v_z y}{(y \cos{\phi} - x \sin{\phi})^2 + z^2} \cos{\phi} + \frac{-v_z x + v_x z}{(y \cos{\phi} - x \sin{\phi})^2 + z^2} \sin{\phi} \end{equation} which is not the form I am expecting because $(y \cos{\phi} - x \sin{\phi})^2 + z^2 = y'^2 + z^2 \neq y^2 + z^2$. Similar unexpected result would occur for $\omega_{z'x'}$ (I am expecting $\omega_{z'x'} = \omega_{zx} \cos{\phi} - \omega_{yz} \sin{\phi}$, but this doesn't happen.). Now, this is my question: what has gone wrong in my argument?