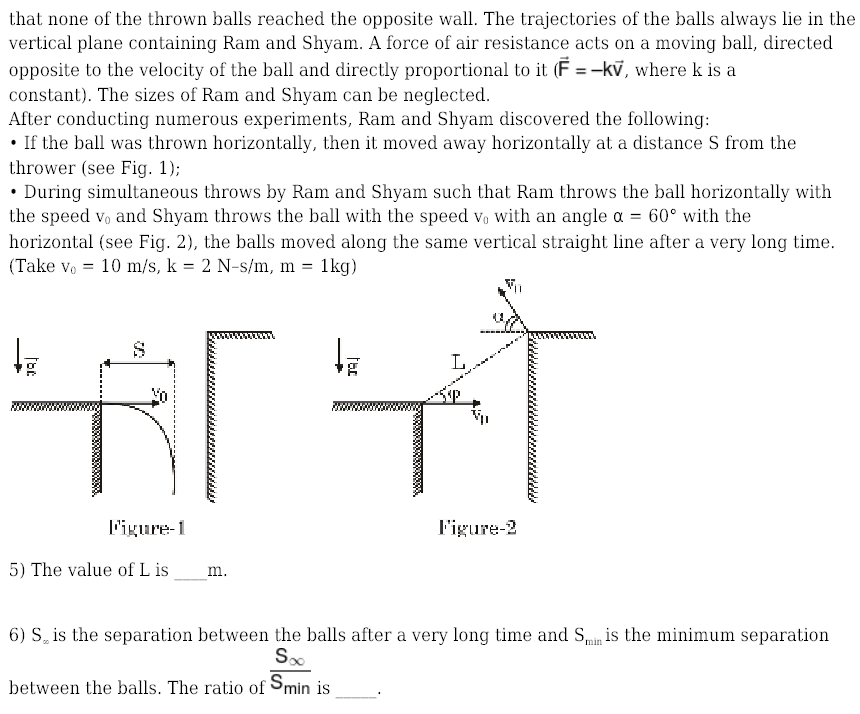
The following was a question posed in a recent test series:
What would the intended solution be to such a question? Are there any defining features that could be used to simplify the problem or is manual calculation necessary for Q6?
My attempt at a solution:
For the left projectile:
$$F_x = -kv_x$$
$$mdv_x/dt = -kv_x$$
$$mv_x = v_0e^{-t}$$
$$x = v_0(1-e^{-t})/m$$
And creating a similar setup for $v_y$
$$F_y = mg - kv_y$$
$$mdv_y/dt = mg - kv_y$$
$$v_y = mg(1-e^{-kt/m})/k$$
$$y = mg/k \cdot (t + m/k \cdot (1 - e^{-kt/m}))$$
And do the same thing for the other ball in order to get $x_1(t)$, $x_2(t)$, $y_1(t)$, $y_2(t)$ as functions of time and operate on them in order to get $S$ and $S_{min}$.
However, it is unlikely that this is the intended solution, as we are expected to solve these problems in about 6 minutes - 3 minutes per subproblem. Is there an easier method? Possibly something like $dL/dt = 0$ for q6? I have attempted to find other methods and not had any success, only managing to make the solution longer. Any help is appreciated.
This question is from the ALLEN score test series for JEE advanced preperation physics section. Answers are 8.65m and 1.15m.