Is the angular diameter distance to the surface of last scattering the product of a circular argument?

Question

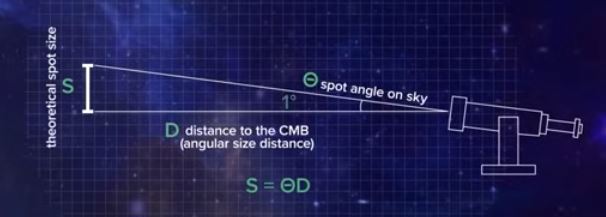
To determine that the geometry of the universe is flat, the physical size of sound waves $S$ at the surface of last scattering was found to match their theoretical size of 431,700 light-years (0.13236 megaparsecs). To perform this calculation both the angular size $\theta$ of the sound waves and their angular diameter distance $D$ was used, which can be demonstrated as follows:

$S = (\theta*D$) = $0.010414$ radians (0.5967 degrees) * $12.71 Mpc$ = $0.13236$ Mpc $(431,700$ $light-years)$

To make this calculation you need to know the angular diameter distance $D$, which itself is obtained from the following: $D=\frac{1}{1+z}\int_0^z \frac{cdz'}{H(z')}$.

However, solving this integral requires understanding the universe's expansion history, which depends on its shape and therefore its matter and energy composition—including dark energy—since the universe has been determined to have a flat geometry.

My question is this: If the angular diameter distance is required to calculate the physical size of the sound waves at the surface of last scattering, which is then used to determine the geometry of the universe (and consequently the existence of dark energy), but determining the angular diameter distance itself depends on knowing the universe's geometry (including its matter and energy composition, such as dark energy), doesn’t this create a circular argument?

Answer 1

If the physical size of the sound horizon at the surface of last scattering is fixed (e.g., Jungman et al. 1995), then the angular scale associated with this depends on the geometry of the universe.

In other words, using your definitions, you measure $\theta$, and $S$ is fixed, so you are effectively measuring $D = S/\theta$. Getting from there to a unique set of cosmological parameters would be difficult - you can't separate all the relevant parameters with one piece of information, although how flat the universe is (i.e. how close $\Omega_M + \Lambda$ is to 1) does have the dominant effect, along with $H_0$

But I think what you are getting at, is doesn't $S$ also depend on the cosmological parameters? Well, yes it does, but not in the same way that $D$ varies with those parameters. This is what avoids the circularity you suggest. A good discussion can be found in section 5 of Reid et al. (2002).

Fortunately, that isn't how the cosmogical parameters are determined at all (other than conceptually). They are found by modelling the full CMB angular power spectrum and fitting that to the data. i.e. Choose your cosmological parameters; generate a model angular power spectrum; does it match the observed CMB power spectrum, if not then iterate the parameters until it does. The additional information that comes from the height and shape of the peaks, not just the first peak, provides enough information to break most of the parameter degeneracies. The sensitivity of the angular power spectrum to those parameters is also discussed in Jungman et al (1995).