7
$\begingroup$

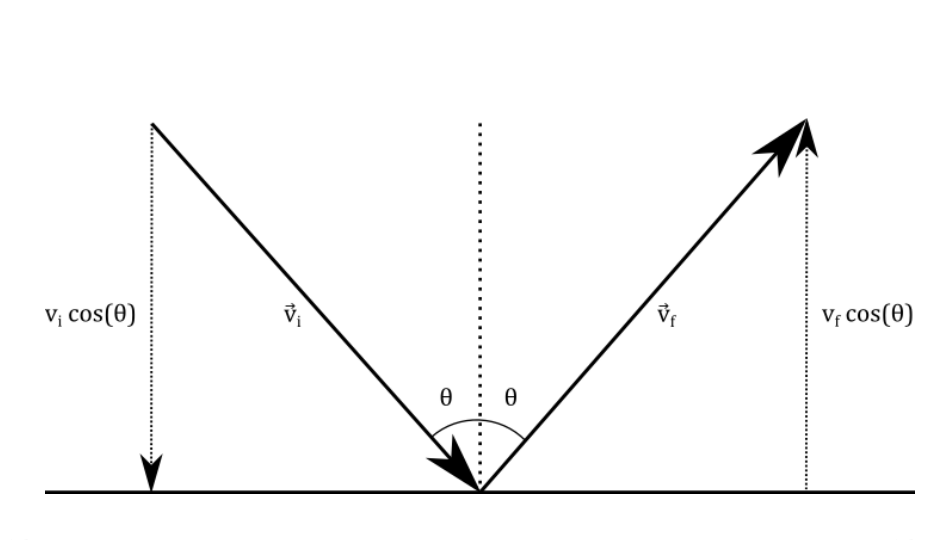
In this figure (p.21, fig.3.5)

enter image description here

a ball with velocity $v_i$ bounces elastically on a surface with an incident angle $\theta$ with respect to the normal. Due to the conservation of kinetic energy the magnitude of the outgoing velocity $v_f$ is the same as $v_i$.

I don’t understand how the speed of the ball does not change after bouncing. If this were the case a billard ball will continue bouncing forever without losing its speed. Can you explain?

Improve this question
$\endgroup$
8
  • 4
    $\begingroup$ here as a toy model, it assumes the collision is ideally elastic $\endgroup$
    – Ma Ye
    Commented Nov 12 at 7:31
  • 9
    $\begingroup$ The single word "elastically" in the problem definition is doing some heavy lifting, indicating that kinetic energy is perfectly conserved before and after the collision. Everyday intuition about billiard balls slowing to a stop doesn't really apply here, as we've defined the system with ideal, unrealistic properties. $\endgroup$
    – Nuclear Hoagie
    Commented Nov 12 at 16:35
  • 2
    $\begingroup$ @zeynel A perpetual motion machine isn't simply something that moves forever, it's a machine that can run forever while producing useful work - it's a limitless source of usable energy. There is nothing that prohibits perpetual motion without doing work - an object floating through deep space will indeed continue on the same trajectory with the same speed forever. No energy is extracted during a series of elastic collisions - even though it bounces forever, it's not a perpetual motion machine under the usual definition. $\endgroup$
    – Nuclear Hoagie
    Commented Nov 13 at 14:45
  • 1
    $\begingroup$ @NuclearHoagie the perpetual motion machine you describe is known as the first kind. The third kind only has to maintain motion forever $\endgroup$
    – Chris H
    Commented Nov 13 at 15:38
  • 1
    $\begingroup$ @zeynel this bounce would necessarily be silent. A real billiard ball's bounce isn't (that's where some of the energy goes). So it's not a model of real collisions. "All models are wrong, some are useful" applies to physics as well as to statistics, from where the quote comes. $\endgroup$
    – Chris H
    Commented Nov 13 at 15:41

5 Answers 5

Reset to default
16
$\begingroup$

In an ideal scenario the billard ball would indeed continue rolling and bouncing forever and never stop.

But nothing is ideal in our world. There are two main things to consider:

  • Rolling resistance: When rolling on a billard table typically made of fabric, the surface deforms a bit and causes an uneven surface with a non-pointlike contact to the ball, thus causing non-ideal normal forces that would work agains the rolling motion.

  • Impact energy loss: This is what your question is refering to. In perfectly elastic collisions kinetic energy must indeed be conserved. So if one object is stationary - such as a wall - then all kinetic energy must be re-absorbed by the ball, giving it the same exit speed as entry speed. But in reality, the wall is covered with a non-ideal material that might undergo deformation or at least delayed elastic spring-back, might experience heating effects at impact and so on. All these factors would cause energy loss resulting in not the same kinetic energy amount after the impact as before and thus a small reduction in speed.

So, in practice nothing will ever move, nor bounce, forever. But physics principles are often stated for ideal circumstances so that we are able to understand them before applying all the real-world non-ideal troubles to the scenario.

Improve this answer
$\endgroup$
5
  • $\begingroup$ The author says in the caption to the figure that the velocity remains constant because of conservation of kinetic energy. But in the text he writes, referring to the same figure, that velocity stays the same due to conservation of momentum. Which one is correct? $\endgroup$
    – zeynel
    Commented Nov 12 at 9:14
  • 3
    $\begingroup$ @zeynel Both. The momentum conservation law applies to all elastic impacts. When one object stays stationary - such as a wall - then a consequence of momentum conservation is kinetic energy conservation. (Also note, by the way, that it is not the velocity that remains constant, it is the speed.) $\endgroup$
    – Steeven
    Commented Nov 12 at 9:26
  • 5
    $\begingroup$ The momentum conservation law applies to all impacts, whether elastic or non-elastic. KE conservation requires elastic impacts. $\endgroup$
    – Neil_UK
    Commented Nov 12 at 19:48
  • $\begingroup$ @Neil_UK Ahh, right, I miswrote in my comment. Thanks for the correction. $\endgroup$
    – Steeven
    Commented Nov 13 at 11:08
  • $\begingroup$ @Steeven pedantic correction to my correction - 'as long as the system is drawn to include all colliding bodies'. Both KE and momentum can be 'lost' to a wall outside the system. $\endgroup$
    – Neil_UK
    Commented Nov 13 at 11:18
7
$\begingroup$

The speed (and energy) of the ball after the collision depends on the coefficient of restitution between the ball and the surface. In the ideal case that is illustrated the coefficient of restitution is assumed to be $1$, so this is a perfectly elastic collision and the ball has the same speed and energy after the collision as before.

However, in real life collisions between balls and surfaces are never perfectly elastic. The real life coefficient of restitution will be less than $1$ and so the ball's speed and energy after the collision will be less than they were before.

(Note that I am assuming that the surface is stationary in all cases)

Improve this answer
$\endgroup$
5
  • $\begingroup$ (+1) If the collision is between 2 balls (instead of ball vs. surface), does the coefficient of restitution still provide some degree of freedom, or are the final post-collision velocities completely determined in this case? $\endgroup$
    – James
    Commented Nov 12 at 11:00
  • 3
    $\begingroup$ @James If you know the coefficient of restitution and the masses of the balls and their speeds before the collision then their speeds after the collision are completely determined by conservation of momentum. $\endgroup$
    – gandalf61
    Commented Nov 12 at 11:30
  • $\begingroup$ thank you. I was thinking about collisions in particle physics (e.g. Compton scattering), it seems that nothing like coefficient of restitution is used in particle physics collision, and the final velocities are completely determined from conservation of momentum & conservation of energy? $\endgroup$
    – James
    Commented Nov 12 at 21:42
  • $\begingroup$ ... I suppose the assumption of perfectly elastic collision will eliminate the coefficient of restitution degree of freedom entirely? Then the coefficient of restitution is measuring the degree of non-elasticity of a collision? $\endgroup$
    – James
    Commented Nov 12 at 23:26
  • 1
    $\begingroup$ @James Yes. For a perfectly elastic collision you can say that the coefficient of restitution is $1$. Equivalently, you can that energy is conserved in the collision. $\endgroup$
    – gandalf61
    Commented Nov 13 at 9:16
4
$\begingroup$

Many, if not all, Physics laws are stated with reductionism in mind. A principle which states that a complex nature processes can be deconstructed into more fundamental ones. In other words Physicists believes that nature behaves in certain way which is describable by "the sum" of core laws and principles.

This implies that for any physics law $\mathbb X$ we must omit irrelevant features and trivial details for capturing fundamental law or phenomena principle. In this given task core concept is perfectly elastic collisions, which assumes no kinetic energy dissipation.

To understand how billiard game physics works and to model it in a computer, at first you need to understand perfectly elastic collisions and only after that you can proceed introducing kinetic energy dissipations as in inelastic collisions. From simple, to more complex, that's how education goes. Skipping first core topic and jumping to the "realities" will do more harm to the students than more good.

Improve this answer
$\endgroup$
1
$\begingroup$

Note that this is a circular definition: "Perfect elasticity" is the property that no heat is generated by deforming and "un-deforming" the objects, so that all kinetic energy is preserved; that the overall system does not "lose" kinetic energy by transforming it into heat is how we know that the materials are perfectly elastic!

By the way, the model is ideal (i.e., does not describe actual reality) in more ways, some of them fundamental: There is probably some air friction, some minuscule energy is transferred to the planet the wall is attached to (albeit very little since Earth has such a large mass), there is a gravitational field next to other masses, leading to curved trajectories, and a classroom or lab is not an inertial system since it's on multiple superimposing orbits.

Overall, air friction and less-than-perfect elasticity are by far the largest deviations from the ideal here, but rigorous thinking should not neglect the other ones either.

Improve this answer
$\endgroup$
0
$\begingroup$

A very subtle gotcha is also angular momentum, assuming real friction and ball diameter but other ideal setup. This turns linear momentum into angular momentum. This conserves energy, but turns the trajectory from bouncing into rolling.

In contact, the floor pushes left but ball's momentum carry it right. Since these forces are parallel, but in opposite directions and separated by the ball's radius, it gives a torque.

Friction is a catch-all term for many related microscopic processes (static, Coulomb, Stribeck, viscous, etc) but if the ball's surface perfectly locked with the floor, none would apply. All of it's energy would become non-slip rotation/translation, and none would become heat.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.