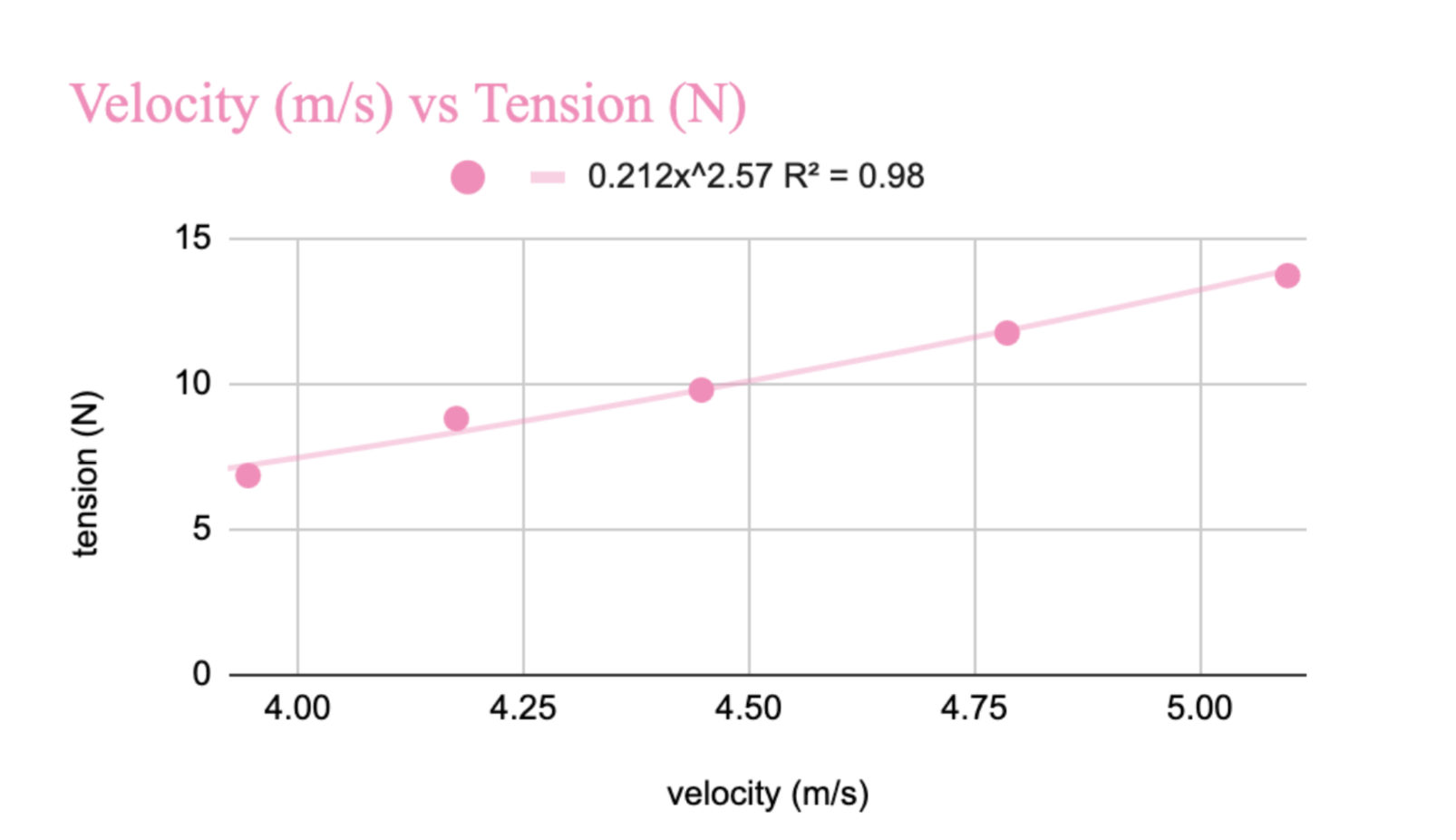
The same goes for a graph of velocity vs the force of tension
For a bob of mass m on the end of a string moving in a circle of radius r, the centripetal force that keeps the bob moving in a circle is provided by the tension in the string. Centripetal force F is is given by $F = M \omega^2 r = M v^2 / r$. This means the relationship between tension and velocity is $F \propto v^2$ and the relationship is indeed quadratic.
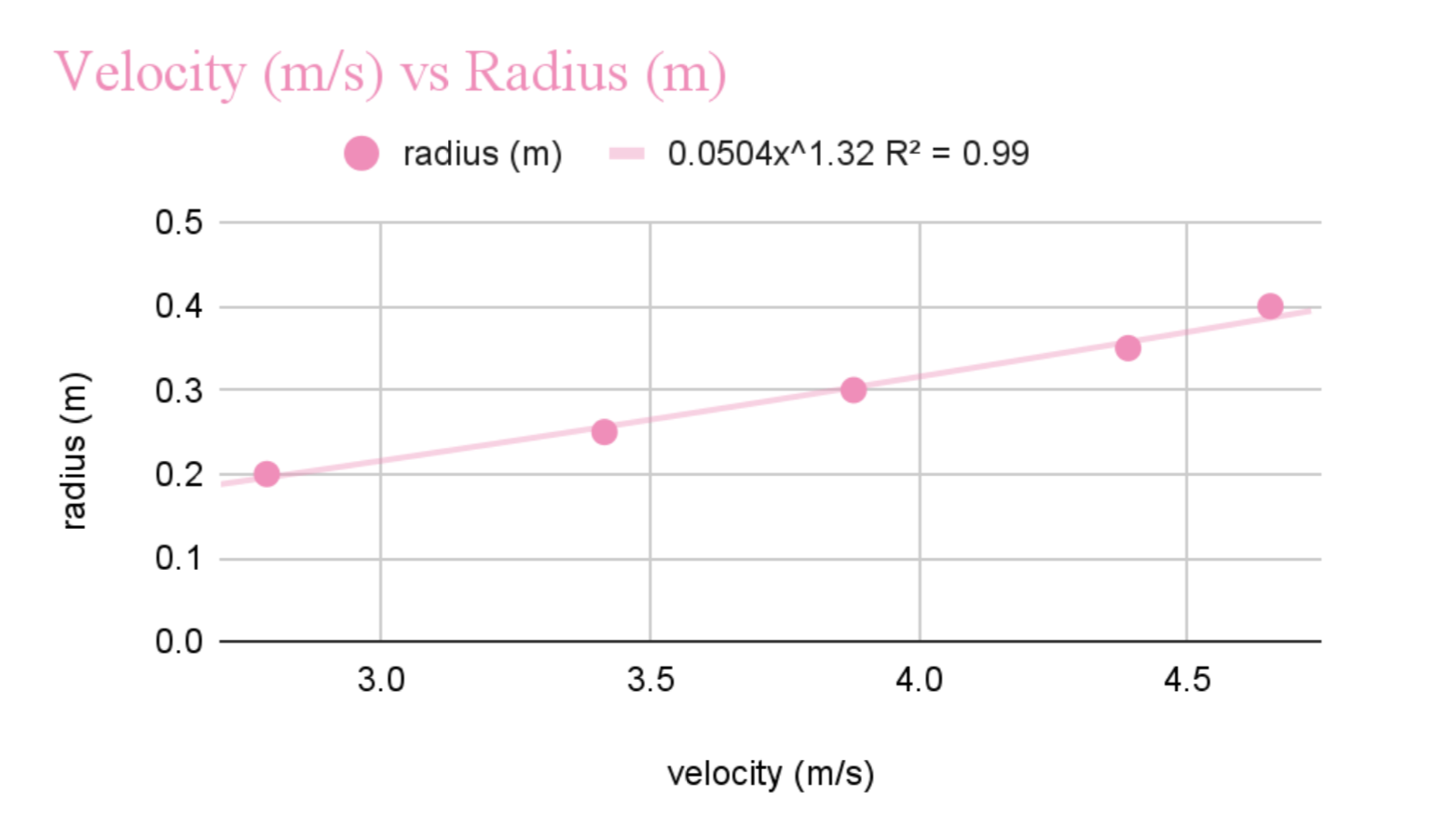
On a graph of velocity vs radius of a bob undergoing uniform circular
motion, there is a quadratic increase.
You have not specified the scenario precisely. I am going to assume the bob is with moving with constant angular momentum and the radius is altered. If we treat the bob as an idealised point particle, the angular momentum L is given by $L = I \omega = m r^2 \omega = m r v$ In this context $v \propto r$ and the graph is linear, not quadratic.
If we assume the string is elastic and the radius increases due to stretching as the tangential velocity increases, then the relationship between the radius and the velocity can be found using Hooke's law ($F = k\Delta X =$), where $\Delta X $ is the extension of the string and k is the stiffness constant of the string. In this case $$R = X + \Delta X = X+ kF = X + kM v^2 / R,$$ where X is the relaxed length of the string. Solving for R gives us $$R = v \sqrt{kM +X^2}+ X/2.$$ This implies that the relationship between radius and velocity is still linear even in the elastic scenario.
Is the radius one because the area of a circle is r^2?
The area of a circle is actually $A = \pi r^2$. If $A = r^2$ this implies $\pi r^2 = r^2$ and $\pi =1$ which is obviously not correct.
Is the radius one because the area of a circle is r^2?
The area of a circle is actually $A = \pi r^2$. If $A = r^2$ this implies $\pi r^2 = r^2$ and $\pi =1$ which is obviously not correct. Even if we use the correct definition for the area of a circle the radius cannot be determined, without additional information.
Above both your graphs are some equations in x and r but you have not told us what the variables are or where the equations come from. If you clearly define all the parameters and equations in your scenario, this will help you find the clarity required to find the solution you are looking for.