Confusion when utilizing complex electric field to do calculations

Question

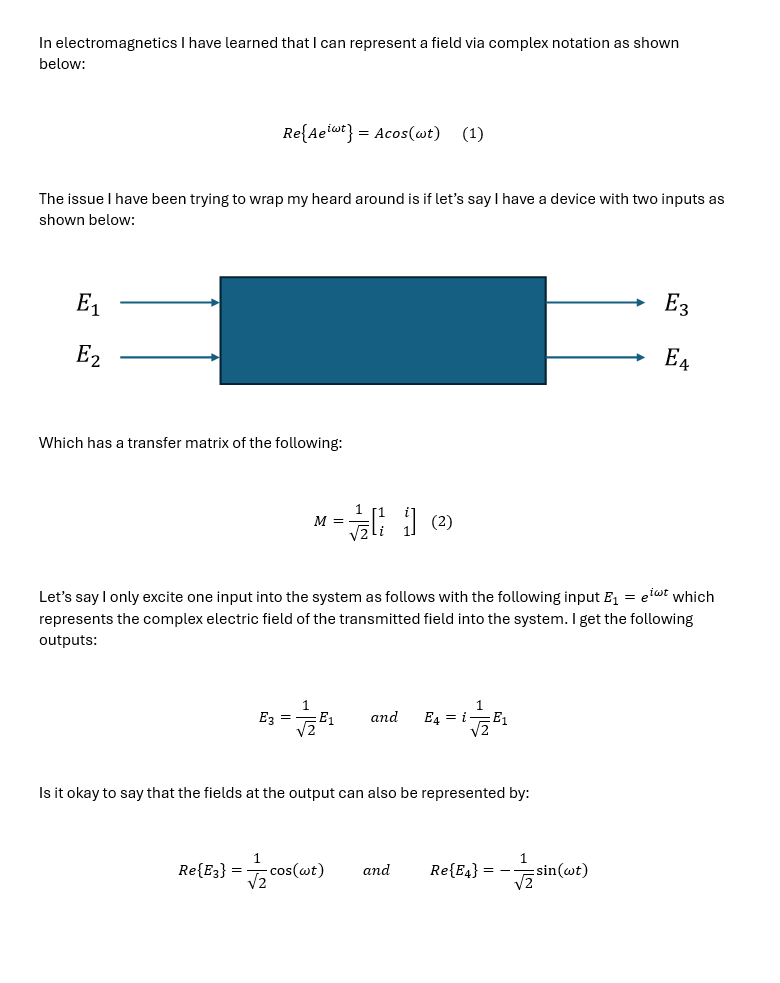
I've been struggling using complex exponentials. From my understanding using complex exponentials can simplify calculations without the use of sinusoidal waves. The thing that has been bothering me is that the system I have posted should display a split in power between the two outputs as well as show a 90 degree phase shift between the two outputs.

From my understanding the two outputs of the system are e^(iwt) and ie^(iwt). In this scenario "i" displays a phase shift in the complex plane, but let's say I wanted to plot the field relationship and physically see the phase shift would I just take the real part of my outputs, resulting in cos(wt) and -sin(wt)?

Answer 1

From my understanding the two outputs of the system are e^(iwt) and ie^(iwt). In this scenario "i" displays a phase shift in the complex plane,

Remember that

$$i = e^{i\frac{\pi}{2}}$$

so

$$ ie^{i\omega t} = e^{i\omega t + \frac{\pi}{2}}$$

which should make it more obvious why multiplying by i is the same as a phase shift of $\pi/2$.

Let's say I wanted to plot the field relationship and physically see the phase shift would I just take the real part of my outputs, resulting in cos(wt) and -sin(wt)?

In general when we talk about a field $E = E_0e^{i\theta}$ it's a shorthand for $E(t) = {\rm Re}\{E_0e^{i\theta}e^{i\omega t}\}$

Note we usually just take the $e^{i\omega t}$ part as understood and don't include an "$i\omega t$" in our complex representation of the field. In cases where there are signals or fields with different frequencies present in a system we might choose to include it.