Magnetic field visualization based on electron at constant velocity

Question

I, like this person, am trying to get a workable visualization of how electrons moving at a constant velocity give rise to a magnetic field. For the sake of simplicity, we can imagine a single line of electrons caravanning down a conductor.

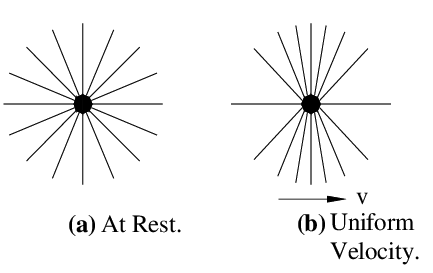
I understand that the field of a charge undergoes a relativistic length contraction when in motion. The images I see associated with this usually look like this:

As a layperson, I can't make any connection from this picture to a rotational field.

The more I look at it, the visual above is probably not even entirely relevant to the "line of electrons" scenario I described, since I think there would be a net cancellation of field lines except those perpendicular to the direction of travel. That realization gets me close to understanding how energy would exist in that orientation, but does not bridge the gap that connects the directionality of travel to the rotational direction of the field.

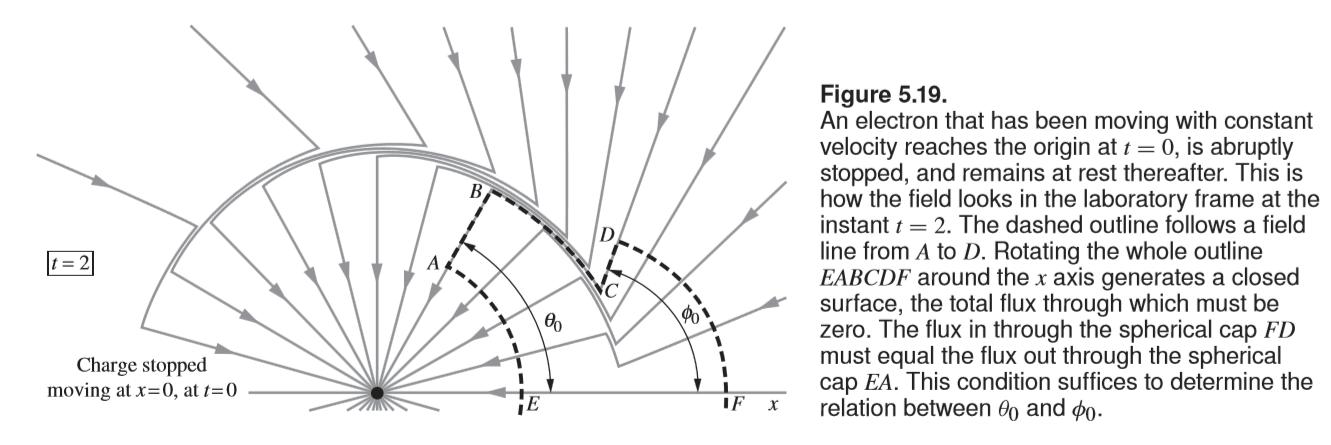
The closest thing I have seen that shows what I think I am looking for are figures like these:

It's a little difficult to follow the story here, but it does otherwise clearly show how a rotational field can arise from the motion of a charge. My hope in looking at this is that my understanding is correct and the curved "shell" field lines are in fact the magnetic field.

Is that the case? If not, is there any better graphical representation that would show how electron motion gives rise to a rotational field?

Author's edit after seeing some answers: I realize now that my initial hope about seeing the magnetic field manifest as plainly as it would seem in the second image was a long shot, and ultimately incorrect. JEB's reference to Jefimenko led me to discover this blog post, which confirmed a suspicion I had:

"But pictures of the electromagnetic field are as rare as hen’s teeth. There’s a gap between the talk and the walk[...]There’s an awful lot of places where you can read about the electric field and the magnetic field as if they’re two separate entities. And there’s virtually no places where you can see the electromagnetic field as the single entity it’s supposed to be. Sometimes it feels like Maxwell’s unification never happened."

I suppose there is a real difficulty in visually conveying the complex relationships at play here, even by way of "3D" animations.

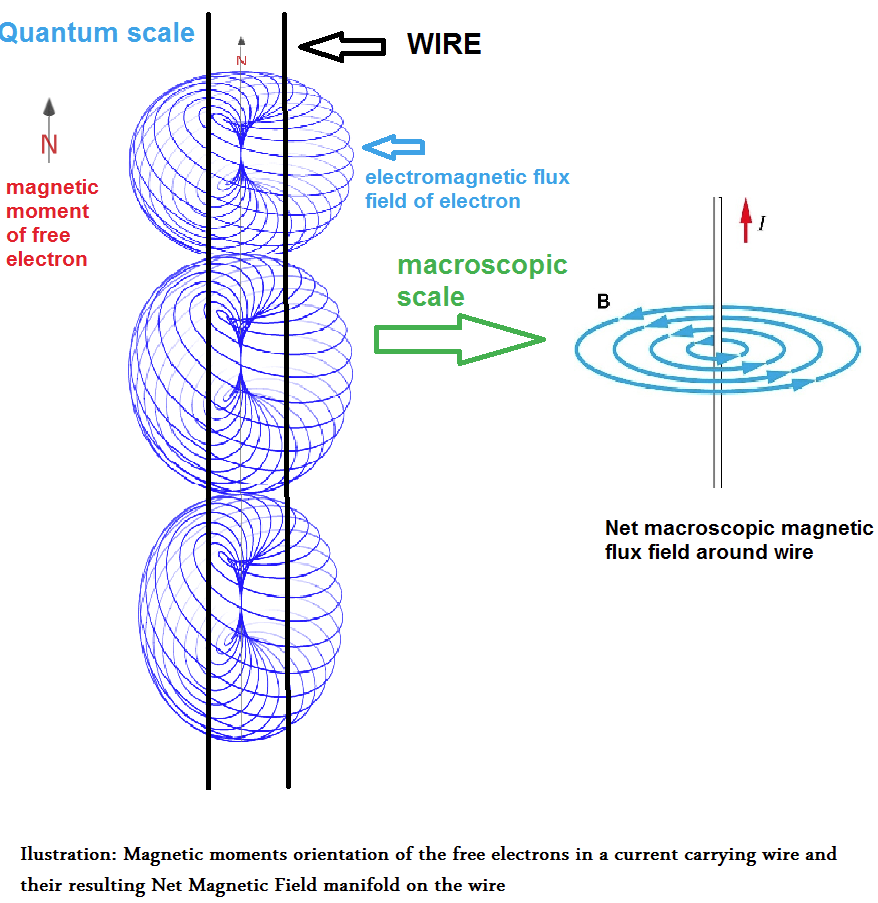
Interestingly, I think one of the images from the linked question is close to presenting a simple representation of what the Physics Detective calls "The Screw Nature of Electromagnetism":

So while I don't have the ability to determine how "mainstream" the text response associated with that image is, the image itself seems helpful.

If anyone else has other ways to visualize this, or even textual analogies, I'm sure I'm not the only one that would benefit.

Answer 1

The answer is: No.

The curved shell in figure 2 is not relevant to the magnetic field (other than: Maxwell's equations--but that is true about any electric and magnetic field configurations).

Since you brought up the boosted electric field:

$$ \vec E'=\gamma(\vec E + \vec v \times \vec B)-(\gamma-1) (\vec E\cdot\hat v)\hat

v $$

which, with no magnetic field is:

$$ \vec E' = \gamma\vec E - (\gamma-1) (\vec E\cdot\hat v)\hat v $$

We can work that out. Note in any multidimensional situation:

1. $\hat z$ is always longitudinal

2. $\hat x$ is always the primary transverse direction

3. $\hat y$ is always OOP (Out-of-Plane), which only matters with 3 body final states, or polarization DoF.

If you violate this: Insta Down Vote.

With that:

$$ \vec E = E\cos(\theta)\hat z + E\sin(\theta)\hat x $$

$$ \vec v = v\hat z $$

Then the boosted term (at origin overlap) are from

$$ \vec E \cdot \hat z = E\cos\theta $$

so

$$ \vec E' = E\cos(\theta)\hat z + \gamma\sin(\theta)\hat x $$

So the longitudinal (aka "parallel") field is unchanged, while the transverse field is boosted by $\gamma$. I would not call this "length contracted". Yes, the shape is, but it's actually getting stronger in the transverse direction.

The magnetic field transform is ($c=1$):

$$ \vec B'=\gamma(\vec B - \vec v \times \vec E)-(\gamma-1) (\vec B\cdot\hat v)\hat v $$

Since $\vec B=0$, that reduces to:

$$ \vec B' = -\gamma(\vec v \times \vec E)$$

In our case, that becomes:

$$ \vec{B}' = -\gamma v ({\hat{z}} \times \vec E) = \gamma v E\sin(\theta)\big(\sin(\phi)\hat x - \cos(\phi)\hat y\big) $$

where $\phi$ is the azimuthal angle.

That is clearly a magnetic field circulating around $\vec v$.

The other option is to use Jefimenko's equation (https://en.wikipedia.org/wiki/Jefimenko%27s_equations) in the frame where the particle is moving. They say:

$$ \vec{B}(\vec r, t) = -\frac{\mu_0}{4\pi} \int \Big[ \frac{\vec r-\vec r'}{|\vec r-\vec r'|^3} \times \vec J(\vec r', t_r) + \frac{\vec r-\vec r'}{|\vec r-\vec r'|^2} \times \frac 1 {c^2} \frac{\partial \vec J(\vec r', t_r)}{\partial t} \Big]dV' $$

where:

$$ t_r = t - \frac{|\vec r - \vec r'|} c $$

is the retarded time.

That is not easy.

The current density at $t=0$ is:

$$ \vec J(\vec r) = q\delta(\vec r)\vec v $$

and so on.