0
$\begingroup$

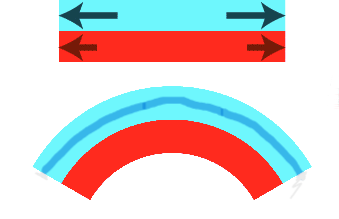
If a bimetallic strip is made by tightly joining two metals (one red and one blue), and the blue metal has a higher coefficient of linear expansion than the red metal, the strip will curl when heated, with the blue metal on the convex side and the red metal on the concave side. The blue metal can also be thought of as consisting of an infinite number of thin elements, but for simplicity, I am focusing on one such element only.

enter image description here

In this case, it seems that the dark blue element of the blue metal expanded less than the surface element of the blue metal. Do different elements of the same metal in a bimetallic strip expand differently, or is my understanding incorrect?

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ Different parts of the metal expand differently because each metal is constrained by the other. That's how bimetallic strips operate. But that doesn't mean the coefficient of linear expansion varies in the metal, if that's what you're getting at. $\endgroup$
    – Bob D
    Commented Sep 18 at 14:38
  • $\begingroup$ Sir is there a resource that discusses the mechanics of how a bimetallic strip works. I searched the web but they just talk about the "racing track" and etc. but not the mechanics. $\endgroup$
    – Sauron
    Commented Sep 18 at 14:50
  • $\begingroup$ Google “Thetmal expansion laminated composite beams” $\endgroup$
    – Chet Miller
    Commented Sep 18 at 15:35
  • $\begingroup$ Look up how a bimetal thermostat works $\endgroup$
    – Bob D
    Commented Sep 18 at 15:40
  • $\begingroup$ Suppose the blue element was not bonded to the red, but you bent it into that arc shape with your fingers. Does that change your question? It should not change your question. The upper part of the arc is stretched relative to the darker blue line, and the lower part of the arc is compressed. What you're doing to the material with your fingers is called stress. How the material responds (e.g., how it bends) is called strain. Bonding to the red element and heating the whole assembly is... $\endgroup$
    – Ohm's Lawman
    Commented Sep 18 at 19:33

2 Answers 2

Reset to default
0
$\begingroup$

You stated the thing slightly wrongly, but your general idea is correct.

Each line, even inside the same material, is expanding a different amount. This is a fact; you can literally see it is true.

The different expansion amounts means that even within each one material, there is a certain bending stress being caused.

Of course, the reason why this is happening, is that the other material is straining a different amount, and that causes the jointed part to have to transfer quite the bit of bending stress over to the other material. That can only happen if both materials are stressed out.

Improve this answer
$\endgroup$
3
  • $\begingroup$ So, is it correct to say that the stress experienced by the element of the blue metal that is in direct contact with the red metal is the maximum, and it gradually decreases as you move toward the surface element of the blue metal? $\endgroup$
    – Sauron
    Commented Sep 18 at 16:35
  • $\begingroup$ Not at all. There might be one line at which it is a minimum or even zero, but it should be a stress distribution pattern that is overly one way at the boundary layer that is stuck to the other material, and then overly the other way at the opposite surface. The minimum CANNOT be assumed to be at the centre of any material and has to be carefully worked out. This assumption is so pervasive that even the great Galileo made the same mistake. $\endgroup$
    – naturallyInconsistent
    Commented Sep 18 at 16:38
  • $\begingroup$ I am having trouble understanding what you just said. Actually, English is not my native language, and I only know basic English. $\endgroup$
    – Sauron
    Commented Sep 18 at 16:47
0
$\begingroup$

Assuming linear elasticity and isotropic materials, Hooke's law describes how solids deform.

Hooke's law in 1D incorporating thermal expansion is

$$\varepsilon = \frac{\sigma}{E}+\alpha\Delta T,$$

with strain $\varepsilon$, stress $\sigma$, stiffness $E$, coefficient of thermal expansion $\alpha$, and temperature change $\Delta T$.

Expressed in words, displacement depends on both thermal expansion and imposed stresses, and the bonding between the strips results in an interface stress state that squeezes the material that would thermally expand more and stretches the material that would thermally expand less. This produces bending (and a longitudinal strain that varies through the materials). See also this derivation.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I know you must be correct, but did you really have to write such a long answer? I really don't think the OP would be able to understand this, but of course it is useful to other readers. $\endgroup$
    – naturallyInconsistent
    Commented Sep 18 at 15:23
  • $\begingroup$ (just for clarity, this answer author took my comment to heart and edited the answer to be much shorter than before.) $\endgroup$
    – naturallyInconsistent
    Commented Sep 18 at 16:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.