Motion of the COM of 2-body system

Question

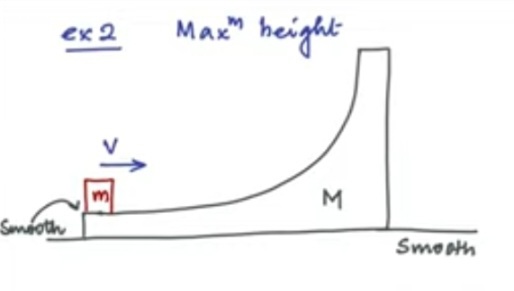
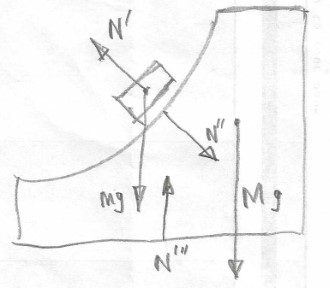
We have a block on a wedge like this;

The block is given an initial velocity $v$,and the wedge is also movable .

So when the block reaches its maximum height on the wedge,the block and wedge are both moving with the same velocity (in the $+x$ direction).

Let's consider a system with the block and wedge

As there were no external forces on the system from when the block was given the velocity $v$, the velocity of the center of mass of the system is constant.

But as the block is also moving upwards, the center of mass (of the system) should also move upwards,right? But...the velocity of the center of mass at the initial time (when the block had velocity $v$ in the $+x$ direction) , was purely at the $+x$ direction,but now it also has a component of velocity in the $+y$ direction (???)

So the velocity of the COM(of the system) changed its direction,that means velocity of COM of the system is not constant,which can't be the case..right? Because $F_{ext}=0$

Where did I go wrong?

Answer 1

There is a normal (vertical) force from the ground to the wedge of mass $M$ that allows for a displacement of the COM in the vertical direction. In other words, this is not an isolated two-body system.

Answer 2

For your block and wedge system the forces acting on and within it are as shown below with the block treated as a point mass and only the directions shown for the forces $N'''$ and $Mg$.

In terms of forces one can say that $N'$ is an internal normal force which is acting on the block and is due to the wedge.
$N''$ is an internal normal force which is acting on the wedge and is due to the block.
$N'$ and $N''$ are equal in magnitude and opposite in direction - N3L.
The horizontal components of these forces are the only horizontal forces acting on parts of the system.
As the horizontal magnitudes of these two forces are equal and act for the same time they apply equal and opposite horizontal impulses on the block and the wedge thus producing equal and opposite changes in momentum of the block and the wedge - conservation of linear momentum horizontally.

$mg, \,Mg$ and $N'''$ are the external forces acting on the system.
The vertically acceleration of the block is determined by the relative magnitudes of the vertical component of $N'$ and $mg$, initially with the vertical velocity of the block increasing and then decreasing.
The horizontal acceleration of the block is determined by horizontal component of $N'$.
The horizontal acceleration of the wedge is determined by horizontal component of $N''$.

External forces $N'''$ and $Mg$ do no work as the displacement of the wedge (CoM) is perpendicular to these forces.
Overall internal force $N'$ and $N''$ do no net work as they act in opposite directions but their displacement are in the same direction.
Thus the only force which does (negative) work on the system is the external force $mg$ (which you could quantify as an increase in the potential energy of the system) with a corresponding decrease in the kinetic energy of the system.

Answer 3

As there were no external forces on the system from when the block was given the velocity 𝑣...

The net external force on the system is $\left[N - \left(M+m\right)g\right]{\hat y}$, where $N$ is the magnitude of the normal force the ground exerts on the wedge.

You're making an assumption that $N = \left(M+m\right)g$.