Ideal and Real Solenoid - Magnetic Field difference

Question

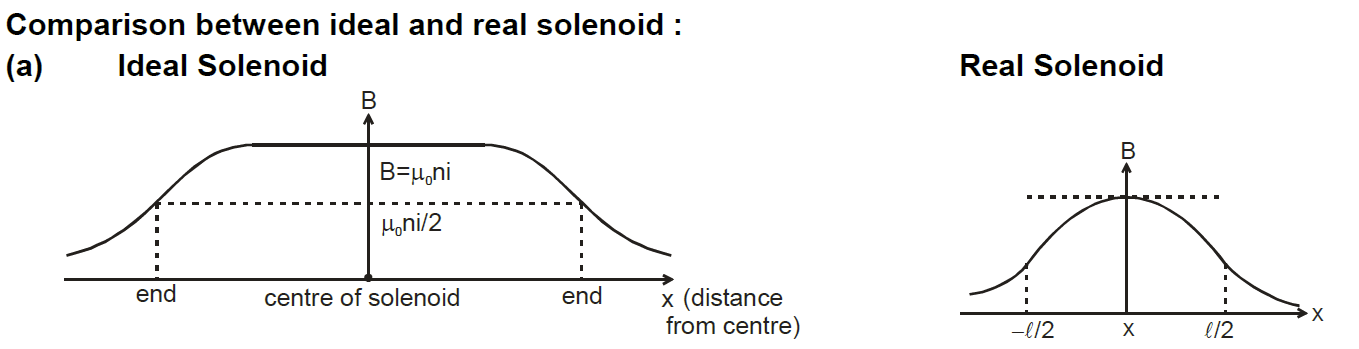
The above graphs show my textbook comparison in the magnetic field inside an ideal and real solenoid. In my opinion, even the graph of an ideal solenoid should be the same as the real solenoid as shown above, but when we mathematically calculate it we find the magnetic field to be constant/uniform inside an ideal solenoid, as depicted in the graph for most of the inner region.

These graphs create a lot of questions to me.

1. Why there is a difference between these two graphs?
2. How is a real solenoid different from an idea solenoid?
3. What is an ideal solenoid? What makes it ideal?
4. What factors all work in a real solenoid, in addition, which are not taken into account for an ideal solenoid?

Kindly help. Thank you.

Answer 1

The left hand graph is of the field at points along the axis of a (real) solenoid with an axial length equal to several times its diameter (a so-called 'long solenoid'). [The word 'solenoid' means tube-like.] The right hand graph is of the field along the axis of a 'flat coil' (a coil whose axial length is much less than its diameter). The terms 'real' and 'ideal' are, in my opinion, inappropriate.

These curves can be plotted from direct measurement of the flux density at points along the solenoid and coil axes, using a Hall probe. The curves are in excellent agreement with the theoretical formulae derived from the Biot-Savart rule. The derivations, to be found in the textbooks, are straightforward. Studying them will answer your question 1.