Why do we define the distance between coils in "Helmholtz coil" arrangement to be equal to radius of the coils

Question

I see that the very definition of Helmholtz coil states that distance between the coils centred on a common axis must be equal to the radius of both of them (which are equal also).

Why is this definition important.

Wikipedia says:

https://en.m.wikipedia.org/wiki/Helmholtz_coil#Description

But I don't really understand what those partial derivatives are trying to say on the wikipedia page.

Also what is a magnetic field gradient. I'm asking this because, apparently Helmholtz coil creates a region of nearly uniform magnetic field gradient when the currents in both its coils are in opposite directions.

Any help is appreciated.

Answer 1

This is a supplement to the answer by @PhilipWood.

An interactive graph can help you visualize what is happening. Here's what I wrote for my class.

---

Helmholtz Coils (robphy) - superposition
https://www.desmos.com/calculator/qbszpfbdgr

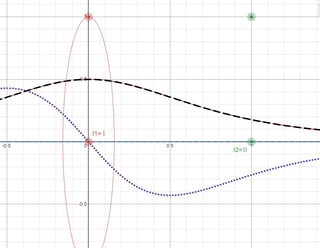
For one loop of radius $R$ and current, here's the field strength (dashed) and its derivative (dotted):

Including an identical loop and oriented-current but translated along the axis by a distance $R$, we have, by superposition,

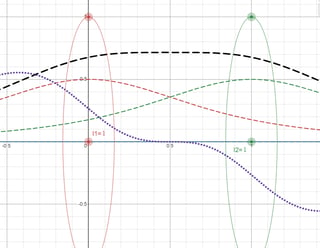
For the best uniform field near the midpoint, we want the flattest graph of $B_z$ near the midpoint.

• Notice how flat the derivative $\frac{dB_z}{dz}$ is near the midpoint of the loop-centers. For identical loops and currents, by symmetry, regardless of the separation, at the midpoint, the odd derivatives are zero.
  • The first derivative being zero says $B_z$ is a local extremum.
  • Although the third derivative is zero, the second-derivative is not generally zero. It turns out (by calculation) that, at the midpoint, the second-derivative is zero when the separation is equal to $R$. (When this is achieved, you have to go to the 4th derivative to get something nonzero.)
• Play around by changing [by dragging control points] the separation between the centers [and by changing the radii] to see a sub-optimal configuration.

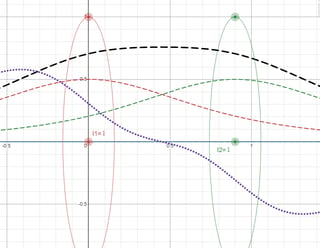
Here it is with separation $0.9R$.
$B_z$ still looks approximately constant near the midpoint, but not as good as when the separation is $R$.

Similarly, with the desmos visualization I wrote, you could also study

• the setup for an optimal uniform-gradient by flipping the direction of one current. (You have to open the "derivatives" folder and display the plot of the second-derivative... then zoom out to appreciate the variations.)
• the setup for the Maxwell coil.

Answer 2

A graph of |magnetic flux density|, $B$, along the axis of a circular coil against displacement, $z$, from the centre of the coil is a bell-shaped curve (though not a Gaussian curve) with its maximum at $z=0$).

For two identical coaxial coils carrying equal currents in the same sense, the resultant B will be roughly constant for a short distance either side of X, a point on the axis midway between the coils. This is because at X itself $\frac{dB}{dz}$ for one coil will be equal and opposite to $\frac{dB}{dz}$ for the other coil. [We have arbitrarily chosen $z=0$ to be at the centre of one of the coils.]

My last paragraph holds whatever the separation of the coils. But when we stray along the axis from X itself, in general $\left|\frac{dB}{dz}\right|$ will be different for the two coils so the change in B from one will not exactly cancel the change in B due to the other. BUT the cancellation will be much better if $\frac{dB}{dz}$ doesn't change much if we stray. So we choose X such that $\frac{d_2B}{dz^2}$ is zero for each coil. It turns out that $\frac{d_2B}{dz^2}=0$ is at a point along the coil axis half a coil radius from the coil centre.