Quantum Beam Splitter Schematic Interpretation

Question

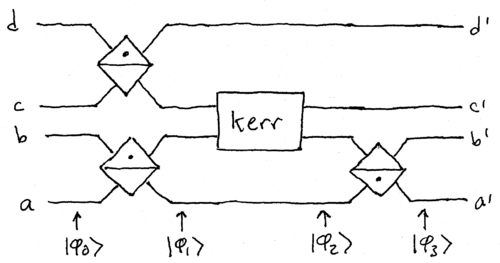
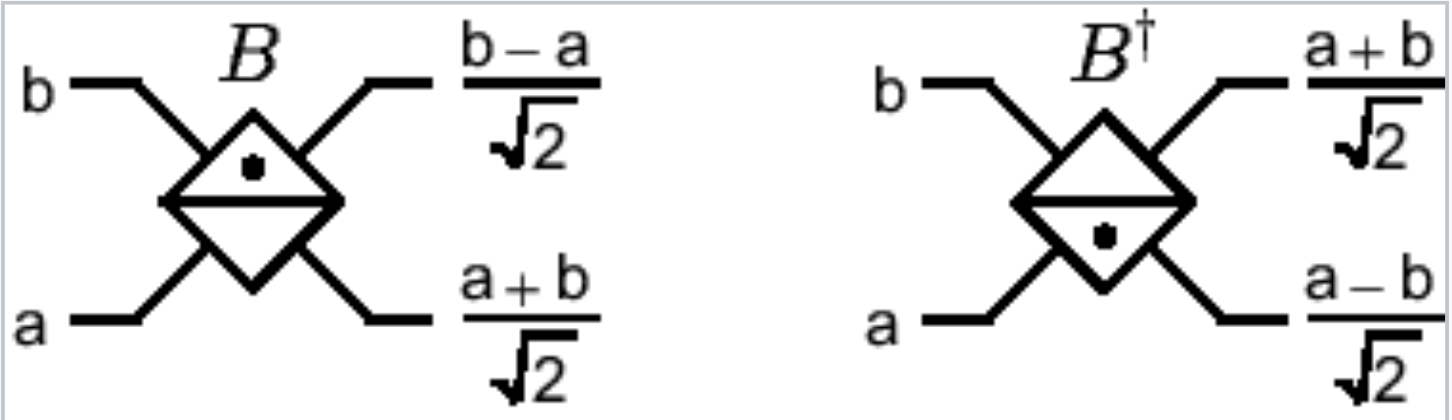I am genuinely confused on how to exactly interpret this element for a beam splitter. This diagram was taken from (https://amowiki.odl.mit.edu/index.php/Single_photons) for reference.

In a beam splitter, I can label the two input modes as $\{a,b\}$ and the output modes as $\{a',b'\}$. When I apply this operator of $B$ the beam splitter to the two photonic states $|ba\rangle$:

$$B|01\rangle = Ba^{\dagger}(B^{\dagger}B)|00\rangle = Ba^{\dagger}B^{\dagger}|00\rangle$$ $$ = (a^{\dagger}\cos(\theta) + b^{\dagger}\sin(\theta))|00\rangle = \cos(\theta)|01\rangle + \sin(\theta)|10\rangle$$

Hence, depending on the angle of the beam splitter, I induce a superposition. Should I have done $B|10\rangle$, then a negative would appear according to the definition they provide in the article.

Why are they labelling the wires coming out of the beam splitter as two distinct superposition? The output state uses both the top wire and bottom wire to express the superposition. To me, this object should have as an input $\{a,b\} = {01, 10}$ and a SINGLE output which is a superposition of the two possible combinations of the state. Why does each output wire contain its own superposition?

When they apply this beam splitter operator to larger circuits, how should I look at $|\psi_1\rangle$ for example is it:

$$|dcba\rangle = \left(\frac{|10\rangle + |01\rangle}{\sqrt{2}}\right)_d \bigotimes \left(\frac{|10\rangle - |01\rangle}{\sqrt{2}}\right)_c \bigotimes \left(\frac{|10\rangle + |01\rangle}{\sqrt{2}}\right)_b \bigotimes \left(\frac{|10\rangle - |01\rangle}{\sqrt{2}}\right)_a $$

But in their description of that circuit, they treat the output of each beamsplitter as either $(|10\rangle \pm |01\rangle)/\sqrt{2}$? If someone could provide clarification on how to interpret the states of each wire, I would greatly appreciate it.

Answer 1

An ideal beamsplitter performs a unitary process. There are no losses. So, when you represent the beamsplitter as a matrix operation then the matrix $M_{bs}$ must be unitary - i.e., $M_{bs}^{-1}=M_{bs}^{\dagger}$.

The are different ways to represent the beamsplitter as unitary matrices. They are all equivalent, because one can always multiply the separate inputs or output by local phase factors without changing the actual state.

So one way is to represent it as
$$ M_{bs} = \left[\begin{array}{cc} C & -S \\ S & C\end{array} \right] , $$ where $C=\cos(\theta)$ and $S=\sin(\theta)$, with the angle $\theta$ parameterizing the reflectivity. If you prefer a more symmetric representation, you can also write it as $$ M_{bs} = \left[\begin{array}{cc} C & iS \\ iS & C\end{array} \right] . $$ Both ways work equally well.