I just ran into the AdS/CFT correspondence, as I am looking at various use-cases of hyperbolic tessellations, specifically related to the pentagrid and heptagrid as defined by Maurice Margenstern in their Cellular Automata in Hyperbolic Spaces work.
I was asking ChatGPT about it, and it led to imagining (without a deep quantum mechanics background), quantum gravity modeled using the $\{7,3\}$ hyperbolic tessellation (heptagrid). How does that work?
Just as lattice models are used in Euclidean space to study quantum chromodynamics (QCD) and other field theories, hyperbolic tessellations can be used to study quantum gravity in AdS space. The discrete heptagonal cells form a lattice that approximates the continuous space.
Just as in lattice QCD, where the continuous space-time is approximated by a finite lattice of points, the {7,3} tessellation approximates the continuous hyperbolic AdS space with a discrete lattice of heptagonal cells.
The larger the tessellation (i.e., the more heptagons included), the better the approximation of the continuous AdS space.
Main question is, how do I conceptually/mentally relate our experience of 3D space, where things are locally connected and such, to an AdS space on the hyperbolic tessellation of $\{7,3\}$? How is it used to model gravity, at a higher level, to point me in the right direction conceptually?
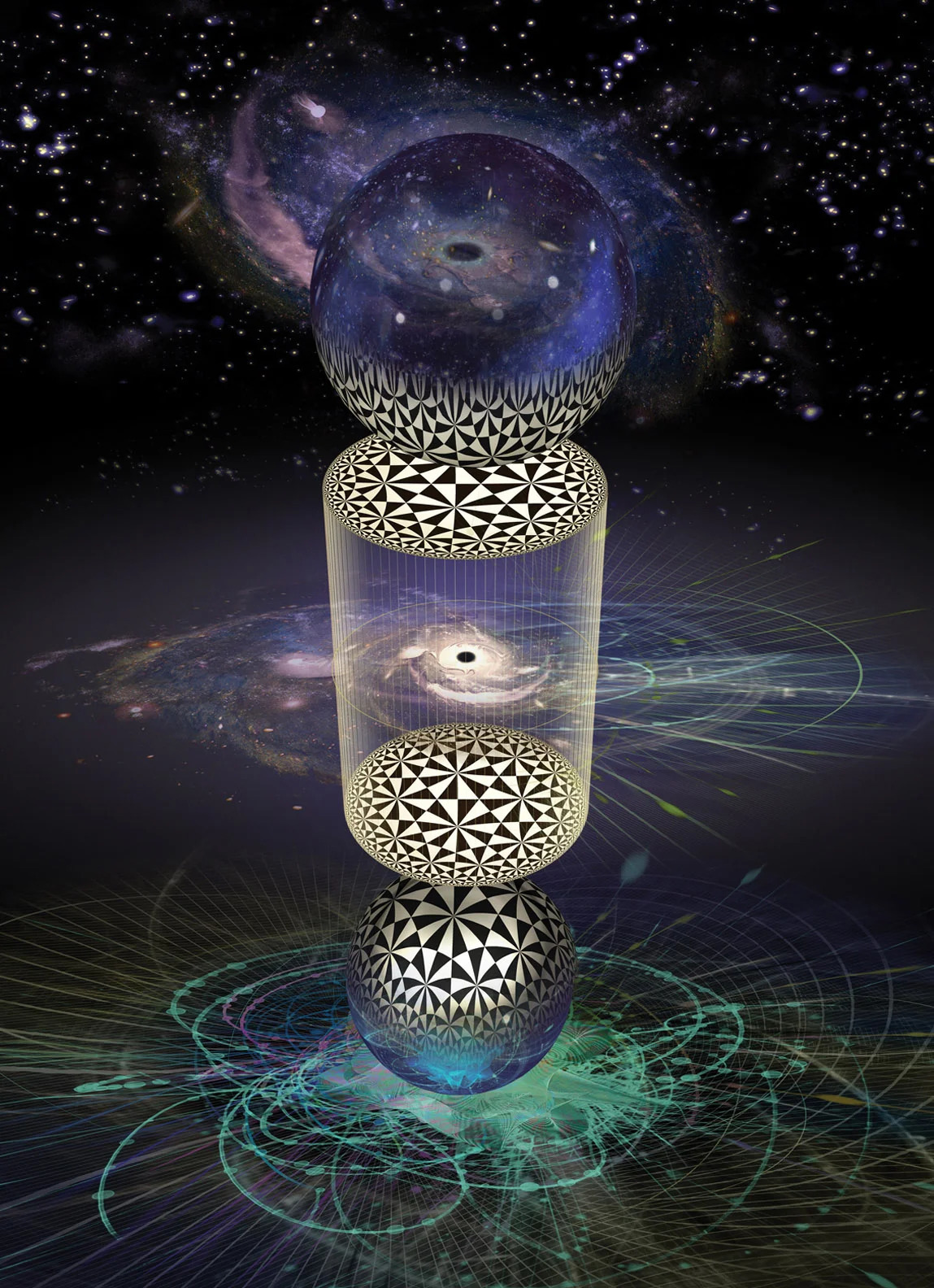
Images such as this make it seem like the hyperbolic Poincaré disk (forming a tube, when projected through time), is somehow a representation of 3D space (or 4D spacetime), but I don't get how the variables are translated from one space to the next. How do you conceptually make the leap from our intuition of the 3D space of the universe, to this hyperbolic tessellation representation? The hyperbolic space is only 2D, so how does it capture 3D relationships?