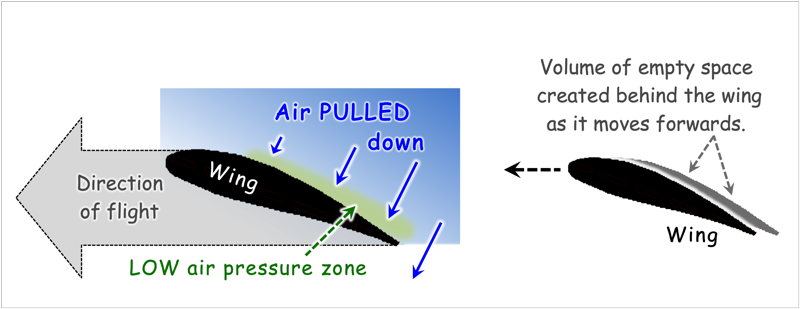
I need a bit of interpretation of your question. If you don't feel comfortable with the idea that the flow on the top of an airfoil can be deviated downwards, you're probably forgetting the role of pressure, or better pressure gradient.
To add some details, it's possible to recast Navier--Stokes equations in a Lagrangian formulation (following the trajectory of a material particle)
$$\rho \mathbf{a} = \rho \mathbf{g} + \nabla \cdot \mathbb{T} \ ,$$
being $\mathbb{T}=-p\mathbb{I}+\mathbb{S}$ the stress tensor splitted as the sum of pressure and viscosity contributions.
Now, it's possible to project this vector equation onto a Frenet bases (tangential, normal and binormal vectors):
$$\begin{cases}
\rho a_t & = \mathbf{\hat{t}} \cdot \left( \rho \mathbf{g} + \nabla \cdot \mathbb{T} \right) \\
\rho \frac{v^2}{R} & = \mathbf{\hat{n}} \cdot \left( \rho \mathbf{g} + \nabla \cdot \mathbb{T} \right) \\
0 & = \mathbf{\hat{b}} \cdot \left( \rho \mathbf{g} + \nabla \cdot \mathbb{T} \right) \ , \\
\end{cases}$$
being $\rho \frac{v^2}{R}=a_n$ the normal acceleration, with $v$ velocity, and $R$ the local radius of curvature of the trajectory, and the bi-normal component of acceleration $a_b=0$ by kinematics.
Working with high-$Re$ flows outside the (thin) boundary layer over an airfoil at small AOA, viscous stresses are negligible, so that the stress tensor becomes
$\mathbb{T}=-p\mathbb{I}$ and the equations (neglecting volume forces as well) read
$$\begin{cases}
\rho a_t & = - \mathbf{\hat{t}} \cdot \nabla P \\
\rho\frac{v^2}{R} & = - \mathbf{\hat{n}} \cdot \nabla P \\
0 & = \mathbf{\hat{b}} \cdot \nabla P \ , \\
\end{cases}$$
As you can see from the tangential component of the vector equation,
the opposite of the derivative of the pressure field along the trajectory is proportional to the tangential acceleration (and thus it makes the absolute value of the velocity increase)
the opposite of the derivative of the pressure field in the normal direction is proportional to the "normal" centripetal acceleration. As a simple example of a flow where you can easily find this contribution, you can think at any 2D-flow with circular trajectories, either rotational or irrotational. Even more qualitatively, like the flow of a tornado swirling around a low-pressure central point.
Short remark about signs. Focusing on the normal component, Frenet basis is defined with the normal vector pointing "inwards" towards the center of curvature of a line, here the trajectory. On the LHS we have a positive term (density, absolute value of the velocity squared, radius of curvature all positive), thus the derivative of pressure field along the $\mathbf{\hat{n}}$-direction must be negative to make the RHS positive, i.e. pressure "decreases towards the center of curvature".
On the other hand, if I have to re-interpret the title of your question as "explaining lift as a sum of elementary forces", the only answer I'd feel to give starts from the expression of the force acting on a solid body surrounded by a fluid,
$$\mathbf{F} = \oint_{S_{solid}} \mathbf{t_n} \ .$$