0
$\begingroup$

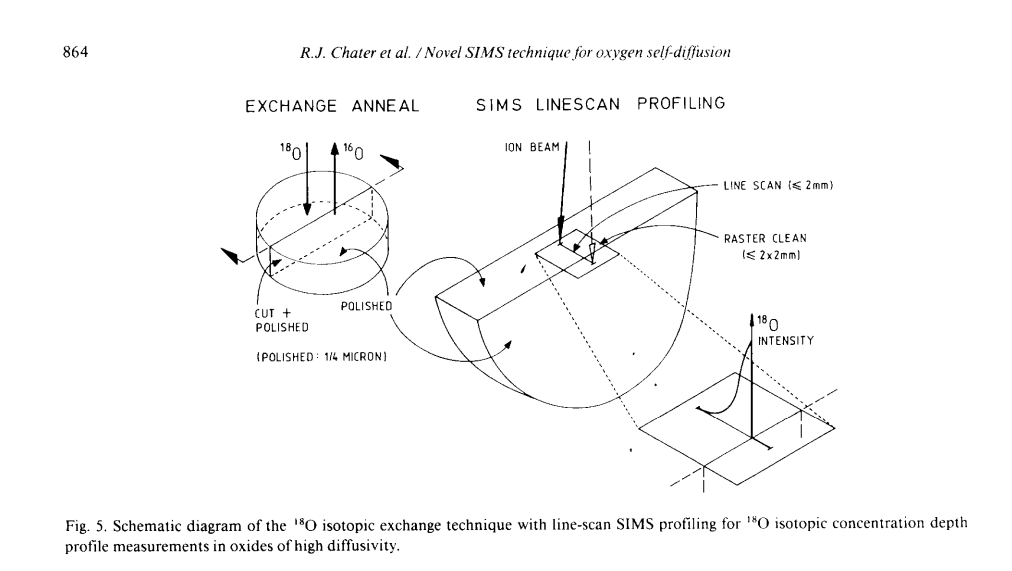
Suppose there is a cylindrical (pellet) sample in the oxygen atmosphere as shown on the photo attached. Oxygen diffuses from the outside to the sample interior everywhere on the outer surface of the sample. From the photo, it can be seen that diffusion profile of oxygen is measured in the axial direction of the cylinder. In another words, diffusion is regarded as one-dimensional even though the sample is three-dimensional. I'm not sure how is it possible that one-dimensional solution of the diffusion equation can be applied here?

Oxygen can move in all three dimensions and will do so if partial derivative of concentration is non-zero in the other two Cartesian coordinates (cylindrical coordinates are more appropriate here, but that's not super important for the question).

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

The source is Chater et al., "Development of a novel SIMS technique for oxygen self-diffusion and surface exchange coefficient measurements in oxides of high diffusivity".

The coupons are reported to be about 1 mm thick, and if the diagram is anywhere close to scale, they are many millimeters wide. (I don't think it's mentioned—the authors evidently assume they are wide enough that the width is unimportant.)

Detectable diffusion of the radio-labeled $^{18}\mathrm{O}$ occurred over <0.4 mm, and the spot size being investigated is less than 10 µm. Therefore, the diffusing atoms near the center don't "know" about the side of the coupon many millimeters away. (Indeed, this is why a disc shape was likely chosen, rather than, say, a cube or sphere.) Nor do they "know" about the opposite side of the disc.

So edge effects and lateral diffusion and finite sizes in general aren't relevant to the model or study. As far as diffusion at the center of the coupon is concerned, the process is occurring across and past a seemingly endless plane, into a bottomless region. This justifies the use of a one-dimensional diffusion model into a semi-infinite medium (sometimes called a "half space").

It would have increased clarity if the authors had noted something like "The sample was sufficiently wide and thick that diffusion from the sides, edges, and opposite side was undetectable and could be ignored, with the resulting lateral translation symmetry justifying simplification of Fick's laws to a one-dimension model of a semi-infinite medium."

Improve this answer
$\endgroup$
4
  • $\begingroup$ Yes, diffusion length is small compared to the sample dimensions. Even though atoms in the centre don't feel the edge effects, they can still diffuse in the other directions (not only axial) given that the sample has three dimensions. $\endgroup$
    – Dario Mirić
    Commented Mar 22 at 11:10
  • $\begingroup$ I guess that the concentration of the tracer is the same at every point on every horizontal plane (if edge effects can be ignored) of the sample due to the fact that the same physical phenomenon happens at every point on the plane. In another words, if you take a look at the upper surface of the sample, at every point there, the same process occurs and hence concentration of the tracer is the same at every point in time on that plane. There is a symmetry, process looks the same everywhere on the plane. $\endgroup$
    – Dario Mirić
    Commented Mar 22 at 11:15
  • $\begingroup$ This makes directional derivative of concentration zero in every direction except for the axial. $\endgroup$
    – Dario Mirić
    Commented Mar 22 at 11:17
  • $\begingroup$ Yes: A 1D model in $x$ implies lateral translational symmetry in $y$ and $z$, so $\nabla$ can be replaced by $d/dx$. $\endgroup$
    – Chemomechanics
    Commented Mar 22 at 17:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.