When you consider the positive terminal of battery, wire and capacitor plate, they form a single conductor, which is polarised by the field of the other capacitor plate, but it is not polarised as much as it would if the wire to the battery were longer or stretched to infinity. Surely the maximum charge held on the capacitor plate for a given charge on the other plate occurs when the wire to the battery is longer? So $c_{12} $ would increase. For instance, when grounded the voltage of charge that is pulled onto the one conductor is nearly equal and opposite to the voltage at that point within the external field of the other conductor; however, when the conductor is ungrounded it is only slightly polarised because only a small amount of field passes across it, which appears to be the case of a terminal of a battery in close proximity configuration?
-
$\begingroup$ Your question is not clear. With "polarized" you mean charged? The charge does not depend on the length of the connecting wire, except if you consider the resitance of the wire, the higher it is the longer it takes to reach the final charge. Also you are unclear with "grounded" instead of talking of the second end of the batterie. $\endgroup$– trulaCommented Feb 21 at 12:18
-
$\begingroup$ The field outside an ideal capacitor is zero. So only the internal faces of the capacitor plates carry a charge. The outer face of the plates, the wires and the battery carry no charge. Or more precisely negligible charge since while wires and battery do in principle have their own self capacitance but it is vanishingly small. $\endgroup$– John RennieCommented Feb 21 at 12:34
-
$\begingroup$ @JohnRennie I could use one of those ideal capacitors. Where can I get one? $\endgroup$– John DotyCommented Feb 21 at 22:23
3 Answers
You are correct: the capacitance does depend on the geometry of the wires connecting it. We commonly account for that extra capacitance as a separate "stray capacitance" in parallel with the capacitor. There are also stray capacitances between the capacitor and the surrounding environment. They are often small enough to ignore in real life, but they are sometimes important. The presentation is attempting to avoid confusing you with this often negligible effect, but of course it actually produced more confusion.
Physics texts should start out with a disclaimer.
Everything in this book is wrong. The physics is idealized to allow us to focus on particular features of reality in isolation. But such perfect isolation never exists in reality. We are presenting stories about physics: models. "All models are wrong, but some are useful." -George Box. We hope you will find these models useful.
For a plate configuration of a certain capacitance, C, the charges on the plates are determined just by the value of $C$ and the applied potential difference, $\Delta V$, between the plates. $Q=±C\Delta V$. When a battery is connected to a capacitor, there is current through the connecting wires until $\Delta V$ is equal to the pd between the battery terminals, which is then equal to the battery emf, $\mathscr E$. Even if there had been a potential drop across the wires while the capacitor was charging, Ohm's law shows that there won't be when $\Delta V$ has reached its final value and there is no current. So the length of connecting wires is irrelevant to the final value of $\Delta V$, and the final charges on the plates are $±C\mathscr E$.
Having ruled out any effect of the length of connecting wires on the final $\Delta V$, you might wonder if it could affect the charges on the plates by changing $C$. The answer, for any normal capacitor with plates of much greater linear dimensions than their separation, is no, not significantly – see John Rennie's comment.
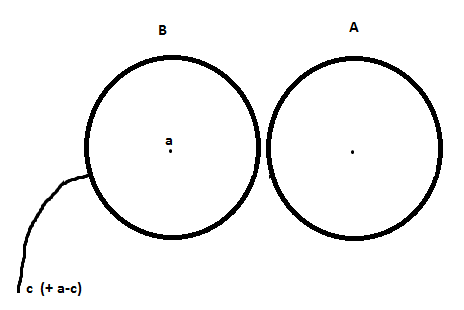
If sphere A has a charge of Q and a radius of 1cm, then the voltage is 100kQ. If sphere A has a radius of 1cm and the gap between the 2 spheres is negligible, then we can say that a is 2cm away, and therefore the voltage in the field of A at point a is 50kQ. Assuming c is 3cm away from the centre of A, the voltage at point c in the field of A is 33.3kQ. The induced voltage is therefore going to be roughly 50kQ-33.3kQ = 16.67kQ.
The battery connected to c will therefore only provide 16.67kQ of voltage to the configuration of the wire and sphere B to cancel this out and cancel out the potential difference across the battery internally. This is short of the 50kQ that would be provided by earth if it were earthed.
The next thing I tried was making the spheres as small as possible, and reducing Q proportionally. Say now that sphere A has a charge of 0.0001Q, and a radius of 1um then the voltage is 100kQ and the voltage at a is 50kQ, but the voltage at point c in the field is now 0.00333kQ, so the voltage induced at c is 49.99667kQ, which is much closer to the amount that would provided by earth.
The problem with this scenario is that the smaller the sphere gets the more significant the wire becomes geometrically and the geometric centre (centroid) would start to move towards c, which would result in the voltage at a decreasing, and the potential difference between a and c becoming a lower fraction.
I realised that this is not a problem in a capacitor because it is a large flat plate instead of a sphere, so therefore the geometric centre remains on the plate due to all the area on the plate, and because the plates are extremely close together on a micrometer scale like the two small spheres are, the field at c is very small. Also, because they are flat plates, the geometric centres are closer together than the centres of the spheres because the sphere centres are limited to a minimum separation of 2r, which means that the voltage at the centre of the plate in the field of the other plate can also be more than 1/2 the voltage of the other plate, and closer to the full voltage of the other plate.
Another thing I noticed is that it looks like the field from A also passes over the battery, which would be the same magnitude of field as the field coming from point c, and also would be decreasing at less of a gradient due to being further away, which would affect the calculation for voltage provided by the battery to the conductor configuration. This field is cancelled out by the field coming from the charge that migrates to the other side of point a. It is better to visualise it as point charges according to the model of method of image charges for an ungrounded spherical conductor
The two charges' fields almost completely cancel out due to the close proximity of the charge (which wouldn't be able to be achieved if conductor A were a sphere as opposed to a point or capacitor plate), and only the Q at the centroid a is left. The battery therefore needs to provide -Q of charge to the conductor configuration to cancel out the field from the centroid a across the battery internals, which will be the voltage of the battery (because it's the Q that was induced on conductor A by the battery).