Rolling of a disc and translational part of it

Question

In case of rolling the rotational velocity of the disc $wr$ will have magnitude equal to $v$. But now the top part of the disc has net velocity $2v $. Does that mean the top part of the disc moves by distance 2v evry second while othe parts of disc move less than that? And while writing it's total kinetic energy (of a particle kept at top of disc) will the velocity (translational) be 2v? And what wold be expression of it's total energy?

Answer 1

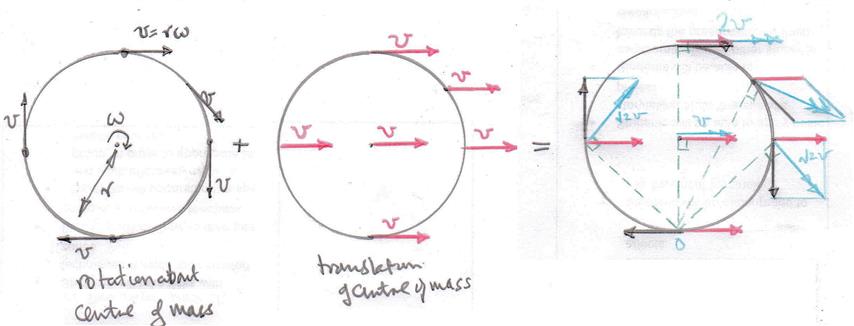
You can consider the instantaneous velocity of any point in the disc (blue) as the sum of the velocity of the centre of mass of the disc (red) and the instantaneous velocity due to the rotation of the disc about the centre of mass (grey).

Thus the top of the disc has a velocity of $2v$ for an instant of time with a speed of less than $2v$ before and after that instant.

Two ways of evaluating the kinetic energy of the disc is as follows.

• Translational kinetic energy of centre of mass plus rotational kinetic energy about the centre of mass $\Rightarrow \frac 12mv^2 + \frac12 I_{\rm com}\omega^2 = \frac 12mv^2 + \frac12 \,(\frac 12 mr^2)\,\omega^2 = \frac 34mv^2$ because for no slipping $v=r\omega$
• Rotational kinetic energy about the stationary point of contact $\Rightarrow \frac 12 I_{\rm contact}\omega^2=\frac 12 (I_{\rm com}+mr^2)\omega ^2 = \frac 34mv^2$ as before.

Answer 2

You need to discern material points and geometric points:

• let's call $T$ the geometric point identifying the top of the disc at all the times;
• let' call $P$ a material point, s.t. $P \equiv T$
• let's call $D$ a material point on the surface of the disc.

Here some facts will show below:

• The geometric point $T$ (and the material point $P \equiv T$) always has velocity $\mathbf{v}_T = \mathbf{v}_P = \Omega R \mathbf{\hat{x}}$;
• the material point $D$ has velocity $\mathbf{v}_D = 2 \Omega R \mathbf{\hat{x}}$ only when it passes through $T$;
• all the material points, when they pass through $T$, always has velocity $\mathbf{v} = 2 \Omega R \mathbf{\hat{x}}$

Kinetic energy. In the expression of the kinetic, you need to deal with the velocity of the material points. If the system has a point mass in $P$, its contribution to kinetic energy simply is

$$K_P = \frac{1}{2} m_P |\mathbf{v}_P|^2 = \frac{1}{2} m_P R^2 \Omega^2 \ .$$

If the system has a point mass in $D$ constrained on the surface of the disc, its contribution to kinetic energy is

$$K_D = \frac{1}{2} m_D |\mathbf{v}_D|^2 \ ,$$

where the velocity $\mathbf{v}_D$ is the time derivative of the position of $D$.

Kinematics. Velocity of points could be readily obtained as the time derivative of their position, $$\mathbf{v} = \dot{\mathbf{r}} \ .$$

Using Cartesian coordinates and the angle rotation angle $\theta$, s.t. $\Omega := \dot{\theta}$, and the assumption of pure rolling, the position and the velocity of points $P$, $D$ read:

$$\begin{aligned} & \begin{cases} \mathbf{r}_P = R \theta \mathbf{\hat{x}} + 2R \mathbf{\hat{y}} \\ \mathbf{r}_D = R \left(\theta - \sin \theta \right) \mathbf{\hat{x}}+ R \left(1-\cos \theta\right) \mathbf{\hat{y}} \\ \end{cases} \\ & \qquad \rightarrow \qquad \begin{cases} \mathbf{v}_P = R \Omega \mathbf{\hat{x}} \\ \mathbf{v}_D = R \Omega \left( 1 - \cos \theta \right) \mathbf{\hat{x}} + R \Omega \sin \theta \mathbf{\hat{y}}\\ \end{cases}\end{aligned} $$

Remark. It's easy to check that when $P \equiv D$, i.e. $\theta = \pi$, the velocity of $D$ reads

$$\mathbf{v}_P(\theta = \pi) = 2 R \Omega \mathbf{\hat{x}}$$

Answer 3

At a particular instance, the bottom most point (let say B) will have 0 velocity and at that same instance a point A which is at top most will have velocity equal to 2v and we know that the top most point will keep on changing every sec. So if I point a laser light on the top most point of the ring/wheel and move that source of laser light such that the light always incident perpendicular to the wheel then I think YES We can say that the laser will move with a speed of 2v per sec.

And if we talk about the velocity we use in finding the total kinetic energy of the body doing translatory as well as rotation motion, in that case we use velocity of centre of mass of the body. So we will be taking v instead of 2v.