Reflection from a spherical surface

Question

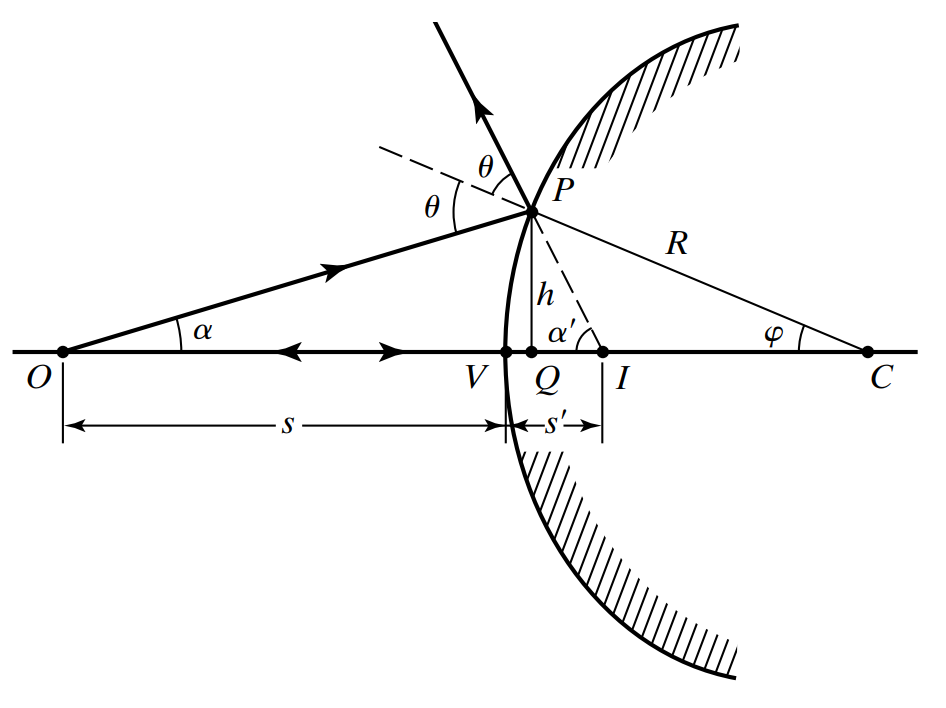
I am self-studying Optics by Pedrotti and I'm stuck on the algebra for an example of spherical reflection.

We have a point source at O that reflects off a spherical surface and creates a virtual image at I.

Assume that $\alpha$ and $\phi$ are small so that the small angle approximation holds. Then

$$ \theta = \alpha + \phi $$ $$ 2\theta = \alpha + \alpha' $$ so $$ \alpha - \alpha' = -2\phi $$ Applying the small angle approximation, $$ \frac{h}{s}-\frac{h}{s'}=-2\frac{h}{R} $$

The thing I'm stuck on is pretty simple. If I apply the small angle approximation to $\alpha'$, for example, I get $$ \alpha'\approx \tan\alpha' = h/x $$

where $x$ is the length of the adjacent side of the triangle. But how can I argue that $x\approx s'$. I can "see" it from the limit as the angles become small, but how do I argue this mathematically?

---

EDIT

Reading further a bit, Pedrotti writes (without much elaboration)

... where we have also neglected the axial distance $VQ$...

But that's not very satisfying! So I'm still wondering what the mathematical argument is that $x\approx s'$.

Answer 1

The difference between $s' = VI$ and $x = QI$, $s' - x = VQ$, is equivalent to the length $VC - QC = R - R\cos\varphi$. Substituting the Taylor expansion $\cos\varphi \approx 1 - \frac12\varphi^2$ shows that the difference is 2nd order in $\varphi$: $$s' - x \approx \frac12 R\varphi^2$$ Which can be neglected for small $\varphi$, meaning $s' \approx x$.