0
$\begingroup$

The following problem is given (from PHYSICS For Scientists and Engineers with Modern Physics - text book):

"A home run is hit in such a way that the baseball just clears a wall 21 m high, located 130 m from home plate. The ball is hit at an angle of 35° to the horizontal, and air resistance is negligible. Find the initial speed of the ball".

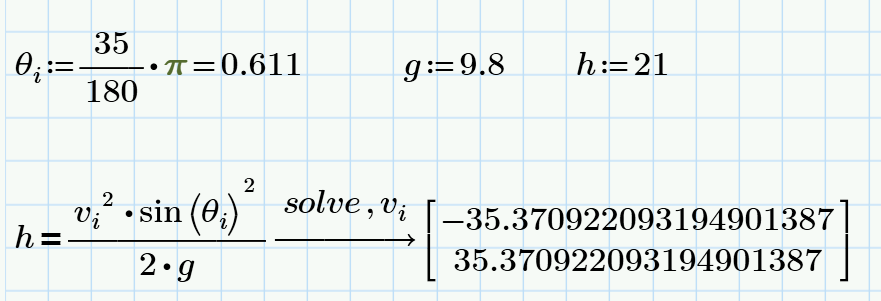
I made the assumption in solving the problem, that the ball reaches its maximum height exactly at the wall and therefor I solved for the initial speed in the maximum projectile height equation: enter image description here

This yielded as a plausible result ~35.37 [meters/second].

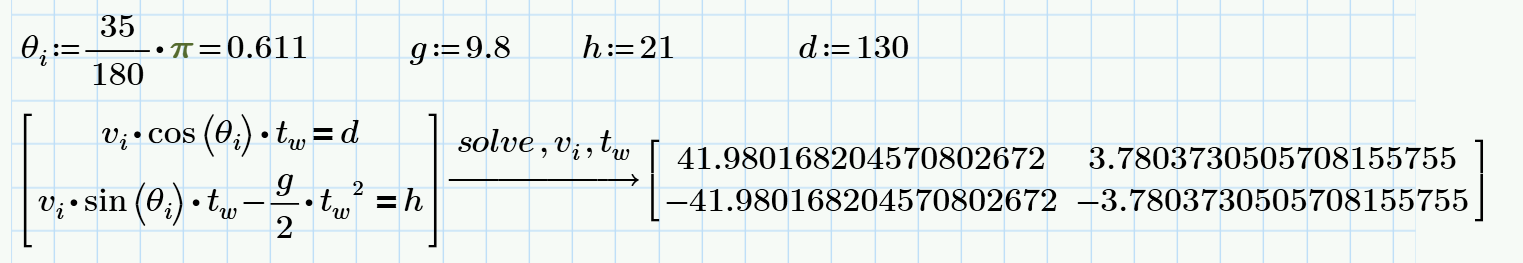
While cheeking with the textbook answers, I've seen they got a different result.They reached to the different result by not making any assumptions on the trajectory of the projectile, so in effect you get to solve the following simulations set of equations (for horizontal and vertical motion): enter image description here

This yields as a plausible result for the initial speed: 41.98 [meters/second].

So I've plotted both trajectories, and seen that both paths pass trough (130;21) meter point.

My question from all this would be: why am I not getting all possible trajectories (/speeds) as a solutions, if I am not imposing any constraints to the trajectory and solve the more general set of equations ?

Thank you!

Improve this question
$\endgroup$
4
  • $\begingroup$ As a partial answer, because there's squares in your equation, it is highly likely that there will be multiple solutions. Most numeric solvers are not designed to exhaustively find all solutions -- they simply find one. $\endgroup$
    – Cort Ammon
    Commented Jan 23 at 17:04
  • $\begingroup$ You don't use the distance to the wall anywhere in your approach, but the required speed of the ball should clearly depend on how far you need to hit it. There's also no reason why the ball should be at its maximum height when clearing the wall - "just clears the wall" means that the ball could be going no slower and still make it. If the wall is very close, the ball might still be on its way up, if the wall is very far, it might be on its way back down. At most wall distances, it's impossible for a ball hit at this angle to just clear it at the apex of its trajectory. $\endgroup$
    – Nuclear Hoagie
    Commented Jan 23 at 17:08
  • $\begingroup$ @CortAmmon it happens to only have one unique solution, despite the appearance of squares. At least, only one tolerable solution. $\endgroup$
    – naturallyInconsistent
    Commented Jan 23 at 17:18
  • $\begingroup$ Please don’t post images of mathematics. Please use MathJax instead. $\endgroup$
    – Ghoster
    Commented Jan 23 at 17:32

2 Answers 2

Reset to default
0
$\begingroup$

from PHYSICS For Scientists and Engineers with Modern Physics - text book

Surely you can see that with a title so generic, it is necessary to also include the authors so that people can find the book.

So I've plotted both trajectories, and seen that both paths pass trough (130;21) meter point.

This is absolutely a lie. Only the actual answer will do this. Yours will only get to (60,21)

In fact, with a bit of manipulation, it is easy to see that there is only a unique possible $v_i$ that will satisfy the requirements, and I can eliminate $t_w$ without needing to find it. The textbook answer key is correct.

Improve this answer
$\endgroup$
1
  • $\begingroup$ You are right, sorry I had an mistake when plotting. Thanks for the help $\endgroup$
    – Bogdan Sofalca
    Commented Jan 23 at 18:10
0
$\begingroup$

You're indeed imposing constraint by saying that the ball crosses the wall at his maximum height, and I guess that is not what the textbook wanted you to do. I suppose that the exercice is asking you to find the lowest velocity such that the ball doesn't hit the wall. So you have to solve this problem by setting :

$ y(x=130) = 21 $

Hope this helps !

Improve this answer
$\endgroup$
2
  • $\begingroup$ I understand that part. What I am asking is why am I not able to find all solutions by just writing down (and solving) the kinematic equations without constraints. i am only able to find one of the trajectories this way $\endgroup$
    – Bogdan Sofalca
    Commented Jan 23 at 17:18
  • $\begingroup$ How would you solve the kinematic equations without any constraints ? $\endgroup$
    – Tristan Diotte
    Commented Jan 23 at 17:31

Not the answer you're looking for? Browse other questions tagged or ask your own question.