I lately have been confused by the work energy theorem which states that:
Work done by all the forces on an object is equal to the change in kinetic energy.
However, I have a doubt
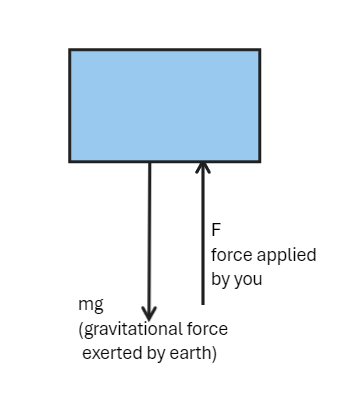
Consider we have an object resting on the ground. We lift the object from the group and bring it to a height say $h$. We ensure that the work done in bringing the object above the ground is done without giving it any velocity (By applying a force $F = mg + dF$, where $dF > 0$). Now, the work done by gravity = -mgh. (h<<R). Now, applying Work-energy theorem:
δ(K.E) = -mgh (neglecting air friction)
But in this case , change in Kinetic energy will be zero... hence implying that mgh = 0, and h = 0 but this is not the case.
So could anyone please tell me what am I doing wrong here?