In an ideal circuit with two wires in parallel, if one path has zero resistance and another has a nonzero resistance, all of the charges will flow through the wire with zero resistance (if I understand correctly). How would the current be distributed if both wires had zero resistance?
Edit: For clarification, I'm asking what would happen if both wires were ideal superconductors.
This is a more general case of two ideal inductors in parallel. Two understand how current is shared between two perfectly conducting paths, we need to consider magnetic fields.
Consider the circuit above. The red segments have zero resistance, and the dashed lines indicate the rest of the circuit which might contain various other components. $\Phi$ is the magnetic flux through $C$ formed by the perfect conductors. We can write $$\Phi = -L_1 i_1 + L_2 i_2.$$ The two terms are the fluxes due to the two currents, respectively. $-L_1$ is technically the mutual inductance between loop $C_1$ and $C$, and $L_2$ is that between $C_2$ and $C$. Note that in general, the non-ideal segments also contribute to these inductances (not just the perfectly conducting loop), so the geometry of the whole circuit matters here.
We write Faraday's law along $C$: $$\oint\limits_{C}\vec E\cdot d\vec\ell=-\frac{d\Phi}{dt}$$ Since $\vec E = 0$ in perfect conductors, this integral is zero and we have $$\frac{d\Phi}{dt}=-L_1 \frac{di_1}{dt} + L_2 \frac{di_2}{dt}=0.$$
Integrating from $0$ to $t$ as in this answer, $$L_1\int\limits_0^t \frac{d}{d\tau} i_1(\tau)~d\tau = L_2\int\limits_0^t \frac{d}{d\tau} i_2(\tau)~d\tau$$ $$L_1\left[i_1(t) - i_1(0)\right] = L_2\left[i_2(t) - i_2(0)\right].$$ This is the general current division rule. If both branch currents are zero before you "flip a switch" and push current through the circuit (i.e. $i_1(0) = i_2(0) = 0$), then we have the simpler form: $$\frac{i_1}{i_2}=\frac{L_2}{L_1}.$$ Note that the initial condition $i_1(0)=i_2(0)=0$ is important and not trivial: a perfectly conducting loop could sustain a current indefinitely with even when not powered by an external circuit.
You would have to define what is joining them.
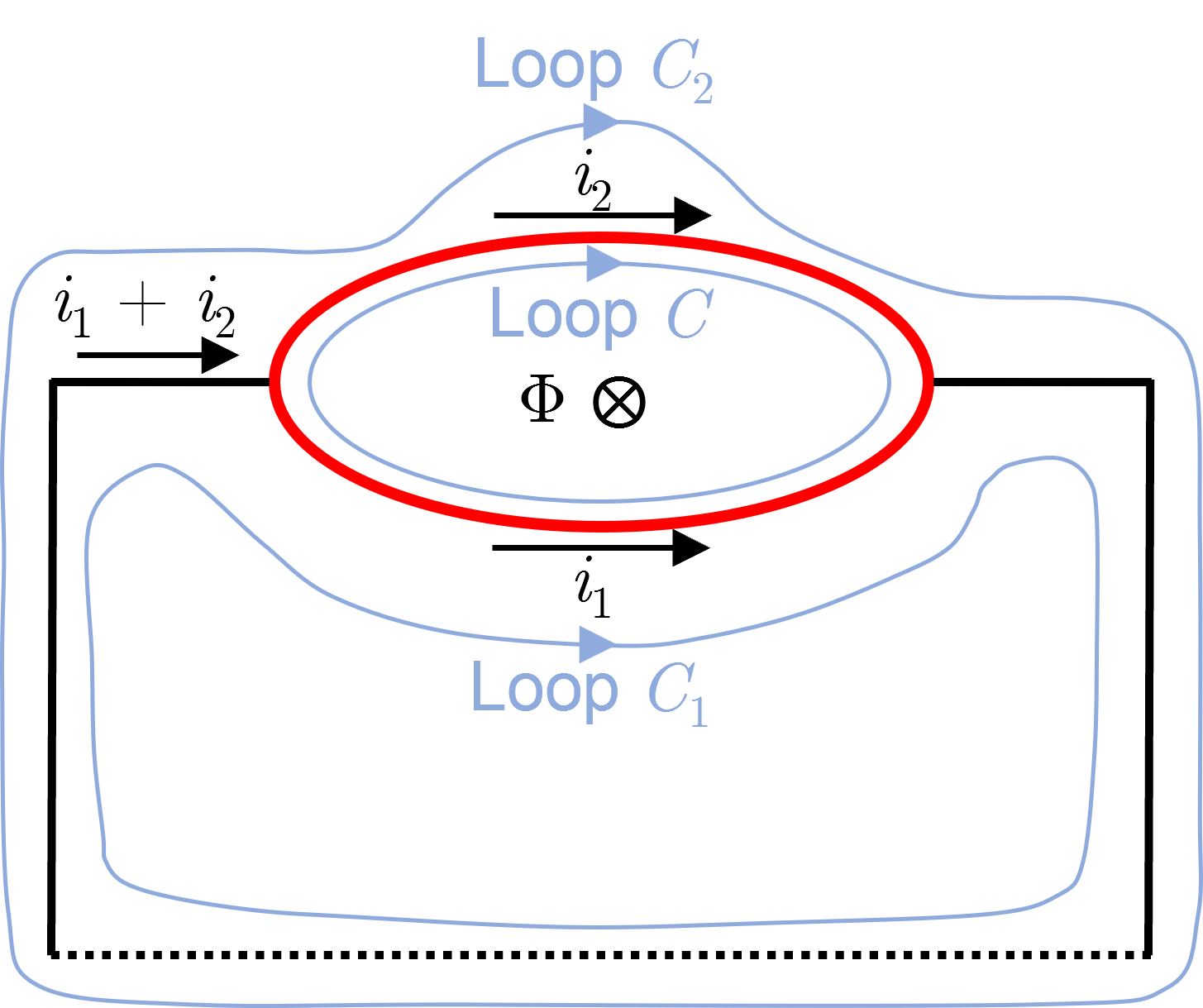
If they are joined by a superconductor between either side, then you have created an entire loop of superconductor, and this by the Meissner effect traps a certain amount of magnetic flux because magnetic field lines cannot get through the loop. The current through either branch will depend on not just the magnetic field trapped inside, but what the external world is trying to do to change that field.
So for example, you set up a magnetic field, then cool beneath the critical temperature, trapping those field lines. You haven't done anything to the system yet so presumably there is no net supercurrent to consider. But then suppose you turn off the external magnetic field. One of your superconducting wires now sees a positive supercurrent, the other sees the negative, so that the current around the loop generates the trapped magnetic field as a simple magnetic dipole field. Want to reverse the current? Turn on the magnetic field again but make it too strong, $2B$, and now the supercurrents need to reverse.
If they are joined with normal conductor (or doped semiconductor), then this is called an NSN junction. Superconductors weren't really my area of study during my MSc so please take everything I say with a grain of salt, but my understanding was that there are a couple phenomena that govern this transport.
First, different charge carriers. Currents in the N regions are carried by either electrons or holes, spin-½ particles and quasi-particles respectively. (Not 100% sure everyone will agree with using the word “quasiparticle” here but given that the holes existence depends on all of the other electrons in the band moving I think I can defend it?) Currents in the S regions are carried by a spin-0 singlet or spin-1 triplet state, so that those carriers can mathematically form a Bose-Einstein condensate. In the usual BCS model this is formed by pairing electrons with opposite momentums into “Cooper pairs” which operate as a different sort of quasiparticle. So to create a Cooper pair at the interface requires not just a electron to fly into the superconductor, but a hole to fly back out of it: this is called Andreev reflection.
This will probably also limit what sorts of voltages you can put across the NSN junction, there is a band-gap energy forbidding normal electron transport through the superconductor, if you put a bias voltage greater than that band-gap then you will just start to excite normal conducting electrons which will flow with normal resistance which will heat up your superconductor and then you will lose superconductivity.
Second, and getting much closer to your question, this probably means that electron transport at low bias needs to be understood via quantum tunneling processes, electrons “hop” into being Cooper pairs and “hop” out again. These small-current behaviors are subject to a nearly-universal behavior which I would not expect superconductivity to mess with, called quantized conductance.
That is, I would expect that even though the superconductor itself transmits charge without loss and the conductors on either side might have a resistance that's a fraction of an Ohm, I would expect that for small currents the perfect NSN junction would behave as if it had a resistance of a 12.9 kΩ, stairstepping down to 3.2 kΩ or so as you put more current through it. The idea would be that spin-0 Cooper pairs allow one ballistic transport channel at first, then maybe spin-1 Cooper pairs open up three more or so, and each one allows the transport of one conductance quantum of current per unit voltage.
So in that case, no magnetic flux is being trapped and things should look way more conventional: there is a resistance which governs the system, it's just coming from the properties of junction hopping rather than the superconducting bulk.
One interesting idea might be, “But those two mechanisms seem very different, but the two physical situations seem like they could be made arbitrarily similar. Just make the normal conductors smaller and smaller, surely you trap the magnetic field more and more?” This is interesting but potentially very risky. I would suspect that if you were able to get this to the point where you could get a cool test, you would find some really interesting results and then present it internally to your research group where your resident grumpy Russian professor (or whoever it is) would say “big deal, you found clumsy new way to measure Hall effect” and you would say “that's not the Hall effect” and then the professor would systematically dismantle months of work in about 15 minutes and you'd initially just feel misunderstood but at about 4am you'd awake with a cold sweat, “crap, it IS the Hall effect, what am I even doing with my life.” Just seems like it'd be one of those problems where fixating on “let's watch the magnetic flux seep through the cracks like air out of a balloon nozzle!” might be the wrong way to look at it.
In physics, when you say that a quantity is zero, it commonly means that its value is just very small compared to another.
In your first example, the first branch has a very small resistance compared to the second one, so it provides a much more favorable path for current.
In your second example, you have nothing to compare the resistance value with. So two things are possible:
If the hypothesis is that the resistance is too low to make a significant effect, then one would look to other impedance characteristics. The current would be shared according to the inductance of the paths, possibly including the mutual inductance coupling to any other ambient objects.
And, because the paths are connected in parallel, both currents (assuming superconductivity) would persist after the initial buildup from the ON switch event.
It really depends on how the 0 resistance is in.If it is a superconductor and lets say there isnt a limit on the superconducting current then yes almost infinite current would flow.However if the 0 resistance branch is formed by 1 positive resistance material and 1 negative resistance material then current wont flow in that branch of the circuit.
