Why isn't energy conserved for this particle?

Question

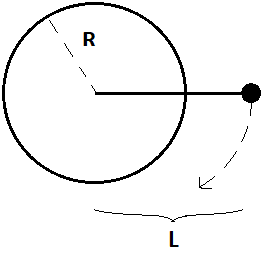
The picture below shows a particle $A$ linked to a disk through a massless rod. The rod can freely turn, with no friction, around a fixed axis that goes through the center of the disk. The system is initially at rest and starts moving because of the torque caused by the rod-particle. The moment of inertia of the disk is $0.5 \, M \, R^2$; the mass of the particle is $m$; the length of the rod is $L$.

I want to compute the speed $v_A$ when the rod is at vertical position.

I know this problem is pretty standard, and it is solved using conservation of energy of the whole system. Let me skip the details: $m g L = 0.5 \, (0.5 \, M \, R^2 + m L^2) \, \omega^2 \implies \omega = \sqrt{\frac{2mgL}{0.5 \, M \, R^2 + m L^2}}$ and therefore $v_A = \omega L = \sqrt{\frac{2mgL^3}{0.5 \, M \, R^2 + m L^2}}$

On the other hand, I feel that the energy of $A$ should also be conserved on its own, because the only non-conservative force acting on it, is perpendicular to the particle's trajectory. In this case, again skipping the details this yields $m g L = 0.5 \, m \, v_A^2$ so $v_A=\sqrt{2gL}$.

Clearly these answers are related, the first one becoming the second one as $R \rightarrow 0$. Based on what I have seen, I would take the first answer being the right one. In other words, the energy of the particle alone is not conserved, but why? What non-conservative force acting on $A$ is doing work here?

Answer 1

the only non-conservative force acting on it, is perpendicular to the particle's trajectory

This is where your reasoning is incorrect. Since the particle exerts a torque on the disc, the disc must exert an equal and opposite torque on the particle. Therefore there must be a force exerted by the rod on the particle that is not along the line joining the centre of the disc and the particle. In practice the rod will bend slightly so that the tension force it exerts on the particle is not quite perpendicular to the particle's path.

You can also see that this must be true by replacing the rod by a string. The string cannot exert a sideways force on the particle, and equally it cannot exert a torque on the disc. The disc does not rotate, the particle is not slowed down, and the particle's velocity at the bottom of its arc is now $\sqrt{2gL}$.