When to apply pulse to coil for maximum acceleration

Question

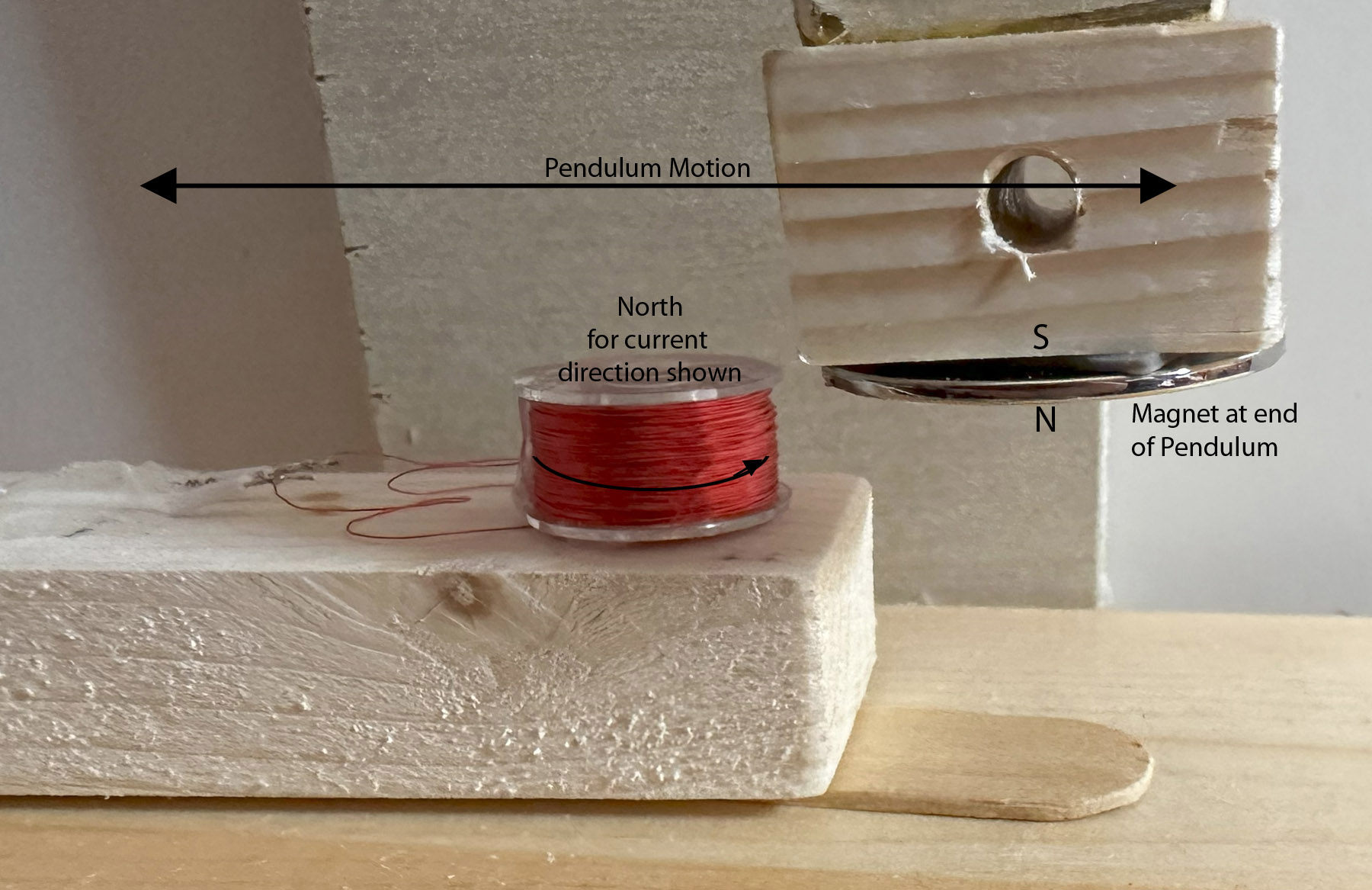
Inspired by this question, I am attempting to improve a clock pendulum (goal is for 2 AA batteries to last one year). A circuit detects the magnet departing from the coil and pulses the coil to repel the magnet. Right now, I have a fairly long pulse of current going to the coil. If I am able to reduce the friction in the pendulum pivot, I will only need a smaller pulse. Creating a narrower pulse is easier and electrically more efficient than creating a pulse with less amplitude.

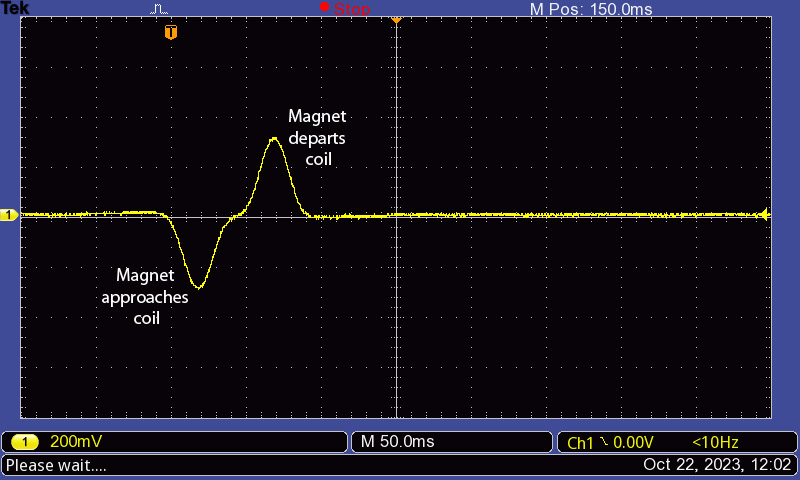
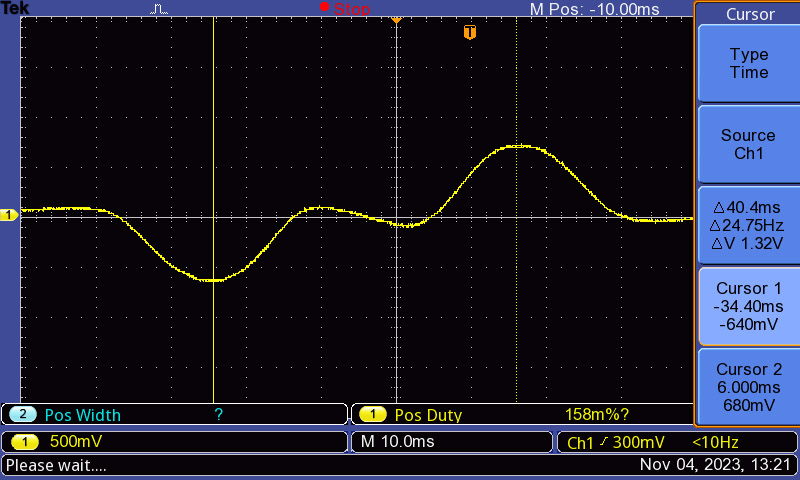
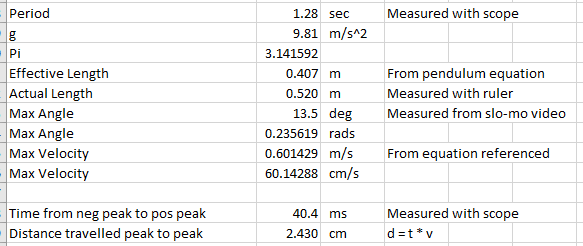
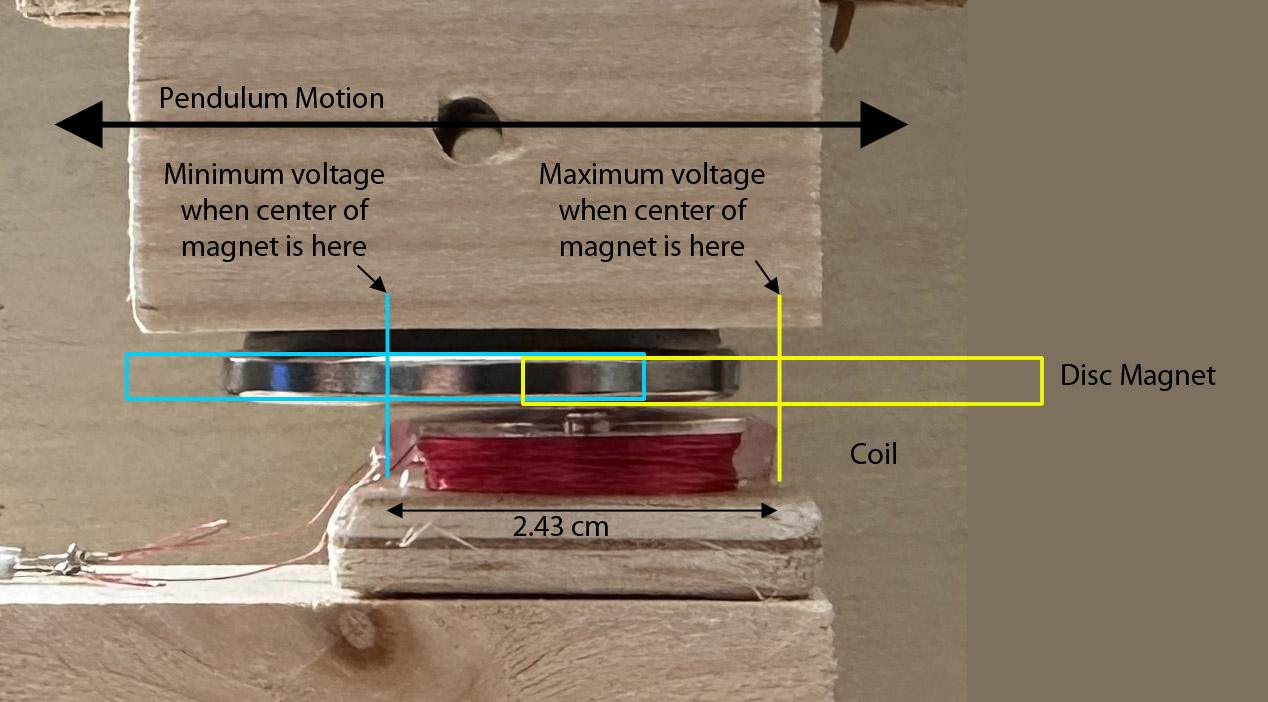
Below is a plot of the voltage from the coil as the magnet passes by (coil pulse circuit disabled). The pendulum is fairly long, so we can assume that the velocity is constant in the vicinity of the coil and the path is straight (arc is minimum).

When should the current pulse be applied to the coil to impart the maximum acceleration? I would guess that a narrow pulse coincident with the positive peak would be most efficient. I have an EE degree, so I had some college physics courses, but that was a long time ago.

Note that the pendulum is for show only, it is not used for timekeeping.

The magnet is a round flat rare-earth magnet slightly larger than the coil diameter.

Answer 1

Via experiments, I determined that the best time to apply the pulse is when the voltage is at the maximum. The experiment is more difficult than expected because the variables interact. When the delay to the pulse is changed, the pendulum amplitude changes which changes the velocity, which changes the locations of the peaks.

If the pulse is earlier, then there is only a slight decrease in pendulum amplitude. So, for simplicity, it might be best to apply the pulse as soon as a positive voltage is detected. Then, if the pendulum has a large swing, the pulse will still be in a reasonable position.

Here are some equations that allowed me to correlate a physical location to the coil voltage waveform. Maybe this will help someone explain this in Physics terms.

Pendulum equation:

$ T_0 $ is the pendulum period. $ l_e $ is the effective length of the pendulum, where the mass would be if it was concentrated in one place. $ l_a $ is the actual length, used when we calculate the velocity at the end of the pendulum (where the magnet is).

$ T_0 = 2 \pi \sqrt{\frac{l_e}{g}} $

or

$ l_e = \frac{g}{\pi^2} \frac{T_0^2}{4} $

Velocity vs time:

$ v = - \theta_0 \omega_p sin(\omega_p t) $

Where:

$ \theta_0 $ is the maximum angle from vertical.

$ \omega_p $ is the pendulum frequency in rads/sec.

$ \omega_p = \sqrt{\frac{g}{l_e}} $

The velocity near the coil is close to the maximum (>99% according to a quick calculation).

Coil voltage vs time (without driving pulse):

Calculations: