Invariance to Symmetry Operations in Taylor Expansion of Free Energy in a Nonuniform System

Question

In Cahn & Hilliards (CH) paper on the Free Energy of Nonuniform Systems (https://doi.org/10.1063/1.1744102) they present an approximation of the free (Helmholtz) energy density, $f$, in nonuniform regions. $f$ is approximated by a function of density and it's spatial derivatives ($\rho, \nabla \rho, \nabla^2\rho,...)$. For simplification, I focus on one spatial coordinate $z$ and omit terms higher than second derivative. With $\rho' \equiv \frac{\partial \rho}{\partial z}$, the free energy can then be written as a Taylor expansion about $f_0(\rho)$, which is the free energy density at $\rho$ if the system were to be uniform:

\begin{align} f(\rho, \rho', \rho'') &= f_0(\rho) + \left(\frac{\partial f}{\partial \rho'}\right)_0 d \rho' + \left(\frac{\partial f}{\partial \rho''}\right)_0 d \rho'' \\ &+ \frac{1}{2} \left(\frac{\partial^2 f}{\partial \rho'^2}\right)_0 d \rho'^2 + \left(\frac{1}{2} \frac{\partial^2 f}{\partial \rho''^2}\right)_0 d \rho''^2 + ... \end{align}

Now, CH state, for example, that $\left(\frac{\partial f}{\partial \rho'}\right)_0=0$,

since for a cubic crystal or an isotropic medium the free energy must be invariant to the symmetry operations of reflection $(z \to - z)$

I don't understand these symmetry arguments made by CH. I'm an engineer, familiar with thermodynamics, but no physicist. Can someone explain this in more simple terms or maybe give a source?

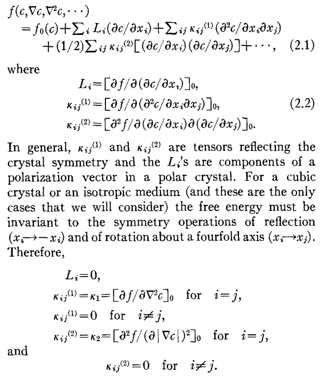
Here is a screenshot of the section in the original paper without my above stated simplifications. Here, instead of $\rho$, CH use $c$, the concentration. But since both, $\rho$ and $c$, are intensive variables the approximation is valid for both which is also stated in the original source. $x_i$ are spatial coordinates.

Answer 1

The Physical meaning of the condition of the symmetry argument arises from the isotropic medium or a cristal in one dimension: There is no invariance in the physics or the studying medium if you see it from a different perspective. Take as example the NaCl crystal. if you look it from a reference frame, you have one configuration. If you apply a 180° Rotation (or transform $z \to -z$), you obtain the same configuration, and thus, the same physics going on. That means the symmetry under the transformation $z \to - z$.

The mathematical consecuence of this argument is the following: Since the physics from the problem does not change under the transformation $z \to -z$, then certain properties of the system does not change under the same transformation. In particular, the free energy density does not change under the transformation, and it can be state that $f(z) = f(-z)$. This means that $f(z)$ is an even fuction, and because is an even function, the only coefficients in the series expantion that contributes are the even powers. That's why all the odd powers vanished, and in particular, $\frac{\partial f}{\partial \rho '} (0) = 0$.