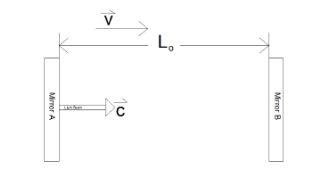
I refer here to the case of a light clock where each light pulse emitted travels forth and then reflected back between the two plates of the clock on the same axis as the direction of the moving clock:
image credits: https://www.ajuronline.org/uploads/Volume%209/Issue%202%263/92.3D-FixelleArt.pdf
Assuming you are using very short duration light pulses much shorter in duration than the time it takes them to travel to the opposite plate in the case where the clock was stationary, then when the clock is on the move and you register only the pulse moving in the same direction with the clock when it reaches the opposite plate, the result will be time dilation for the time recorded by this clock.
However my question is, if you register the time instead, only by using the reflected light pulse coming back to the initial plate, then will there be any time dilation effect?