I have three components, $A,B,C$, with 3 exchange parameters: $\chi _{AB}, \chi _{BC}, \chi _{AC}$. I want to create a FH ternary diagram to see how such a mixture behaves and how phase separation takes place.
The ternary spinodal condition is: $$D=\frac{\partial ^2 f}{\partial \phi _B ^2}\Bigg|_{N,T,\phi_A}\frac{\partial ^2 f}{\partial \phi _A ^2}\Bigg|_{N,T,\phi _B} - \left( \frac{\partial ^2 f}{\partial \phi _B \partial \phi _A} \Bigg| _{N,T}\right)^2 = 0$$ where $$f = \phi _A/v_A\ln \phi _A + \phi _B/v_B\ln \phi _B + \phi _C/v_C\ln \phi _C + \chi _{AB} \phi _A \phi _B + \chi _{BC} \phi _B \phi _C + \chi _{AC} \phi _A \phi _C$$
And the critical points can be found with a more detailed analysis.
My question is, how do I find the binodal curves of such a plot, in general? Consider a specific case of $\chi _{AB} = \chi_{BC} = \chi _{AC} = 2.4$ and $v_a = v_c = 1, v_b =5$. The ternary plot looks like follows:
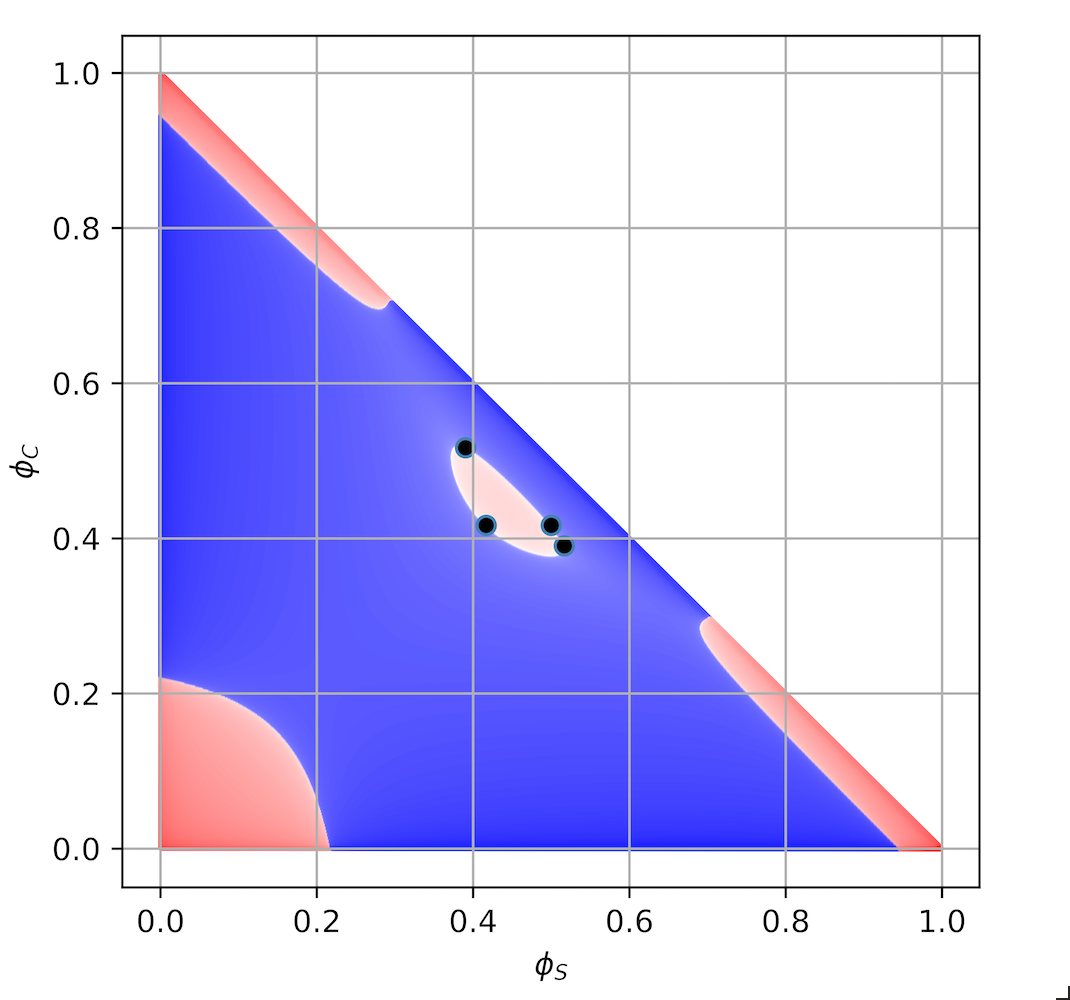
Where the critical points have been plotted -- they lie on the inner region of the spinodal. The red portion is stable, or, $D>0$ while the blue portion is unstable, or $D<0$. This paper has some insights into the exact equations that need to be solved: https://pubs.acs.org/doi/pdf/10.1021/ma00128a005. My question is, how can I evaluate the binodal of such a diagram along with the tielines? The binodal equations are a set of equations with 6 free parameters and 5 constraints, so finding a purely analytical solution is hard. But what is the process I can follow to get a numerical solution? Any advice you have would be appreciated.
