Lenz's Law is a result of the conservation of energy, and the way I think about it is that there are only two directions in which the current can flow in the loop, clockwise and counterclockwise. The resultant magnetic field caused by the loop would either attract the bar magnet or repel it.
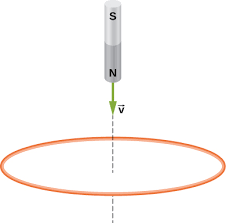
If the magnetic field by the loop was to attract the bar magnet, a small movement in the magnet would cause the loop to attract it, which would make it move faster and increase the current in the loop, which increases the attraction and so on. This creates energy out of nowhere as not only does the loop have an increasing current flowing through it, the magnet now has an increasing kinetic energy.
So it is apparent by contradiction that the resultant emf must repel the bar magnet. This also makes intuitive sense as the kinetic energy lost by the magnet now manifests as the increase in kinetic energy of the charges in the wire. So the repulsion between the magnet and the coil is not due to the power dissipated in the wire, the power that would be dissipated and the repulsion between the coil and magnet are both a result of the induced emf in the coil. This holds true for a wire of 0 resistance as well.
As a side note, a current can flow in a loop of 0 resistance with no work required, as there is no resistance to oppose the flow. In a superconducting loop, any change in flux linkage would instantly produce an opposing magnetic field, such that the flux through the loop is always 0. Reference is this thread on induced currents in a superconducting loop.

