Hofstadter butterfly patterns in different honeycomb lattice structures

Question

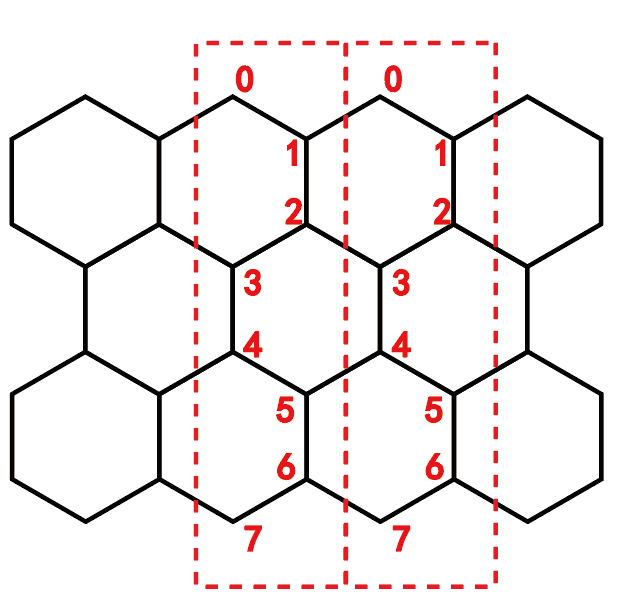
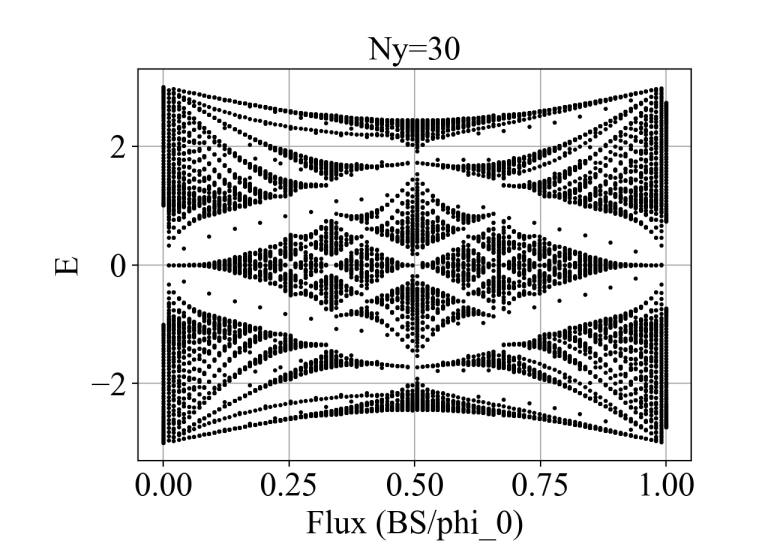
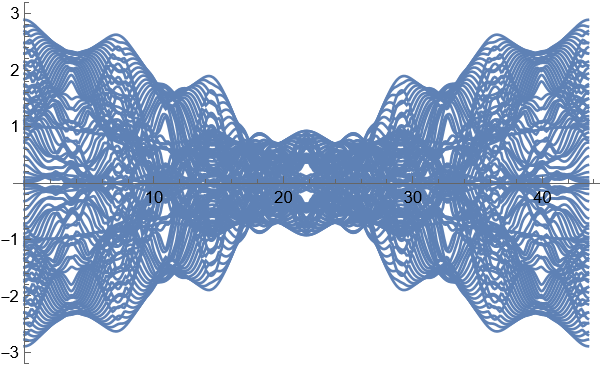
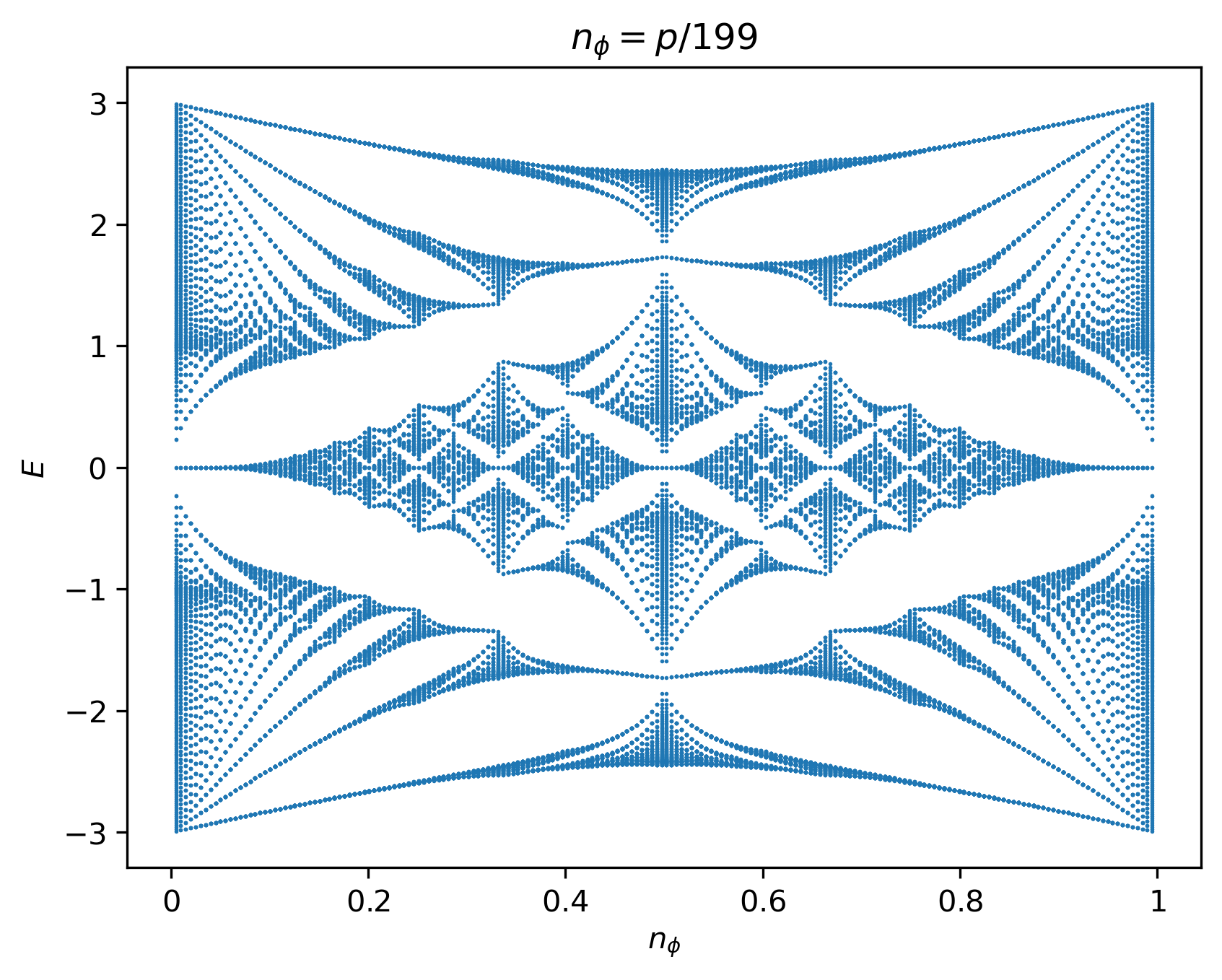
Without loss of generality, we set vector potential $A=\left( -yB,0,0 \right)$. Here B is magnetic field. And due to Peierls substitution, $t_{ij}\rightarrow \exp \left( igaA_{r_j} \right) t_{ij}$, where g is coupling strength. However, in different honeycomb lattice structures, there are different Hofstadter butterfly patterns.

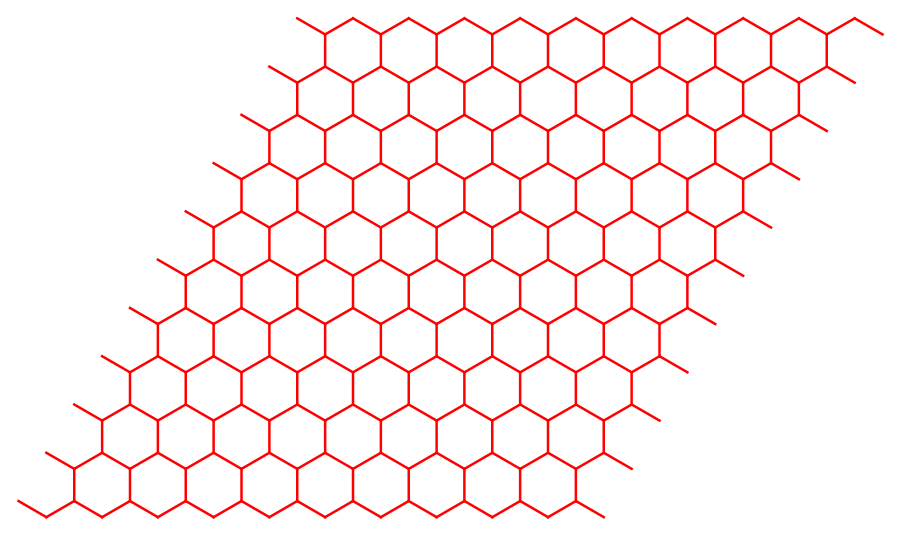
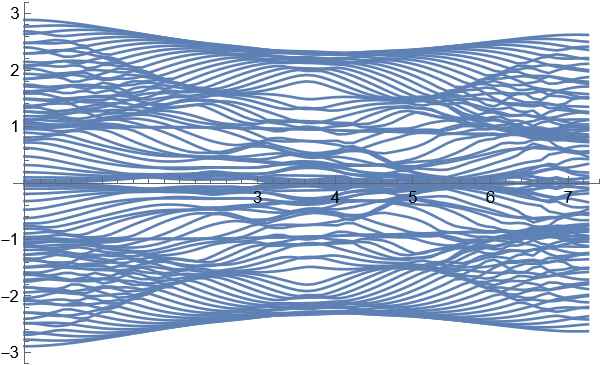
Then if we change another type of honeycomb lattice, we have:

In these last two plots, we can see that beside the period $\frac{2\pi}{\frac{\sqrt{3}a}{2}}$, there is another large $\frac{12\pi}{\frac{\sqrt{3}a}{2}}$ period, which lead energy widths from large to small and large again. Nevertheless, this property does not exist in the first type of lattice structure. I hope to understand the reason on this exotic pattern.

Answer 1

Your Hofstadter butterfly for nearest-neighbor hopping on the honeycomb lattice (first figure) is almost correct, however some aperiodicity remains in the spectrum. A common cause is using artificial Cartesian coordinates to construct the Harper equation, e.g. $(x,y) = (mb, nc)$, where $b=a/2$, $c=\sqrt{3}a/6$, and $m,n\in\mathbb{Z}$. There are several ways that you can restore periodicity in the spectrum:

1. The simplest method is to multiply all of your flux densities by two (or some larger integer). This will yield two (or more) Hofstadter butterflies in the domain $0\leq n_\phi\leq1$ with the correct periodicity.

2. An incorrect method, but one that yields the correct visual result, is to use the approach of e.g. Oh in the paper "Energy Spectrum of a Honeycomb Lattice under Nonuniform Magnetic Fields" or "Energy Spectrum of a Triangular Lattice in a Uniform Magnetic Field: Effect of Next-Nearest-Neighbor Hopping". In this case, you define the Bloch periodicity over $M = q$ $(2q)$ for even (odd) $p$, which yields exponential factors in the boundary terms of the Hamiltonian. This is incorrect because there are a different number of bands for even (odd) $p$, however, in the large $q$ limit the touching bands are indistinguishable and so the butterfly looks correct.

3. A correct method is to perform an explicit unitary transformation of the wavefunctions in the Schrödinger equation. This is sometimes known as a Rammal transformation and is given in Eq.4.7 of "Landau level spectrum of Bloch electrons in a honeycomb lattice".

4. Finally, another correct method, and the one which I recommend because it is the most intuitive, is to write down simultaneous Schrödinger equations for the two basis sites, e.g. $$E \Psi_{m,n}^A = -t e^{\mathrm{i}\theta_1}\Psi_{m,n}^B -te^{\mathrm{i}\theta_2}\Psi_{m-1,n}^B - t e^{\mathrm{i}\theta_3}\Psi_{m,n-1}^B,$$ $$E \Psi_{m,n}^B = -t e^{\mathrm{i}\theta_4}\Psi_{m+1,n}^A -te^{\mathrm{i}\theta_5}\Psi_{m,n+1}^A - t e^{\mathrm{i}\theta_6}\Psi_{m,n}^A,$$ which you can write in $2\times 2$ matrix form, $$\begin{pmatrix} H^{AA} & H^{AB} \\ H^{BA} & H^{BB} \end{pmatrix} \begin{pmatrix} \Psi^A \\ \Psi^B \end{pmatrix} = E \begin{pmatrix} \Psi^A \\ \Psi^B \end{pmatrix},$$ and then construct the $q\times q$ Harper equations for each block of this matrix with respect to the unit cells, which are fundamental quantities of the lattice. This will yield a $2q \times 2q$ matrix in total, which does not have periodicity issues in this case.

The Hofstadter butterfly for nearest-neighbor hopping on the honeycomb lattice, as well as for any combination of hoppings on any lattice, can be easily computed using the open-source Python package HofstadterTools (https://hofstadter.tools). In the package, you can run the following command in the code directory to benchmark your results:

python butterfly.py -lat honeycomb

Fixing the periodicity issue for the general case is also discussed in the HofstadterTools documentation. Once you have fixed this periodicity, you should find that there is only one correct Hofstadter butterfly for nearest-neighbor hopping on the honeycomb lattice.