Finding force vectors

Question

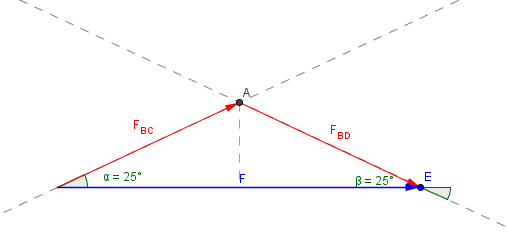
A tugboat tows a ship at a constant velocity. The tow harness consists of a single tow cable attached to the tugboat at point $A$ that splits at point $B$ and attaches to the ship at points $C$ and $D$. The two rope segments $BC$ and $BD$ angle away from the center of the ship at angles of $\varphi = 25.0^\circ$ and $\theta = 25.0^\circ$, respectively. The tugboat pulls with a force of $21,000\text{ N}$ . What are the tensions $T_{BC}$ and $T_{BD}$ in the rope segments $BC$ and $BD$?

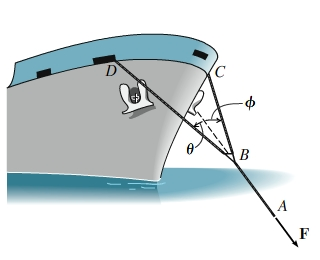
I attempted to do this by finding $BD$ and $BC$ by

$$ BD = 2100/\cos 25^\circ = 2317.1\text{ N} $$

But this is not right and I thought it was a bit too easy. Does anyone know anyway I would solve this?

Answer 1

Here is how to solve these problems in general. Make a sketch for the balance of forces.

and using trigonometry write down the $x$ and $y$ components of the vectors

$$F_{BC}\cos(\varphi)+F_{BD}\cos(\theta) = F \\ F_{BC}\sin(\varphi)-F_{BD}\sin(\theta) = 0 $$

Now solve for $F_{BD}$ and $F_{BC}$.

Answer 2

First: you use $2100 \text{ N}$ for the total force, while the question states $21,000\text{ N}$ (otherwise it would be a pretty puny tugboat...)

Second: there are 2 ropes, not 1.

Other than that: you should be fine :)