If I understand your difficulty correctly, you are assuming that since the spaceship traversed a distance of $\frac{3}{5}c \times 1$ light hours, a light signal sent from that position should arrive back to earth after exactly $\frac{3}{5}c/c = \frac{3}{5}$ hours, so your reasoning is that, including the hour prior to the transmission, a total of $\frac{8}{5}$ hours elapsed between the spaceship leaving earth and the reception of the light signal. (Which is not quite $1.5$ hours as you wrote, that's why I'm uncertain I understand your difficulty correctly).
At any rate, the problem here is not taking into account time dilation. We need to consider that clocks which are in relative motion tick at different rates, and in order to know what the clock on the ship would read once the transmitted light signal reaches earth, we have to use the Lorentz transformation for the time coordinate:
$$t'=\gamma (t-\frac{vx}{c^2})$$
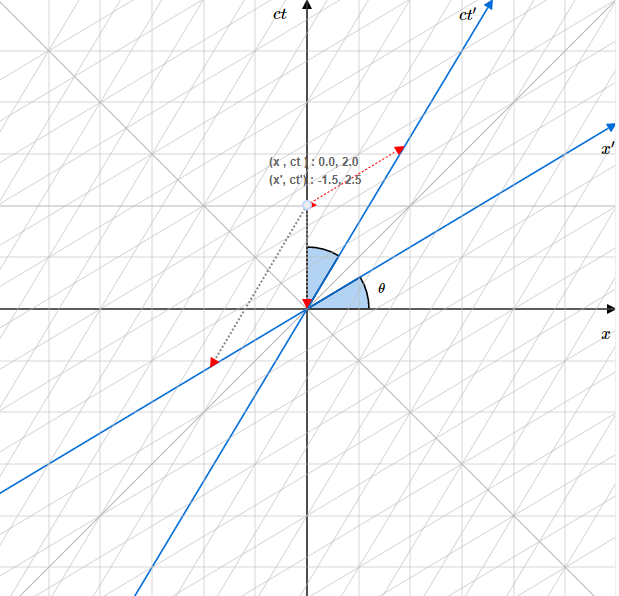
Now, the event we're interested in happens at $(t,x)=(2,0)$ which means, after $2$ hours according to the earth clock we receive a light signal at the origin (Which means $x=0$, or if you like, at zero distance from earth).
We want to find the corresponding $t'$ for that event, which is the time the clock in the spaceship's frame would register for that event.
So, substituting into the above, and putting $\gamma = \frac{5}{4}$ obtained from a previous calculation in the exercise, indeed we get (in time units of hours):
$$t'=\gamma t = \frac{5}{4} \times 2 = 2.5$$
In case you are familiar with spacetime diagrams, I've plotted using this, a diagram to show how in general, with respect to the origin of the earth's frame $x=0$, the time coordinate of the spaceship's frame is always larger than the time coordinate of the earth's frame. In particular I took this screenshot so that the surface of simultaneity of the event corresponding to the reception of the light signal is shown explicitly in the diagram (the red dashed line):
One final note on how to see why this is happening: the time between two events measured in a frame where they both happen at the same point in space is called proper time, and it is easy to show that this time is the least amount of time that can be measured between these two events, among all inertial frames, by inspecting the relation given by the invariant spacetime interval:
$$c^2 \Delta\tau^2 = c^2 \Delta{t}^2 - \Delta x^2 - \Delta y^2 - \Delta z^2$$
In the above $\Delta\tau$ is the proper time. In the general form of this relation the LHS should also have $\Delta x'$ , $\Delta y'$ and $\Delta z'$ but since by assumption both events occur at the same point in space w.r.t to the frame that measures $\Delta\tau$, they are all $0$. From this you can immediately see that $\Delta t > \Delta \tau$.
This explains why the observer on earth that sees both the events of 1. the spaceship leaving earth and 2. the light signal arriving to earth from the ship, happening at the same position in space, measures a smaller time interval than the ship does between these two events.

